Alternating Series (-1/2)^n #1 || Visual Proof

#maths #mathematics #visualmaths #proof
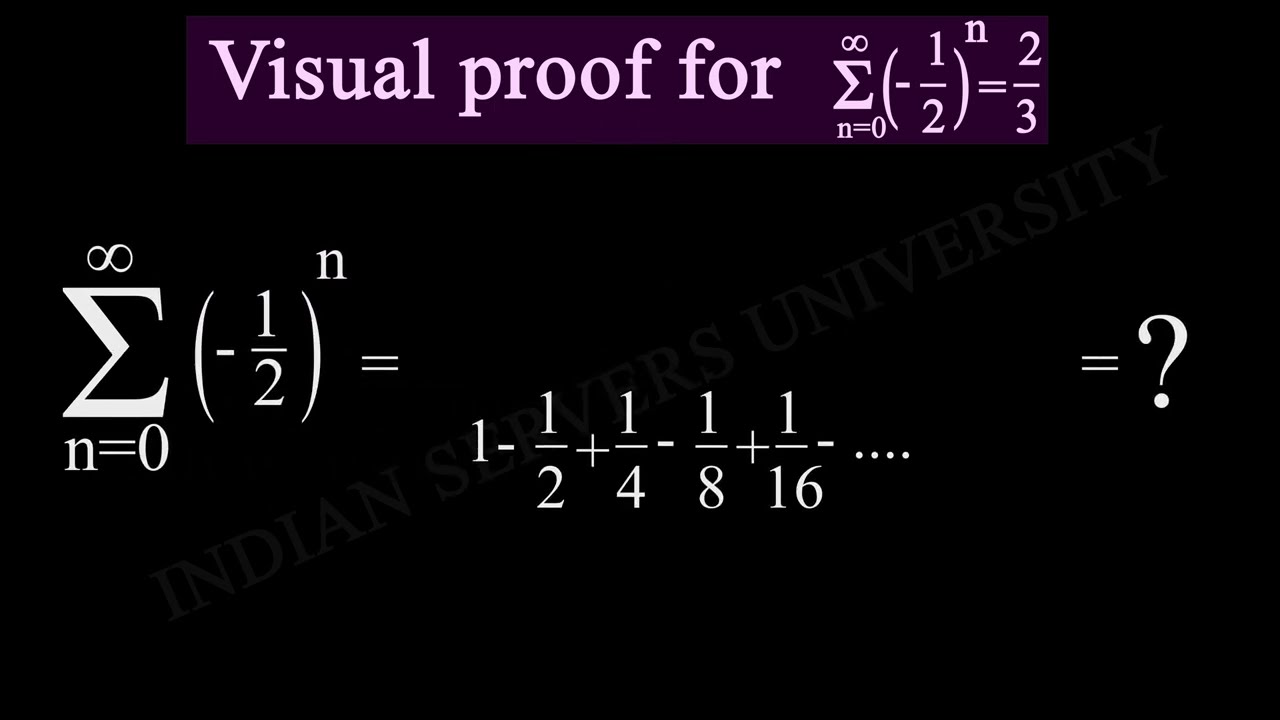
Alternating Series (-1/2)^n:
----------------------------------------------
The sum of the alternating series (-1/2)^n can be found using the formula for the sum of a finite geometric series. The formula for the sum of an alternating geometric series is given by:

S = a/(1 + r), where a is the first term and r is the common ratio

In this case, a = (-1/2)^0 = 1 and r = (-1/2), so:

S = 1 / (1 - (-1/2)) = 1 / (3/2) = 2/3

So the sum of the alternating series (-1/2)^n is equal to 2/3.

It's worth noting that while this formula provides the correct sum for some alternating series, it doesn't guarantee that the sum of a series will always converge. To determine if an alternating series converges, one can use the alternating series test, which states that if the terms of a series decrease in absolute value and approach 0, then the sum of the series converges to a finite value.

Credits:
-------------
Animator: Ravindra @ravan_thopuri
Voice-over: Viswanath Hemanth

Indian Servers University