filmov
tv
Least-squares fitting a second-order polynomial to data

Показать описание
This screen capture video is from my course "Applications of matrix computations," lecture given on April 11, 2018 at University of Helsinki, Finland.
Least-squares fitting a second-order polynomial to data.
We consider the problem of fitting a parabola to a set of measurement points. Our starting point is seven data points (real numbers) m1, m2, ..., m7, measured at times t1=1, t2=2, ..., t7=7 seconds. Here they are not really measured; we just gave some values that came to mind.
We assume an ideal quadratic model of the form m=at^2+bt+c, where a, b and c are unknown numbers to be solved.
The problem is written as a 7x3 matrix equation and solved in the sense of least squares using pseudoinverse.
Least-squares fitting a second-order polynomial to data.
We consider the problem of fitting a parabola to a set of measurement points. Our starting point is seven data points (real numbers) m1, m2, ..., m7, measured at times t1=1, t2=2, ..., t7=7 seconds. Here they are not really measured; we just gave some values that came to mind.
We assume an ideal quadratic model of the form m=at^2+bt+c, where a, b and c are unknown numbers to be solved.
The problem is written as a 7x3 matrix equation and solved in the sense of least squares using pseudoinverse.
Least-squares fitting a second-order polynomial to data
P&S-Curve Fitting-Fit a second degree polynomial to the following data by least squares-class5
Curve fitting method by the method of Least square | Curve Fitting parabola
Curve Fitting 5: Polynomial Regression
Polynomial Curve Fitting Example 2 (with Matrices)
Linear Regression Using Least Squares Method - Line of Best Fit Equation
Second order approximation-least square-curve fitting
Numerical |Polynomial Regression
@btechmathshub7050-To fit a second degree polynomial
3.9 - Curve Fitting using Least Squares Method - Fitting Data using Polynomial Fit with Python Codes
Least squares using matrices | Lecture 26 | Matrix Algebra for Engineers
P&S-CURVE FITTING Chapter-Problem on second degree polynomial by the least squares method-class6
curve fitting-least square method -second degree/Quadratic/ parabolic curve
Linear Least Squares to Solve Nonlinear Problems
Chapter 13: Polynomial Curve Fitting in MATLAB
Quadratic Regression Analysis in Excel
Fitting of a parabola/ fitting a second degree polynomial by method of least squares
Polynomial Fitting by Least Squares - Linear Algebra
Curve fitting method || least square method
The Least Squares Formula: A Derivation
Curve Fitting Straight Line & Second Degree Parabola By Least Square
Least square approximation for fitting a second order curve or parabola
Least Squares Approximation
Second Degree Parabola Straight Line y=x^2+bx+c | Example Problem 1 | Curve Fitting
Комментарии
 0:05:52
0:05:52
 0:18:34
0:18:34
 0:09:19
0:09:19
 0:17:48
0:17:48
 0:08:39
0:08:39
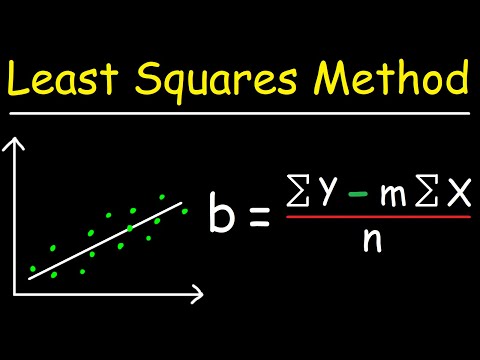 0:15:05
0:15:05
 0:02:56
0:02:56
 0:14:39
0:14:39
 0:14:37
0:14:37
 0:07:46
0:07:46
 0:10:15
0:10:15
 0:11:26
0:11:26
 0:06:27
0:06:27
 0:12:27
0:12:27
 0:08:49
0:08:49
 0:04:51
0:04:51
 0:19:19
0:19:19
 0:11:18
0:11:18
 0:11:46
0:11:46
 0:10:31
0:10:31
 0:14:59
0:14:59
 0:10:19
0:10:19
 0:07:52
0:07:52
 0:14:09
0:14:09