filmov
tv
Factorizing complex polynomials
Показать описание
Hi again everyone. Chris Tisdell here again. In this presentation I am going to continue my
series of videos on complex numbers. In the previous presentation we talked about factorizing
complex polynomials and in this video I am going to do another example illustrating how to use
these kinds of ideas. Let me share my screen with you and we can get underway. This is what
we looked at last time. I did not prove any of these things but the ideas were on the application.
Suppose I have got a complex polynomial , the coefficients could be real, could be complex.
These 4 points are very important, very deep results. 00:00-00:48.
Every polynomial of degree n has at least one root in complex numbers. That is there is one
number α such that p(α ) is equal to 0. If the coefficients a terms are real then the roots of
the complex polynomial appear in conjugate pairs. If α is a root then z – α is a factor of the
polynomial. That is like the factor theorem. And every polynomial of degree n can be factored
into n linear parts. You can write it like this where αs are the roots and the a_n is the coefficient
of the z^n. 00:48-01:30.
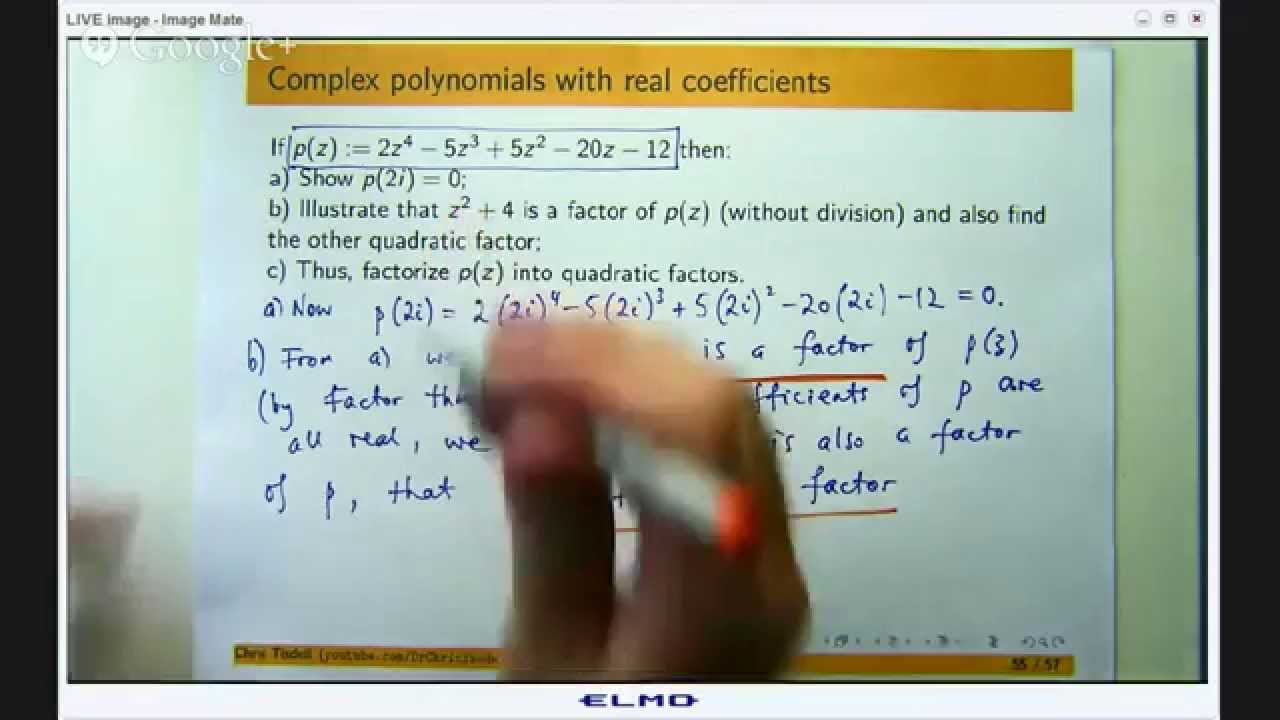
Let's talk about an example now. Here we have defined a polynomial of degree 4. Show that 2i is a
root of the polynomial. Illustrate that z^2 + 4 is factor without division and also find the other
quadratic factor. Thus factorize p(z) into quadratic factors. We are not talking about linear
factors there just quadratic factors. So, polynomials of degree 2. Part a is the easiest part. Let
us just box our polynomial. All you need to do is put 2i into the polynomial for z . I will not spend
much time on that. The important thing to remember is i^2 = -1. Pretty much everything flows
from there. If you expand these out you will get 0. Now what do we know then We want to
illustrate the z^2 – 4 is factor without division. Part a tells us z - 2i is a factor of p(z) by the factor
theorem. And all the coefficients of the z’s and z^2 etc are real What does that mean ? That
means that the conjugate of 2i is also a root. Coefficients of p are all real, we have z – the
conjugate is also a factor of p. what is the conjugate of 2i ? It's -2i. So it will be z - - 2i. so we have got z - 2i is factor z + 2i is a factor. What we are trying to show
is that z^2 + 4 is factor. The product of these two things will be factor. And it turns out that the
product of these two things will be exactly that. So if I work this out by expanding I will have z^2 + 4 again remembering that i^2 = -1. So that is part b. 01:30-05:32.
Also find the other quadratic factor. Well now we were not allowed to use division up here but
we are allowed to use it now. Let us divide in and see if we can simplify here. So this is part b
continued. So I am going to divide z^2 + 4 which z^2 + 0 * z + 4 into
my polynomial. Now sometimes there are faster techniques but this is the way I am just going to
do it now. That into that goes 2 z squared times.. So that times that, that times that, that times
that gives you that. Take this away from that and you will get the following. Bring that one down.
Okay now divide this into this. You will get -5z. Now that times that that times that gives
you the line down here. This taken away from this gives you the following. Bring -12
down and finally this divides into that -3 times and then this time p, that will give you exactly this.
This gives you identical row yielding 0. 05:32-07:56.
There is one quadratic factor and there is another quadratic factor. So this is part c now. Okay
so there you go. You can go a little bit further. In here the polynomial can be factorized into
linear parts. A sub n is the coefficient of z to the n and the a I’s are roots of p. I want to leave
you with something to do. This is part d. this is for you. Show that p of z has its linear factored
form like this. This is z -3. This is a little exercise for you to do. You know that this is going to go
into that and it is really just a matter of splitting this up into this by finding the zeros or using
some other method. 07:56-09:47.
Okay that is my presentation. Hope you enjoyed it. Hope you found it useful. If you have any
suggestions or any feedback leave me a message or post a comment. Hope you are enjoying
these videos and please join me in the next few videos on complex numbers. Hope to see you
then. 09:47-10:18.
series of videos on complex numbers. In the previous presentation we talked about factorizing
complex polynomials and in this video I am going to do another example illustrating how to use
these kinds of ideas. Let me share my screen with you and we can get underway. This is what
we looked at last time. I did not prove any of these things but the ideas were on the application.
Suppose I have got a complex polynomial , the coefficients could be real, could be complex.
These 4 points are very important, very deep results. 00:00-00:48.
Every polynomial of degree n has at least one root in complex numbers. That is there is one
number α such that p(α ) is equal to 0. If the coefficients a terms are real then the roots of
the complex polynomial appear in conjugate pairs. If α is a root then z – α is a factor of the
polynomial. That is like the factor theorem. And every polynomial of degree n can be factored
into n linear parts. You can write it like this where αs are the roots and the a_n is the coefficient
of the z^n. 00:48-01:30.
Let's talk about an example now. Here we have defined a polynomial of degree 4. Show that 2i is a
root of the polynomial. Illustrate that z^2 + 4 is factor without division and also find the other
quadratic factor. Thus factorize p(z) into quadratic factors. We are not talking about linear
factors there just quadratic factors. So, polynomials of degree 2. Part a is the easiest part. Let
us just box our polynomial. All you need to do is put 2i into the polynomial for z . I will not spend
much time on that. The important thing to remember is i^2 = -1. Pretty much everything flows
from there. If you expand these out you will get 0. Now what do we know then We want to
illustrate the z^2 – 4 is factor without division. Part a tells us z - 2i is a factor of p(z) by the factor
theorem. And all the coefficients of the z’s and z^2 etc are real What does that mean ? That
means that the conjugate of 2i is also a root. Coefficients of p are all real, we have z – the
conjugate is also a factor of p. what is the conjugate of 2i ? It's -2i. So it will be z - - 2i. so we have got z - 2i is factor z + 2i is a factor. What we are trying to show
is that z^2 + 4 is factor. The product of these two things will be factor. And it turns out that the
product of these two things will be exactly that. So if I work this out by expanding I will have z^2 + 4 again remembering that i^2 = -1. So that is part b. 01:30-05:32.
Also find the other quadratic factor. Well now we were not allowed to use division up here but
we are allowed to use it now. Let us divide in and see if we can simplify here. So this is part b
continued. So I am going to divide z^2 + 4 which z^2 + 0 * z + 4 into
my polynomial. Now sometimes there are faster techniques but this is the way I am just going to
do it now. That into that goes 2 z squared times.. So that times that, that times that, that times
that gives you that. Take this away from that and you will get the following. Bring that one down.
Okay now divide this into this. You will get -5z. Now that times that that times that gives
you the line down here. This taken away from this gives you the following. Bring -12
down and finally this divides into that -3 times and then this time p, that will give you exactly this.
This gives you identical row yielding 0. 05:32-07:56.
There is one quadratic factor and there is another quadratic factor. So this is part c now. Okay
so there you go. You can go a little bit further. In here the polynomial can be factorized into
linear parts. A sub n is the coefficient of z to the n and the a I’s are roots of p. I want to leave
you with something to do. This is part d. this is for you. Show that p of z has its linear factored
form like this. This is z -3. This is a little exercise for you to do. You know that this is going to go
into that and it is really just a matter of splitting this up into this by finding the zeros or using
some other method. 07:56-09:47.
Okay that is my presentation. Hope you enjoyed it. Hope you found it useful. If you have any
suggestions or any feedback leave me a message or post a comment. Hope you are enjoying
these videos and please join me in the next few videos on complex numbers. Hope to see you
then. 09:47-10:18.
0:03:10
Factoring polynomials using complex numbers | Khan Academy
0:11:26
Polynomial Function - Complex Factorization Theorem
0:11:54
How To Factor Polynomials The Easy Way!
0:04:07
Factoring Complex Polynomials 1
0:05:33
Factoring 5-term polynomials by grouping
0:11:40
2.6 factoring complex polynomials
0:05:35
Finding All of the Zeros of a Polynomial Including Complex
0:03:14
Example: Complete Factorization of a Polynomial in the Complex Numbers (4)
0:04:55
How to factor a hard 4th degree polynomial (no rational zero, can't do it by grouping)
0:07:05
Complex numbers lesson 21 - Factorise quadratic polynomials over C
0:03:45
Solve a Polynomial with Complex Zeros by Factoring
0:10:19
Factorizing complex polynomials
0:34:46
Factoring Trinomials & Polynomials, Basic Introduction - Algebra
0:05:47
How To Factor Difficult Quadratic Equations
0:00:47
Factoring 3x³ + x² - 15x - 5 #Shorts #algebra #math #maths #mathematics #education #learn #learning...
0:07:25
Factor Polynomials with Complex Numbers
0:01:32
Example: Complete Factorization of a Polynomial in the Complex Numbers (1)
0:05:20
How to factor a 5-term 4th degree polynomial (factor by grouping)
0:18:22
Factorising polynomials with complex numbers
0:03:26
Factoring a polynomial to the fourth power using factoring to second power
0:09:22
Solving Higher-Degree Polynomials by Synthetic Division and the Rational Roots Test
0:14:19
Solving Polynomial Equations By Factoring and Using Synthetic Division
0:01:31
Example: Complete Factorization of a Polynomial in the Complex Numbers (2)
0:04:33
Factor Polynomials with Complex Numbers: Sum of Squares
Комментарии