filmov
tv
My Proof of Pythagoras' Theorem is NOT circular!

Показать описание
Pythagorean Theorem: Six Proofs
Pythagorean theorem: Euclid’s proof reimagined
Easiest Proof of Pythagoras' Theorem
Simple proof of Pythagoras' theorem for everyone
visual proof of Pythagoras theorem! #ibps #ibpspo #sbipo #upsc
Pythagoras' Theorem edit 🐐🔥 | #shorts #maths
18 year old students just discovered a proof of Pythagoras that mathematicians said was impossible
Visual Proof of Pythagoras' Theorem
Proving the Pythagorean Theorem
Pythagorean theorem proof by scaling
Pythagoras Would Be Proud: High School Students' New Proof of the Pythagorean Theorem [TRIGONOM...
What they don' t tell you about Pythagoras Theorem. Cool proof !
Visualising Pythagoras: ultimate proofs and crazy contortions
High School seniors discover possible new proof for the Pythagorean theorem
Proof of Pythagoras' Theorem
Pythagorean Theorem & Its Inverse (my favorite proof)
My Proof of Pythagoras' Theorem is NOT circular!
Pythagorean Theorem Proof (Geometry)
Pythagorean Theorem - Behold!
Pythagorean Theorem Dissection
Pythagoras' Theorem | Maths made Beautiful
Proof of Pythagorean Theorem with Similar Triangles
Pythagoras' theorem explained visually (changed my life!)
Proof of Pythagoras' theorem - GCSE Maths
Комментарии
 0:01:00
0:01:00
 0:00:48
0:00:48
 0:01:00
0:01:00
 0:05:25
0:05:25
 0:00:16
0:00:16
 0:00:19
0:00:19
 0:18:07
0:18:07
 0:11:14
0:11:14
 0:03:34
0:03:34
 0:00:54
0:00:54
 0:13:57
0:13:57
 0:06:10
0:06:10
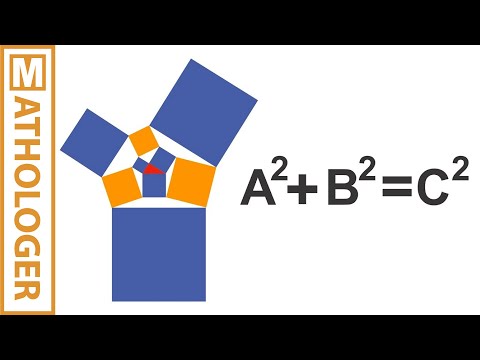 0:21:01
0:21:01
 0:04:26
0:04:26
 0:02:20
0:02:20
 0:08:12
0:08:12
 0:14:16
0:14:16
 0:04:10
0:04:10
 0:00:54
0:00:54
 0:00:36
0:00:36
 0:01:58
0:01:58
 0:03:31
0:03:31
 0:02:08
0:02:08
 0:01:51
0:01:51