filmov
tv
Algebraic Structures: Groups, Rings, and Fields
Показать описание
This video covers the definitions for some basic algebraic structures, including groups and rings. I give examples of each and discuss how to verify the properties for each type of structure.
Algebraic Structures: Groups, Rings, and Fields
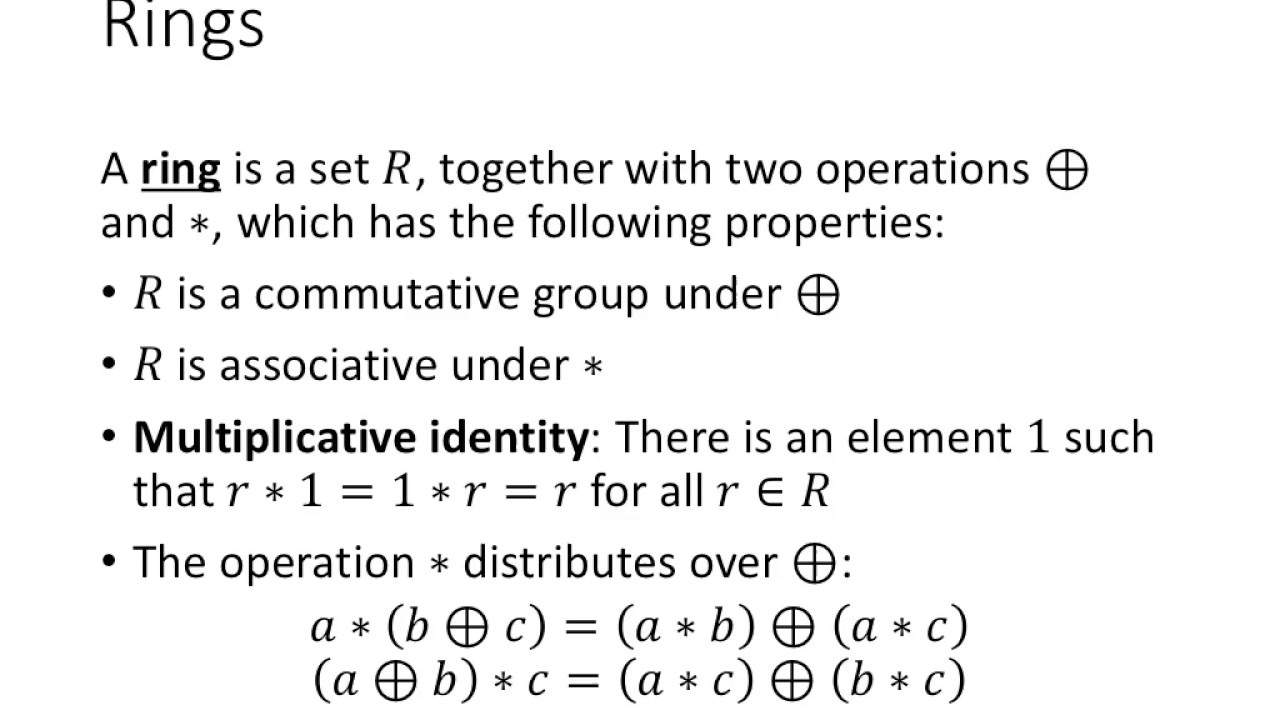
Ring Definition (expanded) - Abstract Algebra
Rings, Fields and Finite Fields
Group theory, abstraction, and the 196,883-dimensional monster
Group Definition (expanded) - Abstract Algebra
Introduction to Ring, Field and Integral Domain - Algebraic Structures - Discrete Mathematics
Group and Abelian Group
Ring Examples (Abstract Algebra)
Group Rings
Lec01 Introduction to Algebraic Structures Rings and Fields
Algebraic Structures: Groups, Semigroup, monoid, Ring and Field Axioms with Example
Recap of Groups, Rings, and Fields
Algebraic Structures by Serge Lang #shorts
Abstract Algebra | What is a ring?
COMP231 Lecture 18 Groups, Rings, Fields Part1
Algebraic structure
Introducing Algebraic Structures: Magmas and Groupoids [ N is closed under + ]
Part II - 13. Algebraic structures: (Abelian) groups, rings & fields
AES I - Group, Ring, Field and Finite Field - Abstract Algebra Basics - Cyber Security - CSE4003
Groups - Showing G is a group - Part 1
Semigroups and Abelian Algebraic Structures
01 Algebraic Structures
Group,Abelion group,Ring and field
Group & Ring #2 | Group Theory | Algebraic Structures
Комментарии
 0:23:42
0:23:42
 0:06:51
0:06:51
 0:13:04
0:13:04
 0:21:58
0:21:58
 0:11:15
0:11:15
 0:17:06
0:17:06
 0:10:44
0:10:44
 0:07:18
0:07:18
 0:15:23
0:15:23
 0:32:20
0:32:20
 0:02:27
0:02:27
 0:07:44
0:07:44
 0:00:23
0:00:23
 0:08:52
0:08:52
 0:18:30
0:18:30
 0:17:29
0:17:29
 0:06:02
0:06:02
 0:13:55
0:13:55
 0:35:45
0:35:45
 0:05:35
0:05:35
 0:10:56
0:10:56
 0:25:09
0:25:09
 0:06:57
0:06:57
 0:14:40
0:14:40