filmov
tv
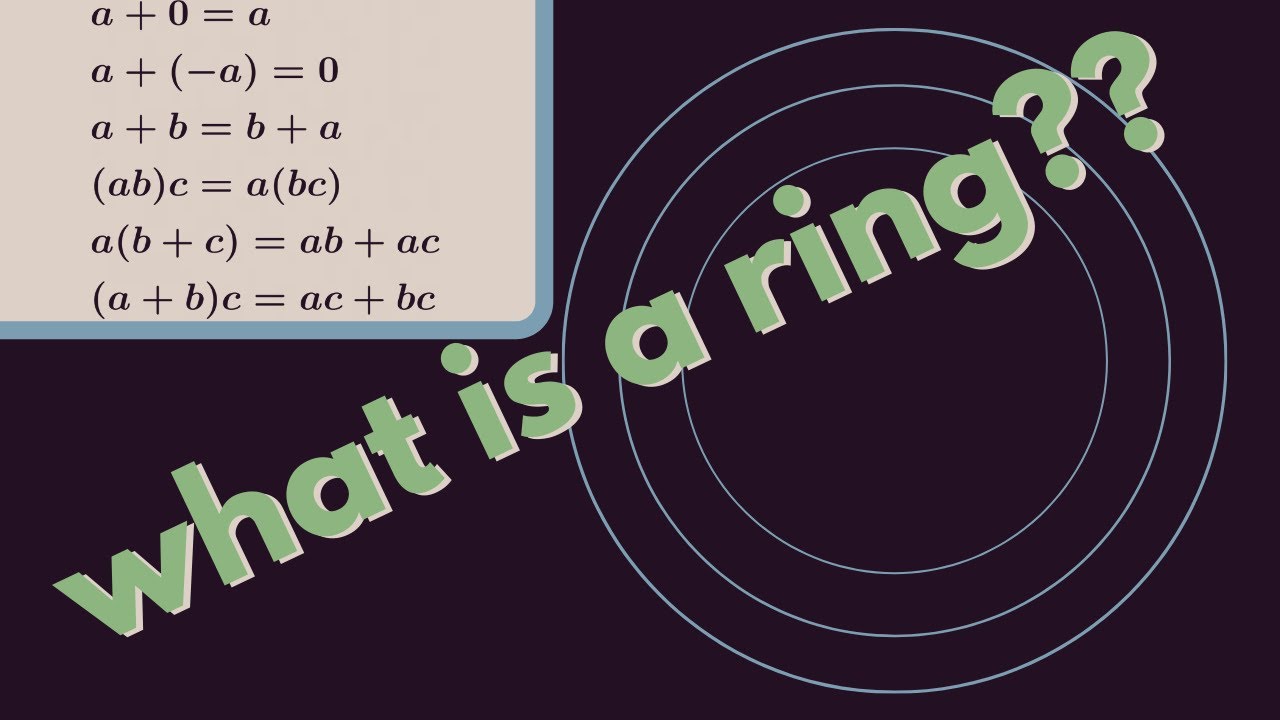
Abstract Algebra | What is a ring?
Показать описание
We give the definition of a ring and present some examples.
What is Abstract Algebra? (Modern Algebra)
Group Definition (expanded) - Abstract Algebra
Abstract Algebra: The definition of a Group
Group theory, abstraction, and the 196,883-dimensional monster
Teaching myself abstract algebra
Ring Definition (expanded) - Abstract Algebra
Exploring Abstract Algebra
(Abstract Algebra 1) Definition of a Group
SET MATHS /NUMBER THEORY/ CLASS 1#setexammathematicalscience #setmathsjanuary2024numbertheory
What is Abstract Algebra?
Field Definition (expanded) - Abstract Algebra
What is a Group? | Abstract Algebra
A Friendly Introduction to Abstract Algebra / Group theory (lesson 1): SYMMETRY GROUPS
What is a Module? (Abstract Algebra)
Abstract Algebra Book with TONS of Content
Abstract algebra and linear algebra | What is abstract and linear algebra |#shorts #youtubeshorts
Abstract Algebra | What is a ring?
Concepts in Abstract Algebra
Which is the Harder Math Class? Abstract Algebra versus Advanced Calculus
Abstract Algebra is Impossible Without These 8 Things
Abstract Algebra is Nuts
Introduction to Abstract Algebra
Abstract Algebra: The definition of a Ring
Abstract Algebra - 2.1 Definition and Examples of Groups
Комментарии
 0:03:22
0:03:22
 0:11:15
0:11:15
 0:03:11
0:03:11
 0:21:58
0:21:58
 0:14:41
0:14:41
 0:06:51
0:06:51
 0:00:25
0:00:25
 0:12:25
0:12:25
 0:05:51
0:05:51
 0:09:42
0:09:42
 0:08:06
0:08:06
 0:19:46
0:19:46
 0:27:32
0:27:32
 0:07:43
0:07:43
 0:00:34
0:00:34
 0:00:59
0:00:59
 0:08:52
0:08:52
 0:05:08
0:05:08
 0:08:09
0:08:09
 0:14:10
0:14:10
 0:21:15
0:21:15
 0:09:10
0:09:10
 0:03:13
0:03:13
 0:16:11
0:16:11