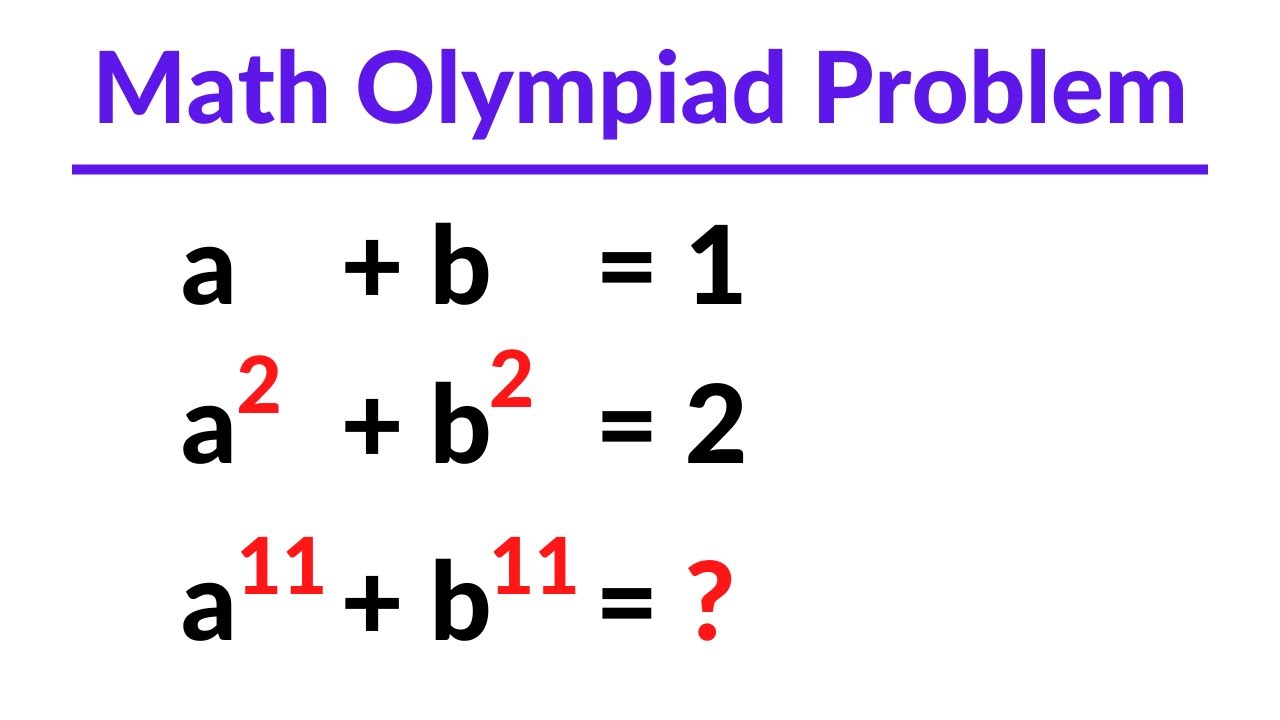
Math Olympiad Problem | A Nice Algebra Challenge

Math Olympiad Problem | A Nice Algebra Challenge

Topics Covered :-
Math Olympiad Problem
Algebra Problem
Olympiad Mathematics
System of Equations
math olympiad algebra problem
math
olympiad mathematics
math olympiad preparation
mathematics
maths
math olympiad questions
math olympics
math problems
olympiad
premath
math olympiad problems
challenging math problems
algebra
sybermath
algebra 1
math puzzles
math way
algebra 2
sat math
quadratic equations
algebraic equations
nice algebra problem
olympiad exam 2022
khan academy
pre math
imo
solve the rational equation
math olympiad question
high school math
math olympiad
how to study math
college math
algebra problem
precalculus
math tournament solutions
math olympiad problems with solutions
challenging math questions
trending math problem
olympiad trending math questions
difficult math equations
equations
mathematical olympiad
challenging math
trending mathematics
olympiad math
algebra for beginners
math problems with solutions
math olympiad solutions
math competition solutions
solve for x
difficult math problems
challenging maths questions with answers
how to solve system of equations
maths challenging questions
how to solve system of equations for x and y and z
system of equations
how to solve equations
algebra problems
find x
math olympiad 2022
mind your decision

Math Booster will provide you quality content on different topics of mathematics such as algebra, calculus etc.. that will be helpful for math olympiad or any other competitive exams. In every video, you will get a new math problem with step by step solution. So Subscribe the channel and press the bell icon to get notified of my new videos.

Join this channel to get access to perks:

Watch More Videos
----------------------------------
For Math Olympiad

For IIT-JEE

Algebra

Geometry

Trigonometry

Quadratic Equations

logarithms

Sequence And Series

Functions

Differentiation

Integration

Number Theory

Permutation And Combination

Probability

Limits

Differential Equation

Interesting Math

#mathbooster #matholympiad #mathematics #premath #sybermath #blackpenredpen #justalgebra #math #maths #olympiadmathematics #matholympics #mindyourdecisions #solveforx #mathproblems #algebra

[anson]

just substitute b for (1-a) and use quadratic equation to find (a) in the second equation, then calculate a^11 + (1-a)^11, and the answer will be 989/32

[marizacabral]

You can use the original 2 equations to solve for a and b directly. Two equations with 2 unknowns...

[lusax]

a+b=1 … set T1
a²+b²=2 … set T2 (ex. a^n+b^n=Tn)
∴ ab=-1/2
equation whose root is a, b
t²-t-1/2=0
it's identity so we can substitute t to x
x²-x-1/2=0 … ×x^n
x^(n+2)=x^(n+1)+(1/2)x^n
same method,
y^(n+2)=y^(n+1)+(1/2)x^n
add two equations

then
Tn+2=Tn+1+(1/2)Tn
∴ T3=T2+(1/2)T1
T4…
and finally, solve T11. that's the answer.

[claudiosaccon]

Let c_n:=a^n+b^n.
Then c_1=1 and c_2=2.
Moreover 1=(a+b)^2=a^2+2ab+b^2=2+2ab hence ab=-1/2.
Let's find a recursive formula for c_n :
c_n=1 × c_n=c_1 c_n=(a+b)(a^n+b^n)=
a^(n+1)+a^n b+a b^n+b^(n+1)=
c_(n+1)+ab c_(n-1)=c_(n+1)-(1/2)c_(n-1).
It follows:
c_(n+1)=c_n+(1/2)c_(n-1).

[GodbornNoven]

a+b=1
b=1-a
a²+(1-a)²=2
a²+1-2a+a²=2
2a²-2a=1
a²-a- 1/2 = 0
Solve for a by using quadratic formula, solve for b by using a. Then raise a and b individually to the 11th power and add them.
No need to overcomplicate it

[davidash]

Another way of approaching this is to let S_n=a^n+b^n for any n. Then S_0=2 (trivially) and S_1=1, S_2=2 (we are given this). a and b must both be roots of some quadratic x^2+ux+v=0. So a^2+ua+v=0 and b^2+ub+v=0, and hence for any n, a^(n+2)+ua^(n+1)+va^n=0 and b^(n+2)+ub^(n+1)+vb^n=0. Adding the recurrence S_(n+2)=-uS_(n+1)-vS_n holds for all n. u must be the negation of (a+b) so u=-1, and S_(n+2)=S_(n+1)-vS_n. Given S_2=2, S_1=1, and S_0=2, we can solve for v: v=-1/2.

The recurrence then becomes S_(n+2)=S_(n+1)+S_n/2, and we can then evaluate S_11 by repeated application of this recurrence.

[vishalmishra]

*Simpler Solution*
a + b =1 and a^2 + b^2 = 2 Therefore, 2ab = 1^2 - 2 = -1. So, ab = -1/2. Therefore, a and b = (1 ± √3) / 2.
Using Pascal's triangle, the co-efficients of (a + b)^11 = [ 1, 11, 55, 165, 330, 462, 462, 330, 165, 55, 11, 1 ]
Due to ± the odd terms will cancel out and the even terms will double. So √3 terms will cancel out and powers of 3 will double. Denominator = 2^11 = 2048.
Powers of 3 are [ 1, 3, 9, 27, 81, 243 ] and (even) binomial coefficients are [ 1, 55, 330, 462, 165, 11 ]
Hence, a^11 + b^11 = 2 x [ 1, 3, 9, 27, 81, 243 ] . [ 1, 55, 330, 462, 165, 11 ] / 2^11 = 2 x 31648 / 2048 = 989 / 32

[MeNowDealWIthIt]

My solution:

a+b=1 -> b=1-a
So a^2+b^2=2 -> a^2+(a-1)^2=2
2a^2-2a+1=2
a^2-a-.5=0
a=(1+-sqrt(3))/2
Checking the solutions for the first to equations means a=(1+sqrt(3))/2 and b=(1-sqrt(3))/2 or vice versa, it doesn't change what we're trying to solve.

Pascal's triangle starting at the part I have memorized:
1 6 15 20
1 7 21 35 35
1 8 28 56 70
1 9 36 94 126 126
1 10 45 132 220 252
1 11 55 177 352 472 472 352 177 55 11 1

a^11+b^11
=((1+sqrt(3))/2)^11 + ((1-sqrt(3))/2)^11


Pascal's triangle starting at the part I have memorized:
1 6 15 20
1 7 21 35 35
1 8 28 56 70
1 9 36 94 126 126
1 10 45 132 220 252
1 11 55 177 352 472 472 352 177 55 11 1
I know the odd degree terms will be opposites, and the even degree powers will be the same, so just doubling the even degree powers will be equivalent.





=31088/1024
=16544/512
=8272/256
=4136/128
=2068/64
=1034/32
which means I did some arithmetic wrong somewhere. damn.

[channelsixtysix]

That was an enjoyable nine minutes and fifty seconds. You had me laughing as it all resolved. Marvellous.

[jcb]

If f(n) = a^n + b^n then f(n) satisfies the recurrence f(n+1)=f(n)+ f(n-1)/2. Given f(1)=1 and f(2)=2 it is pretty quick to compute f(11)

[vincenzofonseca]

Guys, I think that if we expand (1-a)^11 using binomial theorem, we would take less time as the video's resolution took. Almost 10 minutes is a lot of time...

[atikshchawla]

Another simpler method for this question is as follows
first get the value of ab by using the formula
(a+b)^2 = a^2 +b^2 +2ab

then use the formula
(a-b)^2 = a^2 +b^2 -2ab
to get the value of a-b

then simply equate a+b and a-b together to get the value of a and b
and then simply substitute them to the required equation i.e. a^11 +b^11

[l.w.paradis]

Your approach brought back my many winter nights of solving definite integrals. :)

[owlsmath]

Good problem. Was hoping for a shortcut but I guess you just have to keep multiplying in this case

[MrLidless]

Once you get ab = -0.5, ie b = -1/2a, slot it into the first equation.
a - 1/2a = 1

Rearranging:
a^2 = a + 1/2
a^3 = a^2 + a/2 = a + 1/2 + a/2 = 3a/2 + 1/2. So a^3 is a multiple of a + constant. Multiply this by a again to get
a^4 = 2a + 3/4
Etc etc. You’ll soon see the pattern for a^n = f(n)a + g(n) and can therefore jump to a^11

Ditto with b.

You’ll get to the answer more elegantly.

[michroz]

I got 30.90625 straightforward with Desmos graphing calculator to skip calculations.
a = 1-b; b=1/2-(sqrt(3))/2, a=1/2+(sqrt(3))/2, etc.
Not sure I'd pass the Olympiad this way - they prefer tricky solutions.

[filippocontiberas]

This because there are only 2 solutions for A and two for B such that all of them are shared valid solutions for the 3 equations :
Case 1: A = [ 1 - sqrt( 3 ) ] / 2 and B = [ 1 + sqrt( 3 ) ] / 2
Case 2: A = [ 1 + sqrt( 3 ) ] / 2 and B = [ 1 - sqrt( 3 ) ] / 2
The case 1 and case 2 are two points where the line ( first linear equation ), the circle ( second quadratic equation ) and the curve ( third 11th equation ) meets all at the same time.

[rameshchandra-qgsw]

If you had a calculator then you could have solved a and b by using the property: a^2+b^2 = (a+b)^2+(a-b)^2 => a-b= sqrt (3). a=(1 + sqrt(3))/2 and b=(1 - sqrt(3))/2. a= 1.366, b= -0.366. Now substitute back in a^11 + b^11. Approx. 30.89. Off course, if no calculator your elegant method is needed

[hetanshpatel]

A faster method:

a + b = 1
a = b-1

a² + b² = 2
(b-1)² + b² = 2
b² - 2b + 1 + b² = 2
2b² - 2b + 1 = 2

with quadratic formula:
b = (1 ± √3)/2
a= (-1 ± √3)/2

We disregard negative since it gives negative output

b = (1 + √3)/2
a= (-1 + √3)/2

Now we substitute in a¹¹+b¹¹ and we get a value close to 30.60925, which is 19/4.

[erkinjonmeliboyev]

Respect, you are very clever person.
Thank you for this problem which is solved by you