Change of Variables: Homogeneous Differential Equations (Example 1)

Change of Variables: Homogeneous Differential Equations
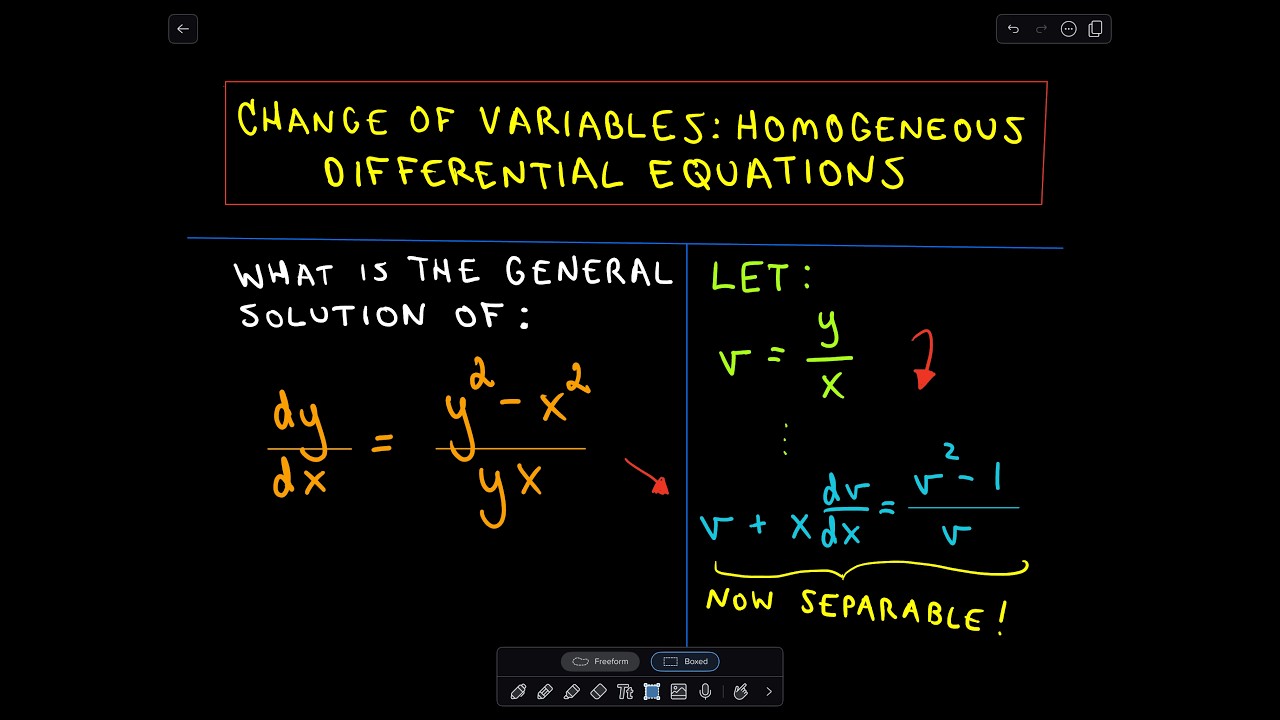
In this video, we explore how to solve homogeneous differential equations using a change of variables technique. Homogeneous differential equations often arise in various fields, including physics, engineering, and economics. We'll break down the steps to reduce the equation to a separable form and find the general solution by applying a substitution. You'll also learn how to solve the resulting separable differential equation, step by step.

What You Will Learn:

How to identify homogeneous differential equations.
The process of changing variables to simplify the equation.
How to separate variables and solve for the general solution.
Key concepts and formulas related to homogeneous equations.
This video is perfect for students studying calculus or differential equations, or for anyone looking to sharpen their math problem-solving skills!

If you find this video helpful, don't forget to like, share, and subscribe for more math tutorials. Feel free to ask questions in the comments and share this video with friends, teachers, and classmates!

Support me on Patreon:

#patrickjmt #calculus #differentialequations #mathtutorial #homogeneousdifferentialequations #changethevariables #separableequations #solvingdifferentialequations #homogeneoussubstitution #mathhelp #stepbystepmath #mathsolutions #learncalculus #engineeringmath #calculusproblems #generalcalculus #advancedmath #mathematics #patrickJMTmath #changethevariablesinhomogeneous #YouTubeMath #highermath

[pacrat]

Seriously, if it wasn't for this guy I would not be studying engineering.

[TheSkyManRules]

Your ability to break a complex problem down into simple and understandable terms is why thousands of students watch your videos when in dire need of mathematical help. One of the most annoying things about online teaching videos is when the video maker assumes that the consumer understands a step and jumps ahead. This can be frustrating, as often times I am simply misunderstanding one simple concept (missing a minus sign or something dumb). Thank you for working through each problem completely and allowing students like me to get a better understanding of differential equations.

[joshpoe]

honestly you may have just saved my life... DE is one of the most complicating subjects. plus my teacher explains it in ways that make no since and are very disorganized. Thank YOU thank YOU thank YOU

[davidontiveroz]

Patrick just makes so math soothing, he could convince me to do anything with that beautiful innocent siren voice

[WesMan]

You have a gift for making these topics 10x less intimidating. THANK YOU!

[jingyiwang]

I started to watch your series of videos since I took my first calculus class in university. I will take ODE next semester and I am trying to preview for that class this summer. I have stuck at this point for a really long period of time. You have offered a really detailed and clear explanation about how to do such kind of problem. I am really grateful for your help!😁

[KLaRue]

I want to thank you for all of your videos. You've helped make being a nontraditional math major possible! With three kiddos and a husband, it is difficult to find time to study in-depth. If it wasn't for your videos, I would not have made it through calculus II. I've had a two-year break from calculus and I am now currently in differential equations... needless to say, I AM RUSTY with my calculus skills and am having to come back here to be saved. THANK YOU, THANK YOU, THANK YOU!!!!

[kevinmatheka]

Suddenly I am enjoying DEs. Thanks alot

[ghosthunter]

just watched more than 5 videos to learn this rule! I have got nothing..now I am watching your video..this rule is now like a piece of cake..hats off 🤗

[pancakeofdestiny]

PatrickJMT, you are the best as always!

[YoshiPeach]

I love differential equations right now. It is so crazy with the methods in the beginning, but when you can see the big picture it is interesting. These videos help reinforce and become more precise with DE, thanks!

[TedBundyJr]

this method is easier than the method my professor showed me. thanks, patrick!

[DianaMuturia]

dude, you just saved me about 15 points in my exam this morning! I couldn't figure it out till i watched this and practiced before heading to school for the exam

[faustdesrosiers]

I think you're the incarnation of either Newton or Leibniz. Thank you, so comprehensive

[xenachan]

What would I do without you?😭thank so much

[dkRun]

this video (like most if not all) has been very helpful to countless persons. keep up the good work

[DSolymanH]

Here we see two variables, x and y, both raised to the first power. Now if you had two x variables multiplied by one another, you know the result is x^2 and of the 2nd degree. The same holds true if you multiply x and y, as they are both variables but they happen to be different unknowns. Since x and y are both 1st degree variables, their multiplied resultant is xy where each single variable is of the 1st degree, but the combination are of the 2nd degree. Hope that helped.

[antoniofigaro]

I would like to thank you
You just saved me from failing a course

[laurentlorquet]

You are a magician, i dont know why my teacher never taught it like this before.. so much easier!!

[mcgarybreik]

from one pat to another, many many thanks for your math videos!