filmov
tv
integral of csc(x), the standard result, calculus 2 tutorial

Показать описание
Integral of csc(x), the standard result, calculus 2 tutorial
----------------------------------------
#blackpenredpen #math #calculus #apcalculus
----------------------------------------
#blackpenredpen #math #calculus #apcalculus
integral of csc(x), the standard result, calculus 2 tutorial
Integral of csc(x), without that trick!
Integral of csc(x)
Integral of csc(x) via Weierstrass substitution
Why the integral of csc x has 'different' solutions
Integrating Cosec(x) | csc(x)dx
Integral of cscx | Integration of cosecant x
INTEGRAL of csc(x) that makes sense
Shortened Integral of cscx
Integral of cscx secx ❖ Calculus 1
Integral of ∫csc(x)dx
Integrals#13 ~ Integral csc x dx ~ Like You've Never Seen It Before...W/Cray-cray Substitution ...
integral cscx dx integral csc^2x dx
Integral of csc(x)*(cot(x) - csc(x))
integral of csc x dx
Integral of sec(x) & integral of csc(x), the usual trick
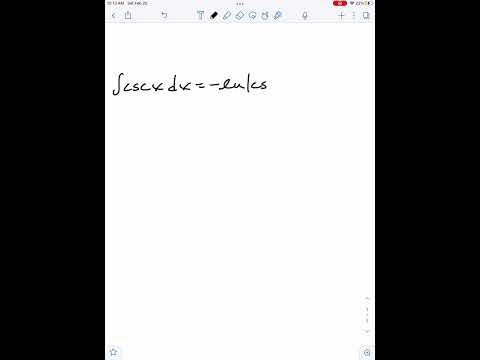
Integral of cscxdx... Standard formula for Integration of cosecx dx...
10. Proof integral cscx = ln(cscx - cotx)
Integral of csc(x)
integral of x(cscx)(cotx)
integral cscx
Integral of sqrt(1 + csc x) dx
Integral of sec(x)csc(x) dx (Integral of trigonometric functions)
Integral of -csc(x)cot(x)/sqrt(100 - csc^2(x))
Комментарии
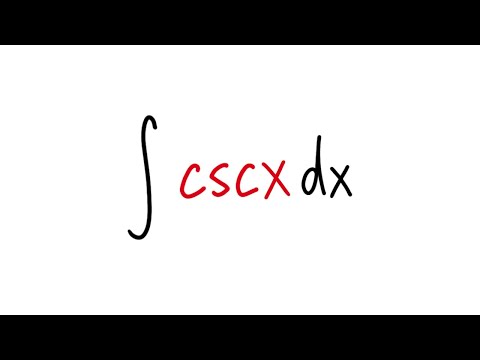 0:03:46
0:03:46
 0:04:00
0:04:00
 0:02:36
0:02:36
 0:00:47
0:00:47
 0:17:44
0:17:44
 0:08:33
0:08:33
 0:03:00
0:03:00
 0:04:18
0:04:18
 0:00:25
0:00:25
 0:05:17
0:05:17
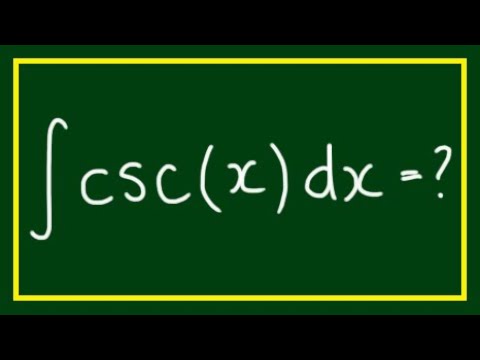 0:15:30
0:15:30
 0:01:08
0:01:08
 0:08:07
0:08:07
 0:02:10
0:02:10
 0:03:08
0:03:08
 0:04:41
0:04:41
 0:05:08
0:05:08
 0:05:24
0:05:24
 0:02:05
0:02:05
 0:01:31
0:01:31
 0:04:54
0:04:54
 0:03:20
0:03:20
 0:01:31
0:01:31
 0:02:44
0:02:44