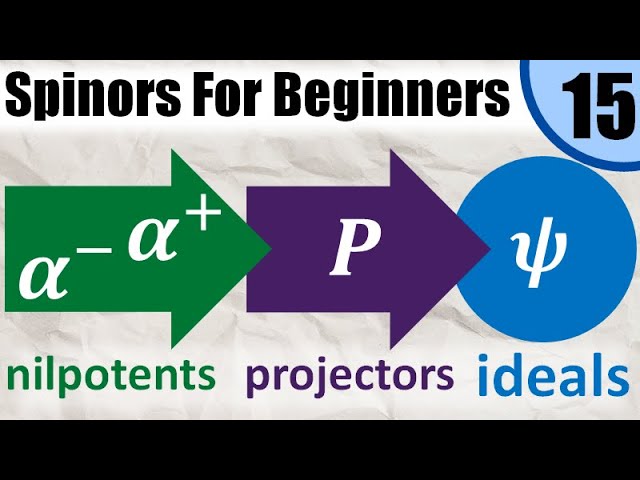
Spinors for Beginners 15: Nilpotents, Fermions, and Maximally Isotropic Subspaces

0:00 - Introduction
0:53 - Creation and Annihilation Operators (Bosons)
2:14 - Fermions
4:58 - Nilpotents
7:14 - Projectors
10:46 - Example in Cl(1,3)
14:18 - More Nilpotents
16:11 - Maximally Isotropic Subspaces
21:02 - Generalizing to C(n,0) and Cl(p,q)
23:57 - Example in Cl(2,0)
26:20 - Conclusion

[eigenchris]

There's a bad typo at 1:30, the raising operator should have a - sign instead of a + sign. Sorry about that.

[muonneutrino_]

This series has become something I binge watch everyday during my free time 😭 Thank you so much for making these videos <3

[nice]

Amazing so far, can't wait for you to get into the lie algebra stuff

[wafikiri_]

At 1:30, the raising and lowering operators are shown to equal each other. I would guess they should differ in the sign of their respective imaginary parts. Am I wrong?
Edit: corrected "operations" (should have been "operators" but somehow my fingers mistyped it.)

[roxashikari]

Minor correction:
11:25 defines U = \gamma_{t} + \gamma_{z}, but at 11:30 you have UU = (\gamma_{t} + \gamma_{x})(\gamma_{t} + \gamma_{x}) instead.

[zaitzerzazza]

Your doing great, i am looking forward to christmas vacation where i will properly watch these and take notes❤

[cmilkau]

24:26 If you just take 1=1, i=I, σ1 = σx, σ2=σy, the other mappings immediately fall into place by multiplication. The main thing to remember is that you need to consider V and iV separately to honour that real algebras don't allow multiplying by i (you need to use the pseudoscalar).

[AMADEOSAM]

Very good and impressive work! Keep on going …

[eelcj]

Thank you for these videos.
sure about the raising and lowering operators at 1:33? they are the same.

[cmilkau]

17:00 When building a descending chain of ideals using projectors, you need to be careful each projector you add is *not* orthogonal to the product you already have, else you get the trivial ideal (you "skipped" the minimal ideals). It's kinda obvious when you think about it, as this is basically the definition of orthogonal projectors: they multiply to zero, and the zero projector is the one taking everything to zero when multiplied.
EDIT: a slightly less destructive situation that also can occur is that multiplying the projector doesn't change the product, but you haven't reached a minimal ideal yet.

[maxqutekerman]

Lol. Just as I started typing comment about this video obviously being influenced by Cohl Furey works, you mentioned her explicitly.
Any plans of making a video on her Cl(8) model?

[enotdetcelfer]

Got super excited at the notification and then saw it was down, did you have to make a correction? :P Glad it's back, this made my weekend!

[TheJara]

Me too!! I realy hope he will take it to higher level!! I even purchased some books on about Lie ...which I can't even flip few pages ..feels like out of my element 😊

[MGoebel-ce]

For 11:32, i am trying to picture that in matrix representation and fail to understand how this then squares to zero - isn’t that [[1, -1], [-1, 1]]^2?

[tommasoantonelli]

Very elegant video as always! :)
I was wondering how this formalism of nilpotent vectors relates to Newman-Penrose formalism in GR, because in that formalism you are also required to choose a tetrad of null vector fields on your manifold (organized in two pairs), which have to be cross normalized in a similar fashion to what you're doing here. In that formalism you also have to complexify your tangent bundle in order to allow to write down two pairs that work, exactly what you're doing here. I also recall that the Newman-Penrose formalism is how one can think about spinors on a manifold, so the connection seems clear to me. What do you think?

[ko-prometheus]

Can you tell me what kind of software, what programs are available to perform the calculations you have outlined?

[GiordanoGaudio]

Amazing video! I often see the conversation phrased in terms of projectors or in terms of nilpotents, but I don't think I've ever seen a clear equivalence of these two ways of speaking. I 100% get the complex case for any signature, the problem I am having trouble with is the case of the real algebra. Is there a similar construction of spinors in arbitrary real Clifford algebras Cl(p, q, R) (you mentioned that there would be an appendix on that, will it come before the Lie algebra section or will you end the section on Clifford algebras with this construction?)

[pyrouscomments]

1:30 Nice half step for the ground state

[Kram]

Cohl Furey is the one working on connecting particle physics to like R x C x H x O, right? Where O is somehow related to quarks?
Since clifford algebras are associative in the usual geometric, product, they won't directly produce octonions but afaik there is a way to have an octionion style product anyway, right?
I wonder what it, like, "means", that stuff is non-associative. Non-commutativity seems to essentially correspond to interaction / measuring. If stuff commutes, the order doesn't matter, so the involved objects basically don't interact at all. They go through orthogonal processes, basically.
But what does it mean in this sense, when stuff fails to associate?

[eduardoGentile]

Can you build a nilpotent in Cl(3, 0, R)? If not, then is it more useful to use is Cl(2, 0, C)?