Probability Distributions A-Z! | Normal vs. Standard Normal vs. Poisson vs. Binomial vs. Bernoulli

In this video, we will illustrate the difference between Normal, Standard Normal, Poisson, Bernoulli and Binomial distributions.

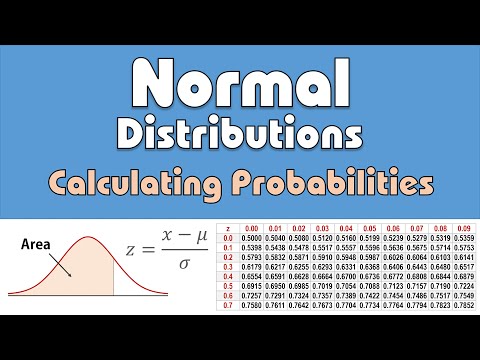
NORMAL DISTRIBUTION
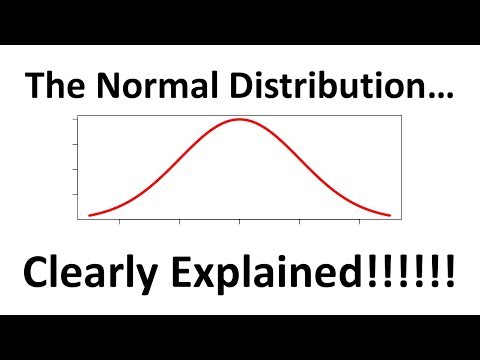
A normal distribution is known as the bell curve because it looks like a bell!
Normal distribution is defined by its mean and standard deviation.
Normal distribution is centered about its mean, with standard deviation indicating its spread.

STANDARD NORMAL DISTRIBUTION
The Standard Normal distribution curve has:
Mean = 0
Standard deviation = 1

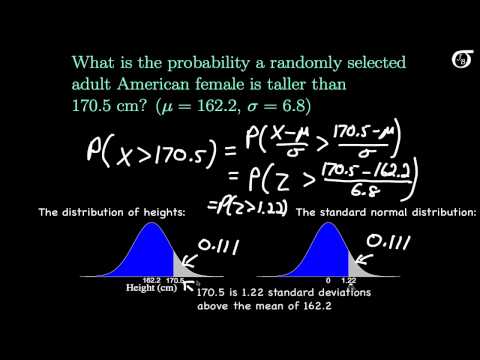
We can convert data that is normally distributed to make it follow a standard normal by subtracting the mean and dividing by the standard deviation.

For normally distributed data:
- 68.3% of observations are within 1 standard deviation from the mean (-1,1).
- 95% of observations are within 2 standard deviations of the mean (-2,2).
- 99.7% of observations are within 3 standard deviations of the mean, interval (-3,3).

POISSON DISTRIBUTION
Poisson distribution is the discrete probability distribution of the number of events that occur in a specified period of time.

Poisson distribution is extremely helpful for planning purposes as it enable managers to analyze customer behavior as they visit a restaurant or store for example.

BINOMIAL DISTRIBUTION
A binomial distribution measures the probability of success or failure outcome when the experiment is repeated several times (ex: outcomes of taking the AWS Machine Learning exam is: pass or fail).

There are only two possible outcomes with fixed probabilities summing to one.

For example, a coin toss has only two possible outcomes: heads or tails where the probability of each event is exactly = 0.5.

BERNOULLI DISTRIBUTION
Bernoulli distribution is a special case of the binomial distribution for n = 1.

Simply put, it is a binomial distribution with a single trial (one coin toss).

Bernoulli distribution is a discrete probability distribution has only two outcomes (“Success” or a “Failure”).

For example, when coin flipping:
Probability of head (success) = 0.5
Probability of tail (failure) = 1 – P = 0.5

The probability of a failure is labeled on the x-axis as 0 and success is labeled as 1.

I hope you guys enjoyed this video and found it useful and informative.

Please subscribe to my channel for more videos!

Thanks,
Ryan

#Distributions #NormalDistribution #Bernoulli #Binomial #poisson

[TomekMachalewski]

I really liked that you provided few examples for those more difficult distributions. What I would love to see is an explanation of where do the probability formulas in Poisson and Binomial distributions come from. I see that they are combinatorics formulas, but I'll have to check euler^(- miu) in Poisson.

[Golden_Tortoise]

In Poisson, the a time interval needs to be stated. So Probability of 7 customers in 1 hour or in one day. Or the probability of 0 customers of in 1 hour or one day.

[thomasanderson]

Can't you use the poisson formula to calculate interval event results like P(x<= 3)?

[JJ_TheGreat]

What about Geometric, Hypergeometric, Negative Binomial, Exponential, Uniform Discrete, Uniform Continuous, etc. Distributions?

[mahdi]

brother you have no idea how much this assisted me thanks a lot.

[mayurgite]

Thank you so much for very clean explanation 🙏🙏

[melodymemories]

Can anyone say why perticularly 68.3%, 95%, 99.7%

[oomeshheerauman]

How u got 2.71828 for the Poisson distr?

[عليمحمد-جصج]

السلام عليكم
استمر يا دكتور
وشكرا جزيلا علي المحتوي الرائع