filmov
tv
Find the VELOCITY Of A Pendulum at It's Lowest Point

Показать описание
I am not sponsored by Sharpie... yet.
A common application of the work energy theorem, this problem looks at the conservation of energy as gravitational potential energy is converted into kinetic energy through work by gravity.
The mass begins with some potential energy relative to its lowest point. But as the pendulum is released gravity does work on the pendulum to convert potential energy into kinetic energy.
At any point, if you can find how much potential energy has been lost, then you can solve for the kinetic energy and there for solve for the velocity of the pendulum.
Just for fun, If you try to solve for the velocity of the pendulum at a point higher than the release height, you will get an imaginary number. In mechanics the result of an imaginary number means it cant happen... That is to say the ball can not go higher than that from which it was released.
This problem commonly comes up in physics including AP Physics 1 and AP Physics C
A common application of the work energy theorem, this problem looks at the conservation of energy as gravitational potential energy is converted into kinetic energy through work by gravity.
The mass begins with some potential energy relative to its lowest point. But as the pendulum is released gravity does work on the pendulum to convert potential energy into kinetic energy.
At any point, if you can find how much potential energy has been lost, then you can solve for the kinetic energy and there for solve for the velocity of the pendulum.
Just for fun, If you try to solve for the velocity of the pendulum at a point higher than the release height, you will get an imaginary number. In mechanics the result of an imaginary number means it cant happen... That is to say the ball can not go higher than that from which it was released.
This problem commonly comes up in physics including AP Physics 1 and AP Physics C
How to Calculate Velocity
Find the VELOCITY Of A Pendulum at It's Lowest Point
How to Solve a Velocity, Distance, and Time Problem (Easy)
Calculate Average Velocity
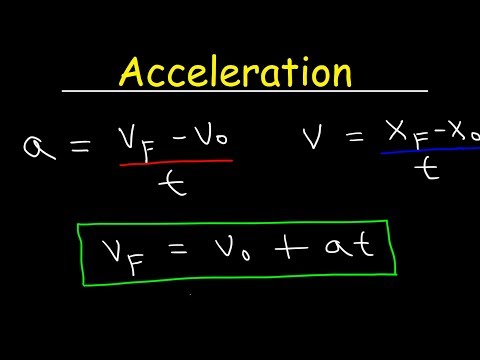
Physics - Acceleration & Velocity - One Dimensional Motion
Instantaneous speed and velocity | One-dimensional motion | Physics | Khan Academy
Finding the instantaneous velocity from a velocity vs time graph
how to find the average velocity and instantaneous velocity? Calculus 1 tutorial
How to Fix VelocityOne Flight Stick Controls in MS Flight Simulator 2024 – Step by Step Guide
Finding Velocity from a Position vs Time graph, Part 1
How to Calculate Velocity
How to calculate velocity from a position vs time graph
Average Velocity and Instantaneous Velocity
How to Find the Speed, Velocity, and Acceleration Given the Position Function(Vector Valued)
Velocity time graph
Position/Velocity/Acceleration Part 1: Definitions
What is Velocity? - Full Concept of Velocity - Physics | Infinity Learn
Calculate Speed & Velocity Easily: Step-By-Step Tutorial - Practice Problems | Physics
How to Calculate the average velocity from a position vs time graph
How to find the average velocity of a ball falling
❖ Position, Velocity, Acceleration using Derivatives ❖
Find the Velocity, Speed, and Acceleration Given the Vector Valued Position Function
Calculating Acceleration From a Velocity-Time Graph - GCSE Physics | kayscience.com
How to find the instantaneous velocity! Calculus 1 tutorial
Комментарии
 0:03:26
0:03:26
 0:04:12
0:04:12
 0:01:12
0:01:12
 0:02:15
0:02:15
 0:18:58
0:18:58
 0:04:38
0:04:38
 0:00:29
0:00:29
 0:03:48
0:03:48
 0:01:54
0:01:54
 0:02:11
0:02:11
 0:01:38
0:01:38
 0:01:44
0:01:44
 0:19:19
0:19:19
 0:02:48
0:02:48
 0:08:02
0:08:02
 0:07:40
0:07:40
 0:06:52
0:06:52
 0:04:16
0:04:16
 0:01:19
0:01:19
 0:02:17
0:02:17
 0:08:46
0:08:46
 0:03:03
0:03:03
 0:05:23
0:05:23
 0:05:30
0:05:30