filmov
tv
Mean and Variance of OLS Estimators in Matrix Form Linear Regression
Показать описание
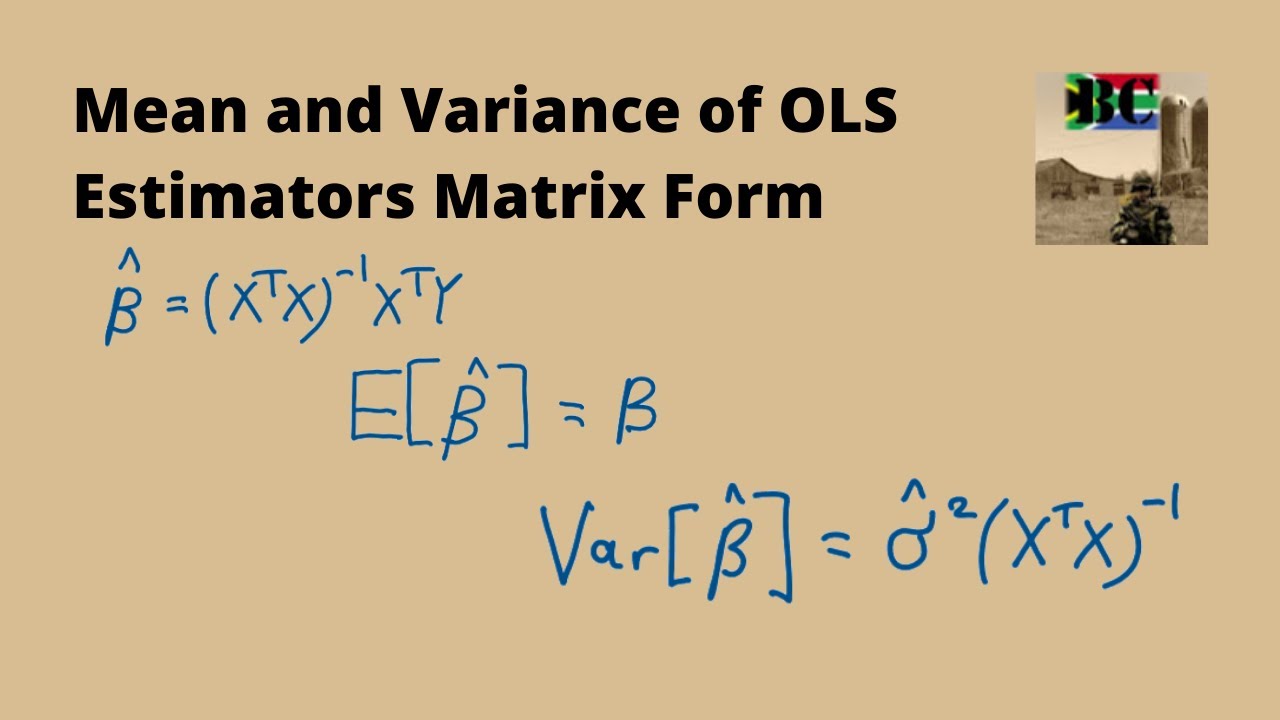
This video follows from the previous one covering the assumptions of the Linear Regression Model in the Matrix Formulation to derive and show the properties of the OLS estimators, E[B] and Var[B].
In this video I derive and show that under the assumptions of the Linear Regression Model, the OLS Estimators are unbiased (E[B] = B) and that the Variance of B (Var(B) = sigma squared*(X'X)^-1).
#Econometrics
#Regression
#OLS
0:00 Introduction
0:16 Derive and show that E[β^] = β
3:05 Derive Var[β^]
In this video I derive and show that under the assumptions of the Linear Regression Model, the OLS Estimators are unbiased (E[B] = B) and that the Variance of B (Var(B) = sigma squared*(X'X)^-1).
#Econometrics
#Regression
#OLS
0:00 Introduction
0:16 Derive and show that E[β^] = β
3:05 Derive Var[β^]
0:10:54
Deriving the mean and variance of the least squares slope estimator in simple linear regression
0:08:38
Mean and Variance of OLS Estimators in Matrix Form Linear Regression
0:02:16
Variance of the OLS estimator (Part 1)
0:07:10
Variance of OLS estimators - part one
0:06:17
Variance of OLS estimators | Linear Regression Model | Econometrics | Harpreet Kaur | Ecoholics
0:17:18
Variance and Standard Error of OLS Estimates | Introductory Econometrics 11
0:09:55
(Un)Conditional mean and variance of the OLS beta estimate || Econometrics || U/Grad || MPhil || PhD
0:06:56
ECO375F - 2.6 - Variance of the Slope Estimator (β1)
0:17:39
Basic Econometrics - The variance of the OLS estimator
0:14:03
The variance of the OLS estimators
0:17:08
3.4 Variance of OLS estimators
0:05:32
Variance of Least Squares Estimators - Matrix Form
0:03:35
3.17 - The Variance of OLS Estimators
0:03:52
Estimated variance of OLS estimators - intuition behind maths
0:09:40
Estimating the variance of the OLS estimators
0:08:56
Find the Value of OLS estimators Linear Regression Model | Mathematical Economics | Ecoholics
0:39:09
Econometrics 31: Mean and Variance of OLS Estimators.
0:30:11
Least Square Estimators - Variance of Estimators, b0 and b1, Proof
0:03:48
10.3) OLS: Variance
0:06:52
Variance of OLS Intercept
0:32:03
ECO375F - 1.0 - Derivation of the OLS Estimator
0:12:13
Deriving the least squares estimators of the slope and intercept (simple linear regression)
0:07:39
Introduction to residuals and least squares regression
0:12:16
2.5b1 Variances of OLS estimators Part 1
Комментарии