filmov
tv
PreCalculus | Two absolute value inequalities.
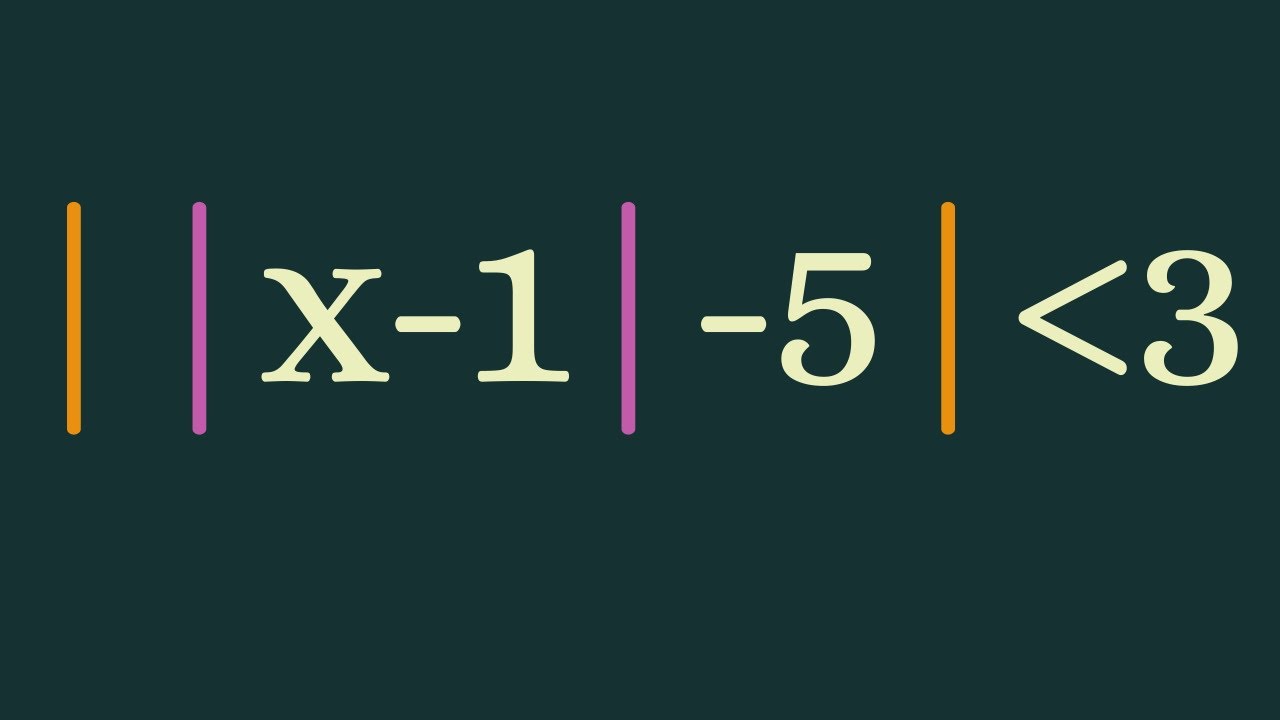
Показать описание
We solve a few "trickier" inequalities involving absolute values.
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
a very TRICKY double absolute value equation
PreCalculus | Two absolute value inequalities.
Two Exercises in ABSOLUTE VALUES and DISTANCE - Precalculus
Worldwide Pre-Calculus: Solving Equations with two Absolute Values (ch1.8 #1.8.1)
PreCalculus Double Absolute Value Equation and Inequality
DOUBLE Absolute Value Equations problem ! ! ! ! !
Pre-Calculus - Solving a linear inequality with an absolute value
How To Solve Absolute Value Equations, Basic Introduction, Algebra
Precalculus: Absolute Value Examples
How to understand ABSOLUTE VALUES and DISTANCE - Precalculus
Solving Absolute Value Inequalities and Equations | Pre-Calculus
Solving Equations w/ Absolute Values Algebraically ('By Cases')
A Look At Absolute Value ❖ Algebra ❖ Pre-Calculus
Double Absolute Value Equation
Precalculus | Algebra | Absolute Value Equations | Case 1 and 2
Pre-calculus: Solving Absolute Value Equations
Transformation of the Absolute Value Function
Precalculus 12: 2.1 Absolute Value Functions
Circles and Absolute Values (Mod 2.6-2.7i) Precalc
PreCalculus Notes 0 2 Absolute Value Inequalities
PreCalculus - Algebra Fundamental Review (2 of 80) The Absolute Value: Examples
Solving Inequalities with Two Absolute Values by Testing Points
Inequality with Two Absolute Functions Using Two Different Strategies
Equation with Double Absolute Value Step by Step part 1/2 - Precalculus - Calculus - Algebra - Math
Комментарии
 0:04:59
0:04:59
 0:09:05
0:09:05
 0:06:07
0:06:07
 0:01:06
0:01:06
 0:15:10
0:15:10
 0:11:09
0:11:09
 0:07:01
0:07:01
 0:04:21
0:04:21
 0:06:29
0:06:29
 0:13:45
0:13:45
 0:17:53
0:17:53
 0:09:42
0:09:42
 0:05:25
0:05:25
 0:06:40
0:06:40
 0:17:24
0:17:24
 0:04:33
0:04:33
 0:04:30
0:04:30
 0:16:29
0:16:29
 0:12:27
0:12:27
 0:12:31
0:12:31
 0:03:13
0:03:13
 0:11:17
0:11:17
 0:14:43
0:14:43
 0:09:25
0:09:25