filmov
tv
finding a harmonic conjugate in complex analysis
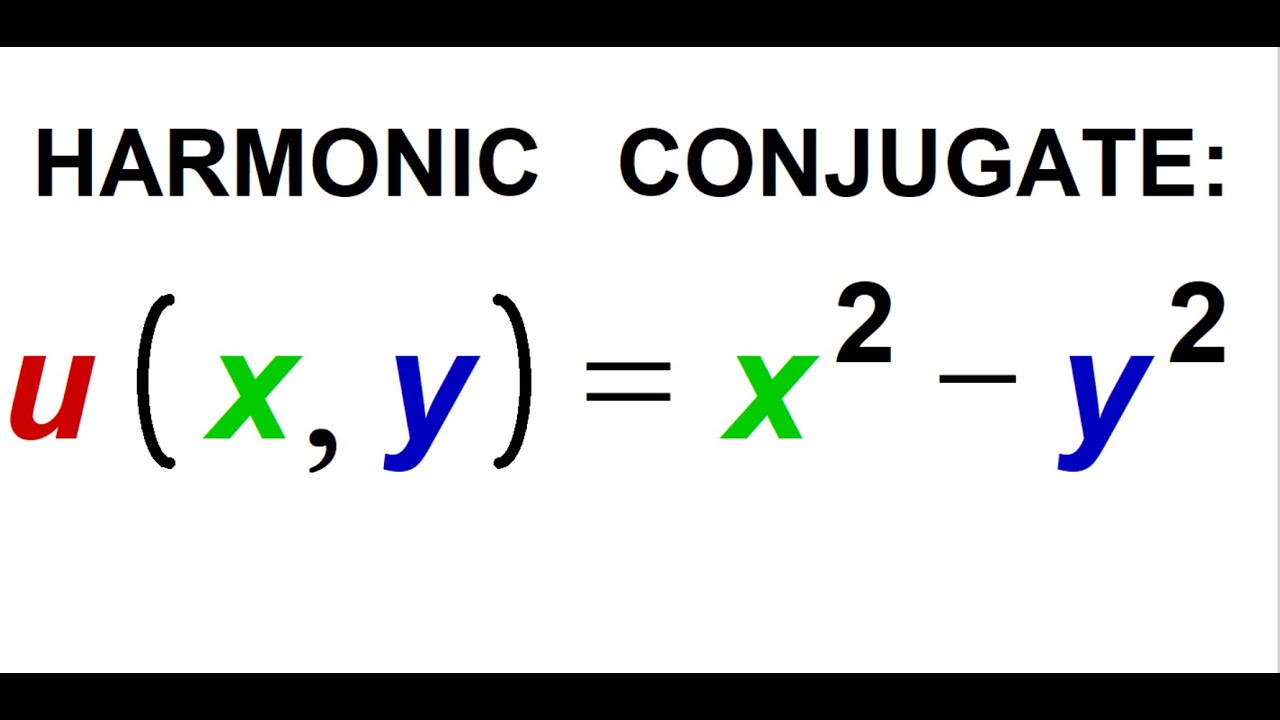
Показать описание
In this video, we will explore how to find the harmonic conjugate of a function in complex analysis. We will use the example function U(x,y) = x² - y² to demonstrate the process. First, we will remind ourselves of the Cauchy-Riemann equations, which state that F_y = iF_x. From there, we can relate the real and imaginary parts of the equations to find the harmonic conjugate V(x,y).
We will differentiate U(x,y) with respect to y to find the value of V(x,y), which will be -2yx + K. We will also use the Cauchy-Riemann equations to find U_x, which equals V_y, and differentiate this to solve for K. Putting all of the pieces together, we arrive at the harmonic conjugate V(x,y) = 2yx + K.
🔍 Keywords complex analysis, harmonic conjugate, Cauchy-Riemann equations, imaginary part, real part, differentiate, anti-differentiate, x², y²
🤔 Internet Questions
1️⃣ What are Cauchy-Riemann equations?
- Cauchy-Riemann equations are a set of equations that relate the real and imaginary parts of a complex function.
2️⃣ What is a harmonic conjugate?
- A harmonic conjugate is a complex function that satisfies the Cauchy-Riemann equations.
3️⃣ How do you find the harmonic conjugate of a function?
- To find the harmonic conjugate of a function, you need to differentiate the function with respect to y and use the Cauchy-Riemann equations to relate the real and imaginary parts of the function.
4️⃣ What is complex analysis used for?
- Complex analysis is used to study complex functions and their properties, such as analyticity and singularities.
5️⃣ What is the difference between a real function and a complex function?
- A real function maps real numbers to real numbers, while a complex function maps complex numbers to complex numbers.
6️⃣ What is the difference between a derivative and an anti-derivative?
- A derivative measures the rate of change of a function, while an anti-derivative (also called an indefinite integral) is the reverse operation of differentiation.
7️⃣ What is the significance of the Cauchy-Riemann equations?
- The Cauchy-Riemann equations are significant because they provide necessary and sufficient conditions for a function to be complex differentiable.
We will differentiate U(x,y) with respect to y to find the value of V(x,y), which will be -2yx + K. We will also use the Cauchy-Riemann equations to find U_x, which equals V_y, and differentiate this to solve for K. Putting all of the pieces together, we arrive at the harmonic conjugate V(x,y) = 2yx + K.
🔍 Keywords complex analysis, harmonic conjugate, Cauchy-Riemann equations, imaginary part, real part, differentiate, anti-differentiate, x², y²
🤔 Internet Questions
1️⃣ What are Cauchy-Riemann equations?
- Cauchy-Riemann equations are a set of equations that relate the real and imaginary parts of a complex function.
2️⃣ What is a harmonic conjugate?
- A harmonic conjugate is a complex function that satisfies the Cauchy-Riemann equations.
3️⃣ How do you find the harmonic conjugate of a function?
- To find the harmonic conjugate of a function, you need to differentiate the function with respect to y and use the Cauchy-Riemann equations to relate the real and imaginary parts of the function.
4️⃣ What is complex analysis used for?
- Complex analysis is used to study complex functions and their properties, such as analyticity and singularities.
5️⃣ What is the difference between a real function and a complex function?
- A real function maps real numbers to real numbers, while a complex function maps complex numbers to complex numbers.
6️⃣ What is the difference between a derivative and an anti-derivative?
- A derivative measures the rate of change of a function, while an anti-derivative (also called an indefinite integral) is the reverse operation of differentiation.
7️⃣ What is the significance of the Cauchy-Riemann equations?
- The Cauchy-Riemann equations are significant because they provide necessary and sufficient conditions for a function to be complex differentiable.
Комментарии
 0:06:00
0:06:00
 0:04:16
0:04:16
 0:02:57
0:02:57
 0:16:01
0:16:01
 0:04:34
0:04:34
 0:06:21
0:06:21
 0:06:06
0:06:06
 0:17:14
0:17:14
 0:15:35
0:15:35
 0:06:55
0:06:55
 0:30:01
0:30:01
 0:11:43
0:11:43
 0:10:43
0:10:43
 0:08:40
0:08:40
 0:33:30
0:33:30
 0:14:06
0:14:06
 0:19:34
0:19:34
 0:09:57
0:09:57
 0:12:25
0:12:25
 0:14:43
0:14:43
 0:06:35
0:06:35
 0:28:51
0:28:51
 0:40:03
0:40:03
 0:25:00
0:25:00