Harmonic functions | Harmonic conjugate | Complex Analysis #3

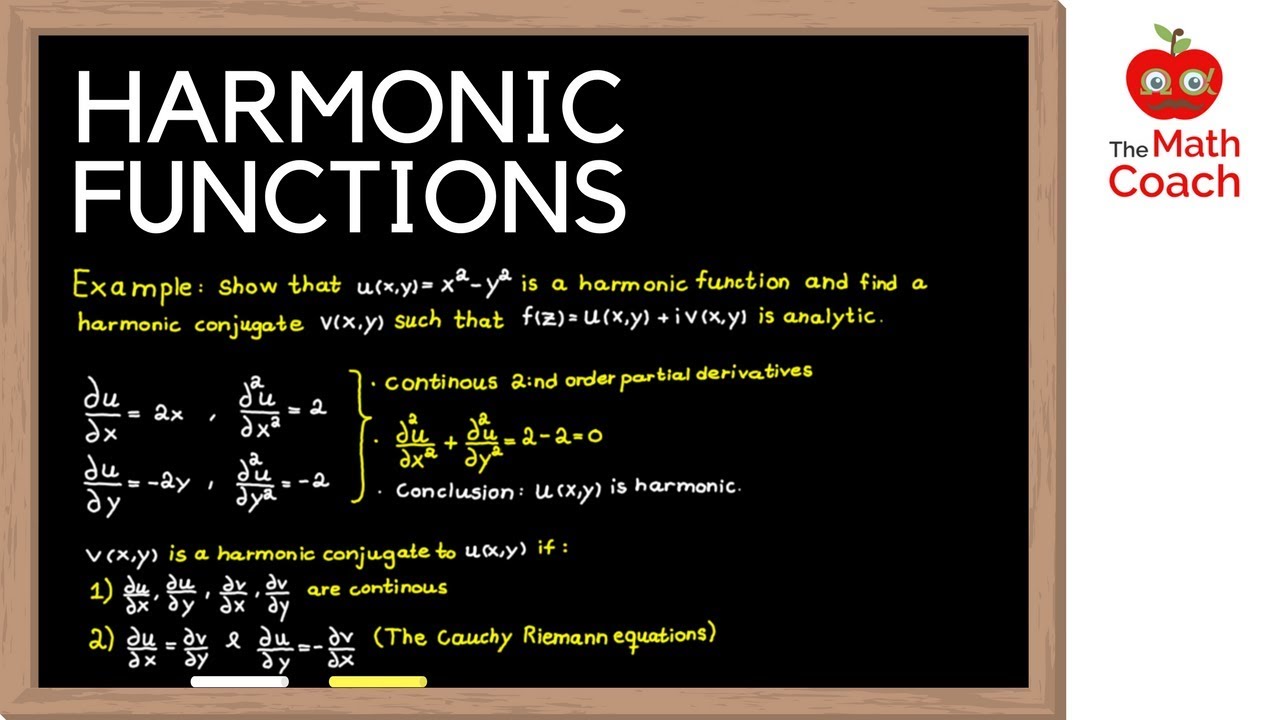
The definition of a Harmonic function, Harmonic conjugate function and how Analytic functions and Harmonic functions are related through some theorems. Examples for each concept are included.

LINK TO COMPLEX ANALYSIS PLAYLIST

LINK TO CANVAS

SUPPORT
Consider subscribing and liking if you enjoyed this video or if it helped you understand the subject. It really helps me a lot.

IMPORTANT LINKS

CONCEPTS FROM THE VIDEO
► Harmonic Functions
Is a real-valued function u(x,y) with continuous second partial derivative which satisfies Laplace's equation.

► Harmonic Conjugate Functions
The harmonic conjugate to a given function u(x,y) is a function v(x,y) such that f(x,y) = u(x,y) + iv(x,y) is differentiable.

► Complex Differentiability
A function f(z) = u(x,y)+iv(x,y) is differentiable in a region R if and only if the following conditions are fulfilled in R:
1) du/dx, dv/dy, du/dy, dv/dx are continous
2) du/dx, dv/dy, du/dy, dv/dx satisfies the Cauchy Riemann Equations
The derivative is defined as f'(z) =du/dx + i*dv/dx=du/dy - i*dv/dy

► Analytic Continuation
It provides a way of extending the domain over which a complex function is defined. Let f_1 and f_2 be analytic functions which are defined on the domains d_1 and d_2, if f_1 = f_2 is true in the intersection of the domains then f_2 is called an analytic continuation of f_1 to d_2 and vice versa. This analytic continuation is unique if it exists.

TIMESTAMPS
Definition: Harmonic functions: 00:00 - 00:23
Theorem: Analytic function to Harmonic function: 00:23 - 00:48
Theorem: Analytic function to Harmonic function: 00:48 - 01:13
Definition: Harmonic functions: 01:13 - 01:35
Example: 01:35 - 01:59

SOCIAL

HASHTAGS
#TheMathCoach #ComplexAnalysis

[emlmm]

I still don't understand why analytic continuation works.

[galibrabat]

I have an exam tomorrow and this is one of the best youtube videos i have seen today. Thank you so so much sir!

[rodrigogazolam]

Great work, thanks for sharing all these important topics in a concise and simple way!

[selenalin]

you are awesome! you helped me survive from my midterm

[PunmasterSTP]

This was another incredible video, and I think that analytic continuation is *very* cool!

[pzz]

Your voice and accent are both so soothing! Where are you from?

[Tengdbuddy]

Thank you for making this great video.

[yagzyalcntas]

that is just perfect it gives me all the things i wanted to understand thank you! I couldnt link these equations and fefinitions to each other but you summed it up nicely :) and are you japanese? you sound like japanese

[zeroeinsof]

Let me try a first question so you can decide if is worth your time...(I am not a mathematician, most is self-taught).

U(x, y) and V(x, y) are harmonic functions. Both represent a 2-dimensional surface. Is it correct to say that satisfying the Cauchy Riemann Equations implies that U(x, y) and V(x, y) define the same surface and that V(x, y) is derived from the surface of U(x, y) by rotating the x, y -axis with -PI/2 (x-axis becomes y-axis and y-axis becomes -x-axis)? If so, can I then say that an analytic function is a (sort of) linear combination of 1 and i with two harmonic functions U and V as coefficients?

To make sure: The random function Phi(x), which turns out to be a constant, only represents a vertical shift along the z-axis?

Thank you

[bendavis]

Hey at 3:58 I'm a little confused how you got from du/dx=2x to the next step where v(x, y)=2xy+phi(x). I see where you plugged in the derivative, but I'm still confused where the 'y' comes from. And also I'm a little confused by the addition of phi(x) at the end of the equation. Thanks for the great video!

[nikkikumari]

I am understand your concept.... Thank you...

[tahseenomar]

In the last video you differentiated between a complex function being differentiable and a complex function being analytic; a complex function can be differentiable but not analytic (e.g. the example where it's differentiable only on the coordinate axes). In this video, however, you seem to be using the terms "analytic" and "differentiable" interchangeably. What am I missing?

[ShehzadiKanwal]

Continuous second order partial derivatives what does it mean Sir?

[marquez]

can you always use the trick set x=z and y=0 ?

[Charky]

I subscribed but why you have not uploaded in 2 years 😢

[safeegull]

I applied same procedure, I got wrong answer of every question

[emmalennie]

The videos are too fast. Good content, but presentation is way too first. Makes it unenjoyable 😢