filmov
tv
if `x^2+y^2 = t - 1/t` and` x^4 + y^4 = t^2 + 1/t^2 `then prove that `dy/dx = 1/(x^3y)`

Показать описание
This is the Solution of Question From RD SHARMA book of CLASS 12 CHAPTER CONTINUITY AND DIFFERENTIABILITY This Question is also available in R S AGGARWAL book of CLASS 12 You can Find Solution of All Question From RD SHARMA and R S AGGARWAL book from class 6-12 on our app DOUBTNUT for Free
#rdsharmasolution #CONTINUITYANDDIFFERENTIABILITY #rdsharmaclass12 #class12 #cbse #rdsharma #doubtnut
==== QUESTION TEXT ====
if `x^2+y^2 = t - 1/t` and` x^4 + y^4 = t^2 + 1/t^2 `then prove that `dy/dx = 1/(x^3y)`
=== DOWNLOAD DOUBTNUT TO ASK ANY MATH QUESTION ===
==== SCORE 100% WITH DOUBTNUT ====
Doubtnut App has video solutions of ALL the NCERT questions from Class 6 to 12 (including IIT JEE). You can also ask any Math question and get a video solution for FREE from a library of more than 1 Lakh Math Videos
==== FOLLOW US ON FACEBOOK ====
Doubtnut PAGE:
DOUBTNUT IIT JEE Group:
==== WATCH DAILY LIVE CLASSES ON YOUTUBE ====
5:30 PM - Class 9
6:30 PM - Class 10
7:15 PM - Class 11
8:15 PM - Class 12
9:15 PM - Class 13 (DROPPERS)
#rdsharmasolution #CONTINUITYANDDIFFERENTIABILITY #rdsharmaclass12 #class12 #cbse #rdsharma #doubtnut
==== QUESTION TEXT ====
if `x^2+y^2 = t - 1/t` and` x^4 + y^4 = t^2 + 1/t^2 `then prove that `dy/dx = 1/(x^3y)`
=== DOWNLOAD DOUBTNUT TO ASK ANY MATH QUESTION ===
==== SCORE 100% WITH DOUBTNUT ====
Doubtnut App has video solutions of ALL the NCERT questions from Class 6 to 12 (including IIT JEE). You can also ask any Math question and get a video solution for FREE from a library of more than 1 Lakh Math Videos
==== FOLLOW US ON FACEBOOK ====
Doubtnut PAGE:
DOUBTNUT IIT JEE Group:
==== WATCH DAILY LIVE CLASSES ON YOUTUBE ====
5:30 PM - Class 9
6:30 PM - Class 10
7:15 PM - Class 11
8:15 PM - Class 12
9:15 PM - Class 13 (DROPPERS)
If `x^2+y^2=t+1/t and x^4+y^4=t^2+1/(t^2)` then `(dy)/(dx)=`
Can You Prove that if x2+y2 is even, then x+y is even.
Find first and second derivative for x = t^2 -3t, y = t^3 in terms of t. Parametric equations
Total Derivative || 𝒖=𝒙^𝟐+𝒚^𝟐+𝒛^𝟐 || Partial Differentiation || 18mat21 || Dr Prashant Patil...
Find dy/dx if x=at^2, y=2at
Total Derivative || 𝒛=𝒙𝒚^𝟐+𝒙^𝟐 𝒚;𝒙=𝒂𝒕^𝟐;𝒚=𝟐𝒂𝒕 |Partial Differentiation| 18mat21 || Dr Prashant Patil...
if `x^2+y^2 = t - 1/t` and` x^4 + y^4 = t^2 + 1/t^2 `then prove that `dy/dx = 1/(x^3y)`
Implicit Differentiation of x^2+y^2=r^2
The Hardest Math Problem on the SAT Exam #maths #shorts
If `x=at^2` and y=2 at then find `dy/dx`
Find 𝒅𝒖/𝒅𝒕 as a total derivative if u = 𝒙^𝟐 + 𝒚^𝟐 + 𝒛^𝟐 , x = 𝒆^𝟐𝒕 , y = 𝒆^𝟐𝒕cos 3t , z = 𝒆^𝟐𝒕 sin 3...
Target 95 || Class 12 || If x2+y2=t−1/t and x4+y4=t2+1/t2, then prove that dydx=1/x3y.
Partial Differentiation || 𝑺𝒊𝒏^(−𝟏) [(𝒙^𝟐+𝒚^𝟐)/(𝒙+𝒚)] || vtu maths || Dr Prashant Patil...
u=x^2+y^2 x=at^2 y=2at find Әu/Әt PARTIAL DIFFERENTIATION
If x=2t/(1+t²) and y=(1-t²)/(1+t²), then dy/dx=? JEE Mains test series Differentiation
`x-2 = t^2, y = 2t` are the parametric equations of the parabola-
total differential coefficient|| problem 3|| partial differentiation
Partial derivatives - Laplace equation
Solving x²-y²=5 for x,y greater than 0
If x=a t^2, y=2 a t, then d^2 y/d x^2 is :(A) -1/t^2 (B) 1/2 a t^2 (C) -1/t^3 (D) -1/2 at^3
If `x = t^(2)` and `y = t^(3)`, then `(d^(2)y)/(dx^(2))` is equal to
Find the value of x and y
Solving an equation for y and x
If x=a(cost+log(tan(t/2))),y=asint then find dy/dx Sky Dhoni || SAQ
Комментарии
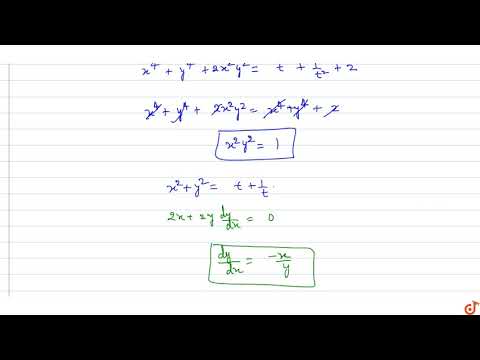 0:02:56
0:02:56
 0:02:32
0:02:32
 0:02:53
0:02:53
 0:07:01
0:07:01
 0:01:07
0:01:07
 0:08:15
0:08:15
 0:01:46
0:01:46
 0:00:59
0:00:59
 0:01:49
0:01:49
 0:09:25
0:09:25
 0:03:00
0:03:00
 0:06:55
0:06:55
 0:02:30
0:02:30
 0:02:47
0:02:47
 0:01:21
0:01:21
 0:12:09
0:12:09
 0:11:40
0:11:40
 0:04:17
0:04:17
 0:02:21
0:02:21
 0:01:57
0:01:57
 0:04:55
0:04:55
 0:03:47
0:03:47
 0:09:19
0:09:19