filmov
tv
Just Move The 4 #shorts

Показать описание
The best way to divide a certain class of numbers is to use absolutely no math at all. WHAT?!
It’s true -- you can get the job done simply by transposing the first digit to the end of the number. 410,256 is a small, accessible number to try it. Just slide the 4 from the first digit to the last digit and you’re all set… and it works with some much larger numbers, too.
In terms of mathematical value, these quirky numbers probably don’t lay the foundation for a groundbreaking Fields Medal-worthy discovery. But this is what recreational mathematics is all about: pushing and pulling on numbers to recognize patterns and curiosities that facilitate your ability to handle heavier-duty work, sort of like how poetry plays with words to express thoughts that *could* be written much more simply. It’s beautiful. And it’s just plain interesting.
#vsauce #education #maths
It’s true -- you can get the job done simply by transposing the first digit to the end of the number. 410,256 is a small, accessible number to try it. Just slide the 4 from the first digit to the last digit and you’re all set… and it works with some much larger numbers, too.
In terms of mathematical value, these quirky numbers probably don’t lay the foundation for a groundbreaking Fields Medal-worthy discovery. But this is what recreational mathematics is all about: pushing and pulling on numbers to recognize patterns and curiosities that facilitate your ability to handle heavier-duty work, sort of like how poetry plays with words to express thoughts that *could* be written much more simply. It’s beautiful. And it’s just plain interesting.
#vsauce #education #maths
Just Move The 4 #shorts
If You Move, You Die 😱 [Part 1] [Movie Recap] #shorts #viral
My Last Move Is Your First Move Challenge | Triple Charm #Shorts
#shorts studio apartment in just 16 lakh/ready to move
Statue of Liberty Moving 😳 #shorts
When u try to make a move 🤣 #shorts
BEST LULULEMON SHORTS 2021! | Running, Yoga, On The Move, etc.
My First RED BRILLIANT MOVE!! #shorts
MOVE YOUR BODY!🎶 The dance and song is perfect😍 #shorts
Walking tree caught moving😳 #shorts
RATE THIS MOVE! 😅🩵 - #dance #trend #viral #funny #couple #shorts
Last move was tough. 😳 #shorts
When she did that move #shorts
only one move in 4 by 4 Rubik's cube #shorts #viral
ILLEGAL Move & WIN the Game! #shorts
Didn’t See That Move Coming! #shorts #viral #chess #memes
Bobby's First BRILLIANT MOVE!! 🤣 #shorts
Just MOVE! #shorts
1 move only #shorts
Move ONE Stick To Make Four Triangles #shorts
trombone player goes WILD with unexpected solo move #shorts
It only takes one move to complete this challenge!🤯 #Shorts
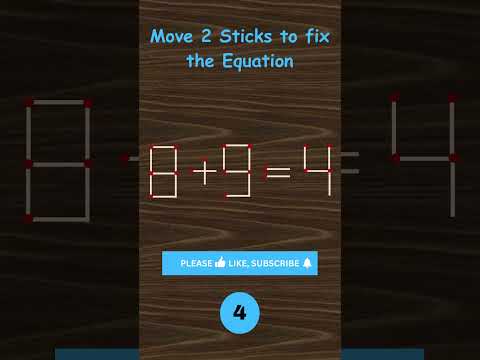
Mind Blowing Trick: Move Only 2 Matchstick | 8 + 9 =4 #shorts
Ultimate Chess Move! #shorts #chess #memes
Комментарии
 0:01:00
0:01:00
 0:01:00
0:01:00
 0:00:12
0:00:12
 0:01:00
0:01:00
 0:00:13
0:00:13
 0:00:49
0:00:49
 0:26:27
0:26:27
 0:00:38
0:00:38
 0:00:14
0:00:14
 0:00:24
0:00:24
 0:00:14
0:00:14
 0:00:36
0:00:36
 0:00:24
0:00:24
 0:00:10
0:00:10
 0:00:35
0:00:35
 0:01:01
0:01:01
 0:00:53
0:00:53
 0:00:12
0:00:12
 0:00:10
0:00:10
 0:00:31
0:00:31
 0:00:12
0:00:12
 0:01:00
0:01:00
 0:00:13
0:00:13
 0:00:14
0:00:14