filmov
tv
Topological spaces - some heavily used invariants - Lec 05 - Frederic Schuller
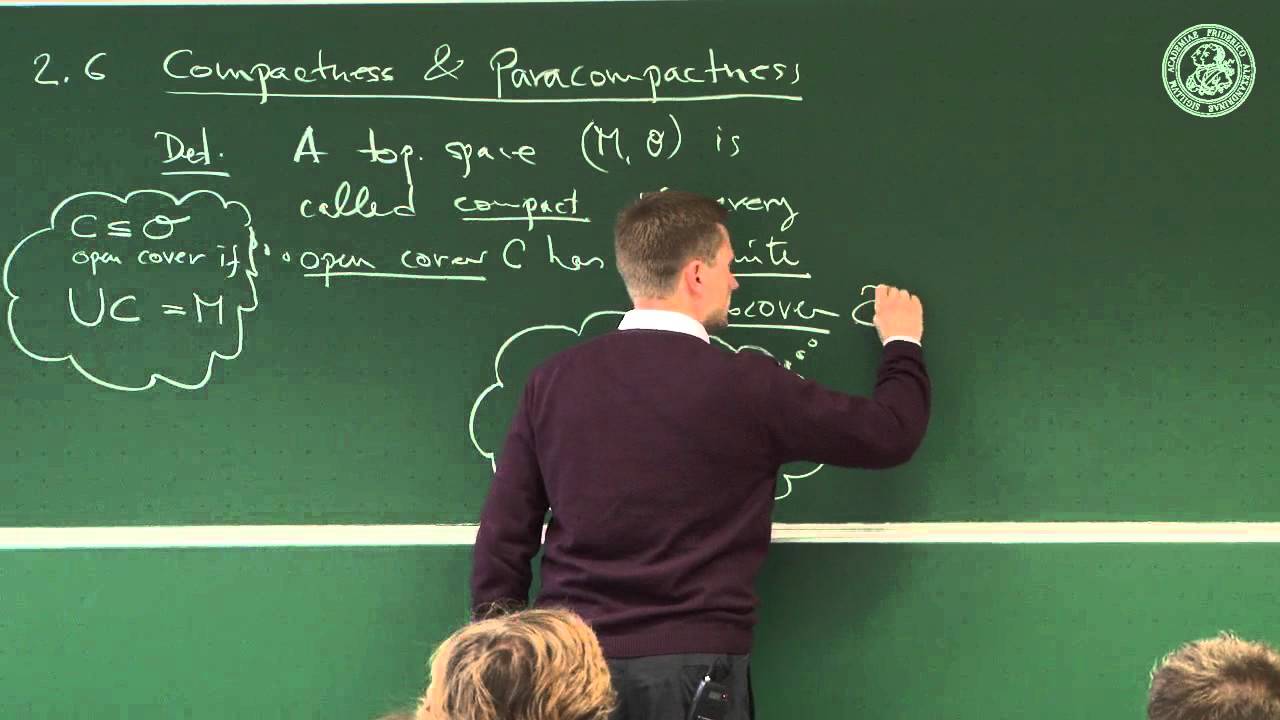
Показать описание
This is from a series of lectures - "Lectures on the Geometric Anatomy of Theoretical Physics" delivered by Dr.Frederic P Schuller
Topological spaces - some heavily used invariants - Lec 05 - Frederic Schuller
Lecture 5-Topological spaces - some heavily used invariants (Frederic Schuller).mp4
Basic Analysis Spaces: Topological Spaces
Topological Spaces Of Topological Space
Functors in Topology
Topological spaces and manifolds | Differential Geometry 24 | NJ Wildberger
Lecture 1.1: Topological Spaces, 'practical' examples
Topological spaces - construction and purpose - Lec 04 - Frederic Schuller
Pirkovskii A. Y. Topological Vector Spaces. 06.10.2023.
Math 145: Continuity in Metric Spaces
Kolchin: Irreducibility = Has Deformations for Sober Topological Spaces
Topological Spaces Part 1
Lecture 0.7: Why topological way of thinking matters? Is it useful? Some examples!!
ActInf MathStream 005.1 ~ Cristian Bodnar 'Topological Deep Learning: Graphs, Complexes, Sheav...
Elyasheev Leibtag: Uniform spaces - part a
Visiting Lecture Topological Space
Topology: Separation axioms Hausdorff space
Algebraic Topology 5: Homeomorphic Spaces have Isomorphic Fundamental Groups
Elements of Point Set Topology by John Baum #shorts
Finite Topologies
msc math (part 1) elements of topology and functional analysis #topology #shortsvideo#shorts#viral
Growth of topological invariants of locally symmetric spaces - Mikolaj Fraczyk
Lecture 9 (Topology)
On metrizable subspaces and quotients of non-Archimedean spaces Cp(X;K)_ Jerzy Kąkol
Комментарии
 1:56:44
1:56:44
 1:56:44
1:56:44
 0:02:19
0:02:19
 0:21:31
0:21:31
 0:32:42
0:32:42
 0:50:14
0:50:14
 0:10:45
0:10:45
 1:38:42
1:38:42
 1:07:53
1:07:53
 0:21:01
0:21:01
 0:12:13
0:12:13
 0:29:12
0:29:12
 0:38:09
0:38:09
 1:05:46
1:05:46
 0:50:04
0:50:04
 2:24:49
2:24:49
 0:22:32
0:22:32
 1:07:59
1:07:59
 0:00:40
0:00:40
 0:14:45
0:14:45
 0:00:31
0:00:31
 0:14:02
0:14:02
 1:52:44
1:52:44
 0:48:04
0:48:04