Functors in Topology

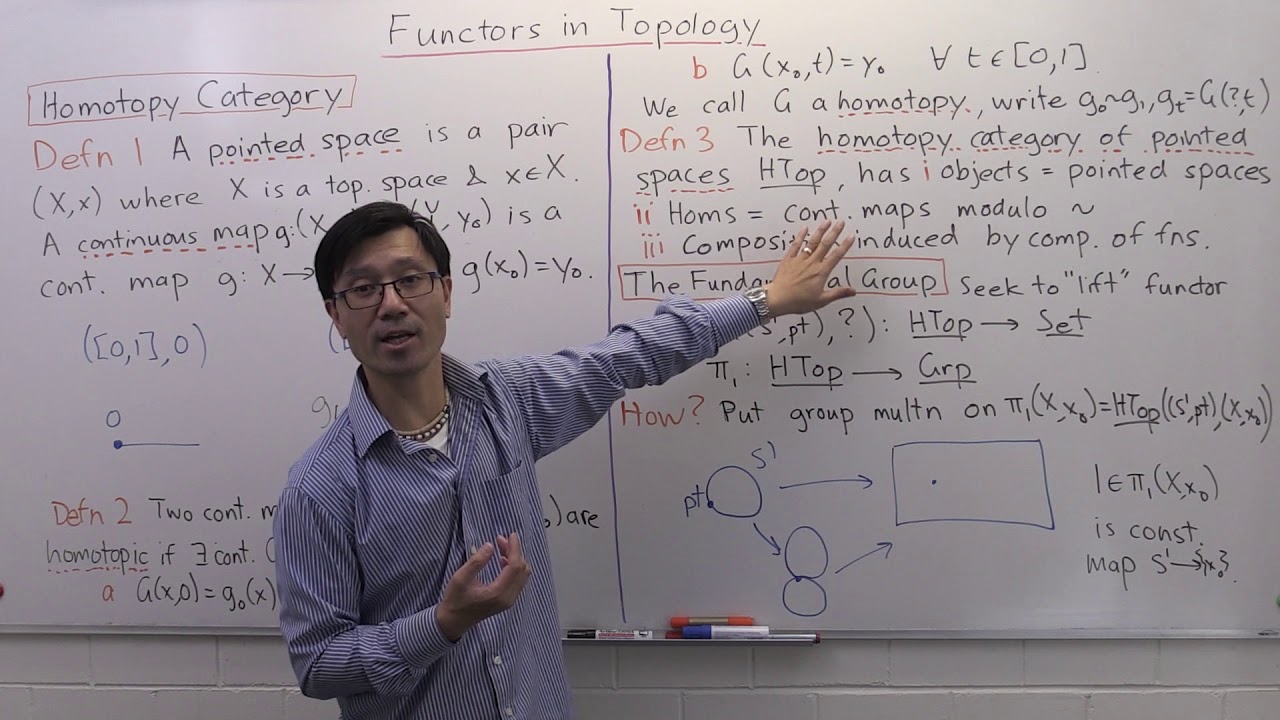
Much of the inspiration for category theory comes from algebraic topology. In this video we look at some functors arising in topology which motivated the development of category theory. We introduce the notion of the homotopy category which features heavily in topology. We define the fundamental group, and see how it gives a functor from the homotopy category to groups. We see how the categorical notion of left invertibility arises naturally in algebraic topology, by using it to prove Brouwer's fixed point theorem. In particular, it illustrates how functors can be used to transfer problems in topology to algebra. We end this video by mentioning some other functors which feature heavily in topology.

[faithfullady]

You are an excellent teacher. Seeing examples clarifies what I read in books.

[tomwatts]

math legend right here, I'm a loyal D.C.M. fan

[asitisj]

When you say twice as fast, do you mean slope or derivative doubles for the trajectory? If yes, could you define the derivative applicable here..

[asitisj]

continuous map from a pointed space, trying to see it

[ziadadwan]

Hello, Make a video about Lie Derivatives please.

[chandankar]

Sir ! I never learned category theory, what are the required prerequisites ?
I have taken analysis and general topology and some abstract algebra, is this enough?