filmov
tv
6. Partial Fraction Decomposition

Показать описание
O Horrible! O Disgusting! (But sometimes useful.) Get the skinny on partial fraction decomposition - everyone's least favorite integration technique - so that you can integrate rational functions when you must.
This is the sixth in what will eventually be a sequence of supplementary videos for a calculus class that I teach based on Chapters 4, 5, and 6 of my book 𝐹𝑢𝑙𝑙 𝐹𝑟𝑜𝑛𝑡𝑎𝑙 𝐶𝑎𝑙𝑐𝑢𝑙𝑢𝑠.
𝐹𝑢𝑙𝑙 𝐹𝑟𝑜𝑛𝑡𝑎𝑙 𝐶𝑎𝑙𝑐𝑢𝑙𝑢𝑠,
𝑇ℎ𝑒 𝐷𝑎𝑟𝑘 𝐴𝑟𝑡 𝑜𝑓 𝐿𝑖𝑛𝑒𝑎𝑟 𝐴𝑙𝑔𝑒𝑏𝑟𝑎,
𝑃𝑟𝑒𝑐𝑎𝑙𝑐𝑢𝑙𝑢𝑠 𝑀𝑎𝑑𝑒 𝐷𝑖𝑓𝑓𝑖𝑐𝑢𝑙𝑡,
𝐿𝑜𝑏𝑎𝑐ℎ𝑒𝑣𝑠𝑘𝑖 𝐼𝑙𝑙𝑢𝑚𝑖𝑛𝑎𝑡𝑒𝑑.
The first three are available for sale as paperbacks at Amazon, and as pdfs at Lulu. (The Lobachevski book is available at Amazon and the American Mathematical Society)
0:00 Intro (to bottom-heavy rational functions)
4:05 Rational = Polynomial + BH-rational
5:20 polynomial division
9:34 the FULL integral of 1/x
16:06 ∫(constant)/(linear)dx
20:03 a little algebraic trick
21:55 Partial Fractions (Case 1: distinct linear factors)
32:25 Another example
39:42 repeated linear factors
45:15 irreducible quadratic factors
This is the sixth in what will eventually be a sequence of supplementary videos for a calculus class that I teach based on Chapters 4, 5, and 6 of my book 𝐹𝑢𝑙𝑙 𝐹𝑟𝑜𝑛𝑡𝑎𝑙 𝐶𝑎𝑙𝑐𝑢𝑙𝑢𝑠.
𝐹𝑢𝑙𝑙 𝐹𝑟𝑜𝑛𝑡𝑎𝑙 𝐶𝑎𝑙𝑐𝑢𝑙𝑢𝑠,
𝑇ℎ𝑒 𝐷𝑎𝑟𝑘 𝐴𝑟𝑡 𝑜𝑓 𝐿𝑖𝑛𝑒𝑎𝑟 𝐴𝑙𝑔𝑒𝑏𝑟𝑎,
𝑃𝑟𝑒𝑐𝑎𝑙𝑐𝑢𝑙𝑢𝑠 𝑀𝑎𝑑𝑒 𝐷𝑖𝑓𝑓𝑖𝑐𝑢𝑙𝑡,
𝐿𝑜𝑏𝑎𝑐ℎ𝑒𝑣𝑠𝑘𝑖 𝐼𝑙𝑙𝑢𝑚𝑖𝑛𝑎𝑡𝑒𝑑.
The first three are available for sale as paperbacks at Amazon, and as pdfs at Lulu. (The Lobachevski book is available at Amazon and the American Mathematical Society)
0:00 Intro (to bottom-heavy rational functions)
4:05 Rational = Polynomial + BH-rational
5:20 polynomial division
9:34 the FULL integral of 1/x
16:06 ∫(constant)/(linear)dx
20:03 a little algebraic trick
21:55 Partial Fractions (Case 1: distinct linear factors)
32:25 Another example
39:42 repeated linear factors
45:15 irreducible quadratic factors
Partial Fraction Decomposion
Ex 6: Partial Fraction Decomposition (Repeating Quadratic Factors)
Episode 6 | Partial Fraction Decomposition contains two Linear Factors in the Denominator | Tutorial
how to setup partial fractions (all cases)
Partial Fraction Decomposition - Example 6
Partial Fraction Decomposition ( 6 Worked Examples)
Partial Fraction Decomposition All Types
6 Steps of Partial Fraction Decomposition (Partial Fraction Expansion) Method |Math Dot Com
Thermodynamics II (Applications of Thermodynamics in 8 hours and 34 minutes)
Integration By Partial Fractions
Integration Techniques Video 6 - Partial Fraction Decomposition Examples
Partial Fractions
Partial Fraction Decomposition: Proof of the Simplest Case
7.3.6 Partial Fraction Decomposition
Partial Fraction #formulas #partialfractions #shorts
Partial Fractions
the cover-up method & why it works! (for partial fractions decomposition)
Find the Partial Fraction Decomposition - Step-By-Step Technique
Partial Fraction Decomposition
Partial Fraction Decomposition (Convenient Values): Ex 6
Partial Fraction Decomposition | How to solve Partial Fraction | Example 6
Find the Partial Fraction Decomposition - Super Easy Way
The Cover-up method of Partial fraction decomposition
(Partial Fraction Decomposition) (x+2)/(x^2+5x+6)
Комментарии
 0:14:12
0:14:12
 0:08:17
0:08:17
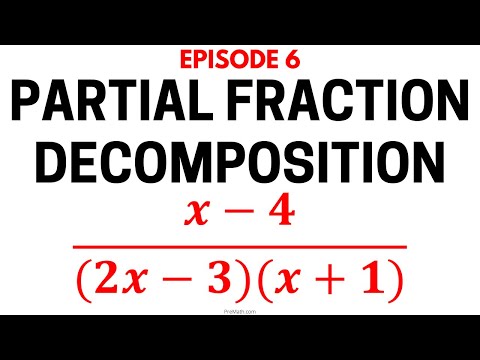 0:08:06
0:08:06
 0:09:08
0:09:08
 0:08:06
0:08:06
 0:44:29
0:44:29
 0:32:24
0:32:24
 0:09:24
0:09:24
 8:34:22
8:34:22
 0:41:07
0:41:07
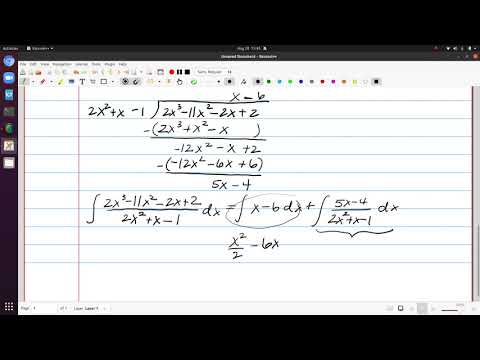 0:17:50
0:17:50
 0:00:05
0:00:05
 0:20:05
0:20:05
 0:11:38
0:11:38
 0:00:08
0:00:08
 0:00:45
0:00:45
 0:08:37
0:08:37
 0:08:04
0:08:04
 0:06:27
0:06:27
 0:11:04
0:11:04
 0:12:54
0:12:54
 0:08:08
0:08:08
 0:12:36
0:12:36
 0:05:56
0:05:56