Mastering Exponential Equations: Same Base Solutions | Logarithmic Functions

Dive into the world of exponential equations with the same base and learn how to solve them using logarithmic functions. In this comprehensive tutorial, we'll explore step-by-step techniques to tackle equations with identical bases, providing clarity and insights along the way. Perfect for students and enthusiasts seeking to enhance their understanding of exponential functions and logarithmic solutions.

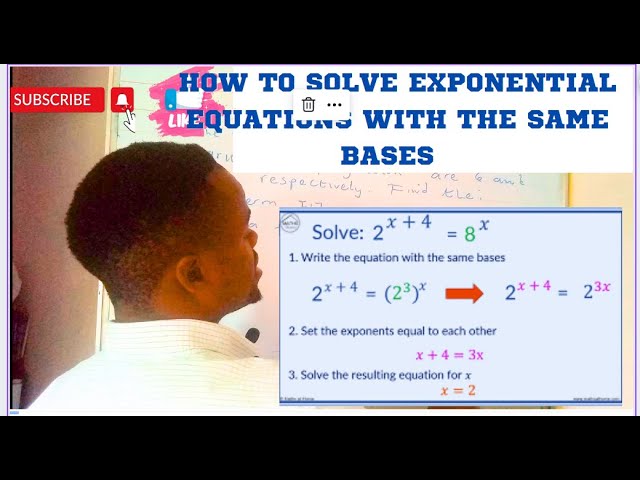
To work out exponential equations with the same bases, follow these steps:Identify the Bases: Ensure that both sides of the equation have the same base. If not, rewrite them with the same base.Apply the Exponent Rule: Since the bases are the same, equate the exponents. For example, if you have ( a^x = a^y ), then ( x = y ).Solve for the Unknown: After equating the exponents, solve for the unknown variable. This can involve basic algebraic manipulation.Let's go through an example:Example: Solve for ( x ) in the equation ( 2^x = 2^5 ).Solution:Since the bases are the same (both 2), we equate the exponents: ( x = 5 ).Therefore, the solution is ( x = 5 ).Remember, the key is to make sure the bases are the same, then equate the exponents and solve for the unknown. Practice with various examples to reinforce your understanding.#ExponentialEquations #LogarithmicFunctions #SameBaseEquations #MathematicsTutorial #ExponentialFunctions #MathHelp