filmov
tv
The Most Remarkable Theorem: Part II (Surface Curvature)

Показать описание
Theorema Egregium Part II: Surface curvature.
#artofproblemsolving #gauss #calculus #algebra #calculus3 #vectorcalculus
#theoremaegregium #gaussiancurvature #curvature #extrinsicgeometry #intrinsicgeometry
#surfaces #curves #tangentvectors #normalvectors #movingframe #frenetserretframe
#parametrizedcurves #parametriccurves #parametricsurfaces #vectors #vector #mathematics
#youareawesome
#artofproblemsolving #gauss #calculus #algebra #calculus3 #vectorcalculus
#theoremaegregium #gaussiancurvature #curvature #extrinsicgeometry #intrinsicgeometry
#surfaces #curves #tangentvectors #normalvectors #movingframe #frenetserretframe
#parametrizedcurves #parametriccurves #parametricsurfaces #vectors #vector #mathematics
#youareawesome
The paradox at the heart of mathematics: Gödel's Incompleteness Theorem - Marcus du Sautoy
The Big Theorem, Part I
Why did they prove this amazing theorem in 200 different ways? Quadratic Reciprocity MASTERCLASS
The most beautiful idea in physics - Noether's Theorem
Bell's Inequality: The weirdest theorem in the world | Nobel Prize 2022
Only U.S. President to prove a theorem
Will.i.am has an incredible theory on #ElonMusk buying #Twitter 🧐🧐🧐 #FreshPair bonus clip #shorts...
This theory of ninja turtles is amazing 🫠🐢
Noether’s theorem
Your Daily Equation #25: Noether's Amazing Theorem: Symmetry and Conservation
Imu, Gorosei Destroys Lulusia Island #onepiece #shorts
Lecture 3: Cantor's Remarkable Theorem and the Rationals' Lack of the Least Upper Bound Pr...
Exploring Incredible Einstein's Infinite Multiverse Theory | Joe Rogan
Bayes theorem, the geometry of changing beliefs
Euler's Equation: 'The Most Beautiful Theorem in Mathematics' - Professor Robin Wilso...
Gumball Just Broke Cartoon Network on TV
We Made It This Far
Plot Twist: There’s No Dark Matter. Our Theory of Gravity is Broken
The Big Theorem of Differential Equations: Existence & Uniqueness
Pick's theorem: The wrong, amazing proof
Existence & Uniqueness Theorem, Ex1
Your Daily Equation #21: Bell's Theorem and the Non-locality of the Universe
Helene Lithium Conspiracy
Metaphysical Implications Of Godel's Incompleteness Theorem - Part 1
Комментарии
 0:05:20
0:05:20
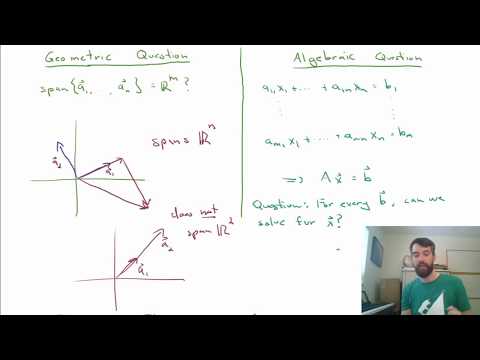 0:14:01
0:14:01
 0:56:37
0:56:37
 0:09:53
0:09:53
 0:13:22
0:13:22
 0:01:00
0:01:00
 0:00:52
0:00:52
 0:00:31
0:00:31
 0:01:01
0:01:01
 0:38:08
0:38:08
 0:00:13
0:00:13
 1:18:40
1:18:40
 0:00:42
0:00:42
 0:15:11
0:15:11
 1:00:42
1:00:42
 0:02:31
0:02:31
 0:01:39
0:01:39
 0:10:20
0:10:20
 0:12:22
0:12:22
 0:06:02
0:06:02
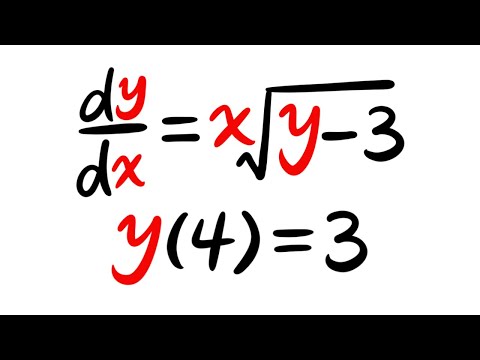 0:11:22
0:11:22
 0:50:34
0:50:34
 0:01:00
0:01:00
 2:02:45
2:02:45