filmov
tv
Trigonometry | Prove Trigonometry Identities | Trig Identities #shorts
Показать описание
Prove Trigonometric Identities
In this video, we will revise the prove of Trigonometric Identities.
We will prove the following Trig Identities
cos^2 A + sin^2 A = 1
1 + tan^2 A = sec^2 A
cot^2 A + 1 = cosec^2A
Hint 💡
Identity :
An equation is called an identity when it is true for all values of the variables involved
Trigonometric Identity :
Similarly, an equation involving trigonometric ratios of an angle is called a trigonometric identity, if it is true for all values of the angle(s) involved
Trigonometric Identities in class 10th NCERT:
cos^2 A + sin^2 A = 1
1 + tan^2 A = sec^2 A
cot^2 A + 1 = cosec^2A
We will prove the above trigonometric identities via a Δ ABC, right-angled at B.
Understand
Above equations hold true for all A such that 0° ≤ A ≤ 90°. So, they are trigonometric identities
Note📝
The above definitions and identities are taken from NCERT book ch-8 Trigonometry
To watch full video👇🏻
#maths #trigonometry #shorts
In this video, we will revise the prove of Trigonometric Identities.
We will prove the following Trig Identities
cos^2 A + sin^2 A = 1
1 + tan^2 A = sec^2 A
cot^2 A + 1 = cosec^2A
Hint 💡
Identity :
An equation is called an identity when it is true for all values of the variables involved
Trigonometric Identity :
Similarly, an equation involving trigonometric ratios of an angle is called a trigonometric identity, if it is true for all values of the angle(s) involved
Trigonometric Identities in class 10th NCERT:
cos^2 A + sin^2 A = 1
1 + tan^2 A = sec^2 A
cot^2 A + 1 = cosec^2A
We will prove the above trigonometric identities via a Δ ABC, right-angled at B.
Understand
Above equations hold true for all A such that 0° ≤ A ≤ 90°. So, they are trigonometric identities
Note📝
The above definitions and identities are taken from NCERT book ch-8 Trigonometry
To watch full video👇🏻
#maths #trigonometry #shorts
How to Prove Trigonometric Identities (and how not to)
Learn How To Prove A Trigonometry Question & Apply Trig Identities Effectively
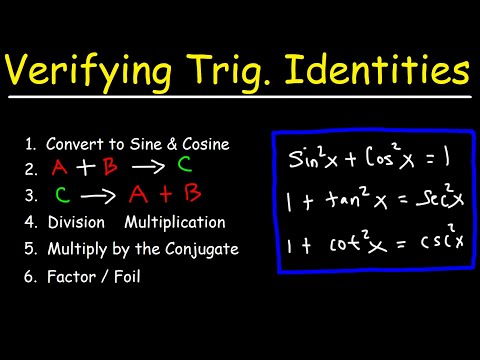
Verifying Trigonometric Identities
The Best Way to Master Trigonometric Identities
Verifying Trigonometric Identities Easily - Strategy Explained (14 Examples)
How to Prove Trigonometric Identities (Precalculus - Trigonometry 24)
Trig Identities
Prove Trigonometric Identities Getting Started MCR3U
Class 10 Trigonometry Identity Prove Easily | SEE Maths Ep - 13
Trigonometry - Proving trig identities
Verifying a trigonometric Identities
Proving Trigonometric Identities Grade 12
Mathematics Trigonometric Proofs: Solving Trigonometric Identities Part 1
How to solve trigonometry identities! (ultimate practice)
Trigonometric Identities
Verifying Trigonometric Identities
Trig Visualized: One Diagram to Rule them All (six trig functions in one diagram)
Intro to Trigonometric Identities - part 1
Prove this Trigonometric Identity | Step-by-Step Explanation
Verifying trigonometric identities, hard with multiple steps
Proving some Random Trigonometric Identities
Trigonometry Grade 12: Identity Exam
Solving Trigonometric Identity EXAMPLE | Pre-Calculus
2 Useful Tips to Help You Prove Trig Identities
Комментарии
 0:07:37
0:07:37
 0:13:17
0:13:17
 0:24:51
0:24:51
 0:03:36
0:03:36
 0:25:08
0:25:08
 0:55:39
0:55:39
 0:27:57
0:27:57
 0:07:57
0:07:57
 0:10:34
0:10:34
 0:10:55
0:10:55
 0:05:12
0:05:12
 0:05:19
0:05:19
 0:11:45
0:11:45
 1:27:10
1:27:10
 0:11:20
0:11:20
 0:09:14
0:09:14
 0:04:15
0:04:15
 0:14:36
0:14:36
 0:07:23
0:07:23
 0:06:53
0:06:53
 0:08:12
0:08:12
 0:04:06
0:04:06
 0:00:55
0:00:55
 0:10:00
0:10:00