Fourier Series - Partial Differential Equation | Lecture 13

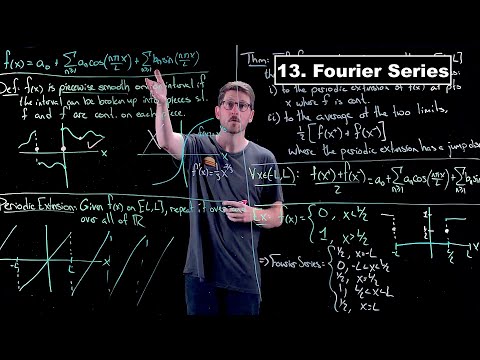
While performing separation of variables we have encountered numerous series solutions involving sine and cosine functions. Such series are called Fourier series and in this lecture we discuss their properties and whether they converge. In particular, we discuss periodic extensions of functions defined on a finite interval, as well as define the class of piecewise smooth functions. We then state the Fourier convergence theorem and examine its consequences with an example.

Follow @jbramburger7 on Twitter for updates.

[omgaddad]

DANG how do you write backwards that's a skill 😆

[FaTeAnimationVault]

ℏ = h / (2π)
Zeros are just naughty.