Solve Trigonometric Equations With Sine | √2·sin(3x) - 4 = -3 | Part 2

Follow us:

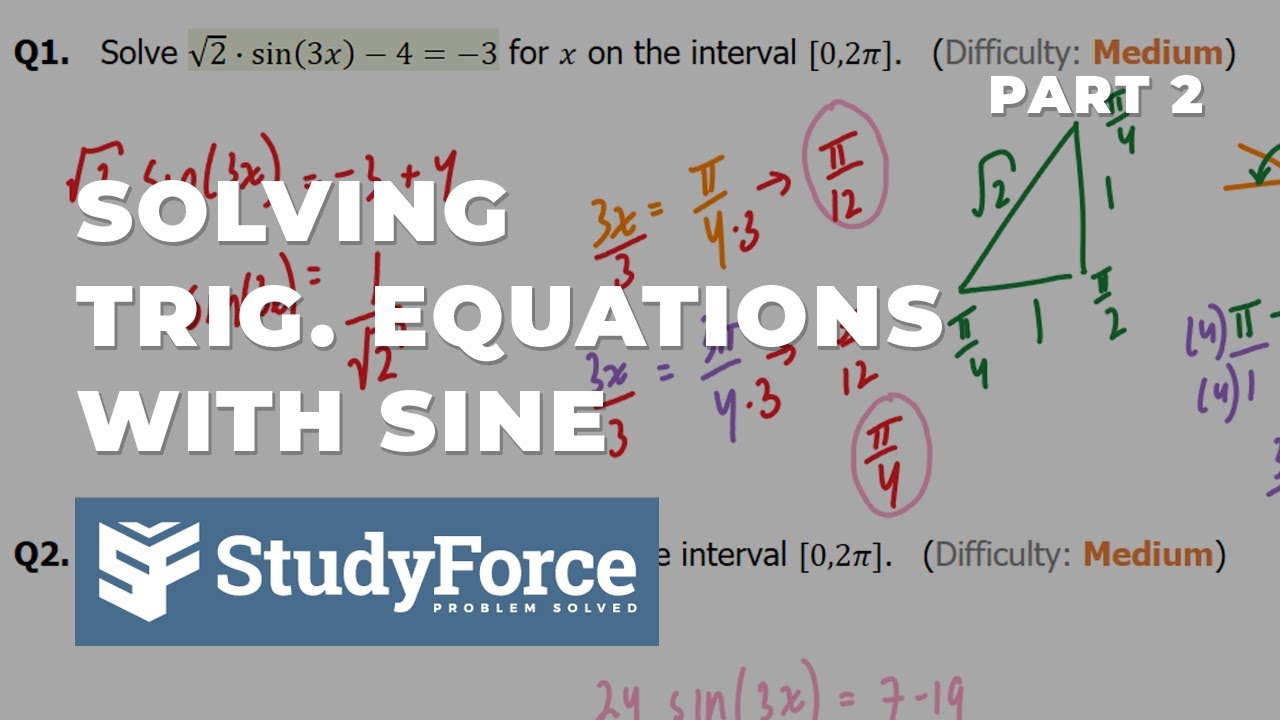
Q1. Solve √2∙sin⁡〖(3x)−4=−3〗 for x on the interval [0,2π]. (Difficulty: Medium)

Q2. Solve 24∙sin⁡〖(3x)+19=7〗 for x on the interval [0,2π]. (Difficulty: Medium)

*******************

To solve an equation containing a single trigonometric function:

1. Isolate the function on one side of the equation.
2. Solve for the variable.

*******************

A trigonometric equation is an equation that contains a trigonometric expression with a variable, such as sin x.

The values that satisfy such an equation are its solutions. (There are trigonometric equations that have no solution.)

When an equation includes multiple angles, the period of the function plays an important role in ensuring that we do not leave out any solutions.