filmov
tv
A very interesting factorial based sum

Показать описание
Full solution development for this cool series problem using a few special functions we've become accustomed to here on the channel.
My complex analysis lectures:
Support my work via Patreon:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
My complex analysis lectures:
Support my work via Patreon:
You can follow me on Instagram for write ups that come in handy for my videos and DM me in case you need math help:
My LinkedIn:
Advanced MathWear:
A very interesting factorial based sum
Very Interesting Math Factorial Problem l find x! l Tricky Solution.
The WEIRD thing about 432 Factorial... #shorts
Fun Factorial Fact
Big Factorials - Numberphile
Can you solve this tricky factorial problem?
A very interesting factorial question for GATE & IITJEE catch the point | concept library
Fun Factorial Problem
MIND-BLOWING Factorial Equation | An Easy Way to Solve it
Interesting factorial division. Very simple to solve
A very tricky math question with factorial
Explore the Amazing Factorial Table Chart!
Factorial (!) ? | Fun Math | Don't Memorise
Factorial Challenge
an exciting factorial number puzzle.
An Amazing Factorial Equation Challenge | Can You Solve This?
A very interesting question on Factorial #cbsemaths #maths #MathsOlympiad #mathsimplification
find factorial in c++|c++ tutorials for beginners #coding #shortvideo #basicofcoding #reels #viral
Amazing Factorial #shorts #youtubeshorts
base factorial -- the most exciting number base
Ronaldo÷Messi=??Amazing Factorial #shorts #youtubeshorts
factorial !🔥|#mathematics #calculus #maths #ytshorts #yt #cuet #cbse #shorts #viral #fun
Sub - factorial Question | Amazing Concept Of factorials |
Factorial Experiment | Interesting Factorial Problem By Sooraj Kerketta Sir
Комментарии
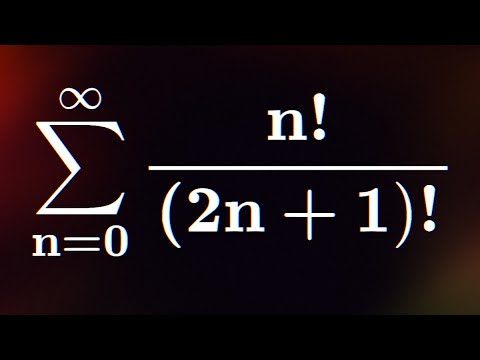 0:08:31
0:08:31
 0:08:03
0:08:03
 0:00:56
0:00:56
 0:00:57
0:00:57
 0:12:27
0:12:27
 0:11:02
0:11:02
 0:01:55
0:01:55
 0:01:00
0:01:00
 0:09:44
0:09:44
 0:01:50
0:01:50
 0:10:18
0:10:18
 0:00:06
0:00:06
 0:00:45
0:00:45
 0:01:00
0:01:00
 0:18:10
0:18:10
 0:15:55
0:15:55
 0:03:39
0:03:39
 0:00:20
0:00:20
 0:00:30
0:00:30
 0:19:48
0:19:48
 0:00:25
0:00:25
 0:00:12
0:00:12
 0:03:24
0:03:24
 0:00:17
0:00:17