filmov
tv
Solving a Cool Diophantine Equation: a^2+a+34=b^2
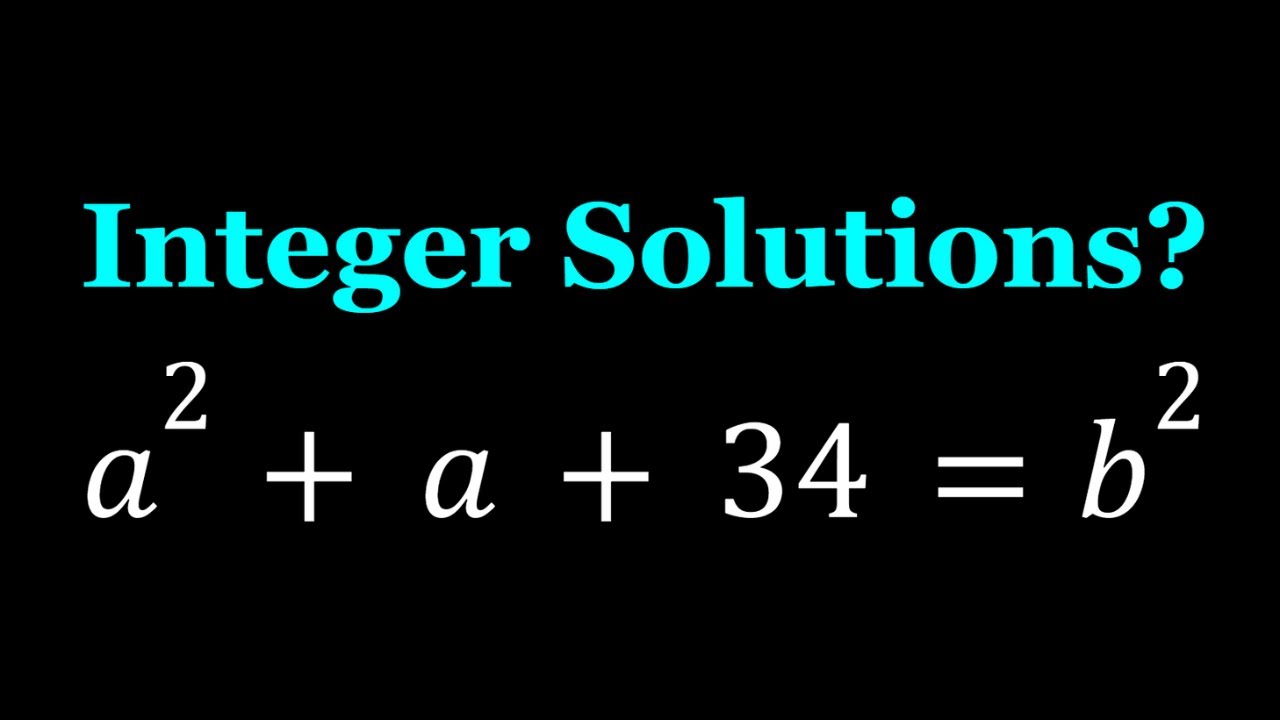
Показать описание
If you need to post a picture of your solution or idea:
#NumberTheoryProblems #DiophantineEquations
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
#NumberTheoryProblems #DiophantineEquations
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
Solving A Cool Diophantine Equation With Integers
Diophantine Equation: ax+by=gcd(a,b) ← Number Theory
Solving a Cool Diophantine Equation: a^2+a+34=b^2
A Nice Diophantine Equation in Number Theory | You Should Learn This Theorem | Math Olympiad
A Cool Diophantine Equation
Diophantine Equations: Strategies and Examples
Solve Diophantine Equations by Factoring
Let's Solve A Cool Diophantine Equation
Number Theory | Linear Diophantine Equations
Solving a Cool Diophantine Problem | Solve For Integer Values
Solving A Set Of Diophantine Equation In Under A Minute
Solving a Quadratic Diophantine Equation
A Linear Diophantine Equation
Cool Algebraic Tricks! | How to Solve the Quadratic Diophantine Equation?
A USAMO Problem: Solve Diophantine Equation (Easier Than You Think)
A Great Book on Diophantine Equations
Cool Trick for Solving the Diophantine Equation (x+y)^2=x^3+y^3 | Integer Solution | Math Olympiad
Solving a Diophantine Equation by Factoring | Solve for Integer Solutions #NumberTheory
Solving A Diophantine Equation with Prime Numbers
Solve Diophantine Equation by Factoring
2023 Number Challenge: Solve the Diophantine Equation, leave your answer in the comments
A Diophantine Equation with Integer Solutions
How To Solve Linear Diophantine Equations
A Cool Diophantine Equation
Комментарии
 0:10:57
0:10:57
 0:09:43
0:09:43
 0:05:17
0:05:17
 0:07:40
0:07:40
 0:04:36
0:04:36
 0:27:34
0:27:34
 0:01:58
0:01:58
 0:07:34
0:07:34
 0:09:17
0:09:17
 0:03:23
0:03:23
 0:00:58
0:00:58
 0:11:04
0:11:04
 0:00:27
0:00:27
 0:03:29
0:03:29
 0:00:59
0:00:59
 0:00:18
0:00:18
 0:03:51
0:03:51
 0:07:08
0:07:08
 0:00:54
0:00:54
 0:00:35
0:00:35
 0:00:56
0:00:56
 0:00:49
0:00:49
 0:10:24
0:10:24
 0:07:09
0:07:09