filmov
tv
Undetermined Coefficients in the First ODE Course

Показать описание
To find a particular solution of the ODE y" + 2 y' + y = x e–x by the method of undetermined coefficients, the characteristic roots m=–1, –1, must be determined; the latent roots m' –1, –1, ± 2 i, must also be extracted from the inhomogeneous term. (What I call "latent roots" are the characteristic roots that determine the complete general solution of which the inhomogeneous term is but a fragment.) The template form of the particular solution must then take into account the totality of the repeated –1, so that the template for the particular solution is not just e–x (a + b x), but yp + e–x(a + b x)x2. This seems to require a form of "backward thinking" that I have found difficult to inspire in my students, no matter what devices I've tried in the past.
In this video I'll detail some of the strategies I've used, strategies that did not seem to be successful in the short time the first ODE course made available for this topic. Then, I'll take a look at some Maple tools that both find the appropriate template form, and give insight into why this "backward thinking" is theoretically sound (and appropriate).
For more information, visit us at:
Explore more Maple videos on this playlist:
Follow Maplesoft:
In this video I'll detail some of the strategies I've used, strategies that did not seem to be successful in the short time the first ODE course made available for this topic. Then, I'll take a look at some Maple tools that both find the appropriate template form, and give insight into why this "backward thinking" is theoretically sound (and appropriate).
For more information, visit us at:
Explore more Maple videos on this playlist:
Follow Maplesoft:
 0:12:44
0:12:44
 0:41:28
0:41:28
 0:13:50
0:13:50
 0:18:43
0:18:43
 0:36:48
0:36:48
 0:12:04
0:12:04
 0:09:19
0:09:19
 0:16:32
0:16:32
 0:07:14
0:07:14
 0:06:01
0:06:01
 0:22:14
0:22:14
 0:16:42
0:16:42
 0:13:48
0:13:48
 0:15:49
0:15:49
 0:05:26
0:05:26
 0:51:35
0:51:35
 0:10:54
0:10:54
 0:15:51
0:15:51
 0:15:39
0:15:39
 0:15:18
0:15:18
 0:26:00
0:26:00
 0:07:15
0:07:15
 0:36:08
0:36:08
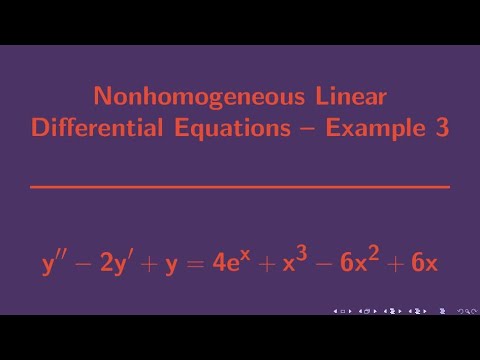 0:11:45
0:11:45