filmov
tv
Given the vertex and focus learn to write the equation of the parabola in standard form

Показать описание
Learn how to write the equation of a parabola given the vertex and the focus. A parabola is the shape of the graph of a quadratic equation. A parabola can open up or down (if x is squared) or open left or right (if y is squared). Recall that the focus and the vertex of a parabola are on the same line of symmetry.
When given the focus and the vertex of a parabola, recall that the focus of a parabola is inside the parabola. This enables us to identify the direction which the required parabola opens. We also need to identify the value of p, which is the distance between the vertex and the focus. p is negative when the parabola opens down or left and is positive when the parabola opens right or up.
Once we identify the direction and the value of p, we can use the equation of parabola given by (y - k)^2 = 4p(x - h) for parabolas that opens up or down and (x - h)^2 = 4p(y - k) for parabolas that opens left or right
#conicsections #parabolaconicsections
When given the focus and the vertex of a parabola, recall that the focus of a parabola is inside the parabola. This enables us to identify the direction which the required parabola opens. We also need to identify the value of p, which is the distance between the vertex and the focus. p is negative when the parabola opens down or left and is positive when the parabola opens right or up.
Once we identify the direction and the value of p, we can use the equation of parabola given by (y - k)^2 = 4p(x - h) for parabolas that opens up or down and (x - h)^2 = 4p(y - k) for parabolas that opens left or right
#conicsections #parabolaconicsections
Given the vertex and focus learn to write the equation of the parabola in standard form
Finding the standard form of a parabola given vertex and focus
How to find the equation of a parabola given vertex and focus | @ProfD
Determine the equation of parabola given the vertex and focus
Finding Equation of Parabola Given Focus and Vertex
FINDING EQUATION OF PARABOLA WITH GIVEN VERTEX AND FOCUS
Given the vertex and focus determine the standard form of the parabola
Vertex form of Parabola from Vertex and Focus
When given the vertex and focus graph the parabola conic sections
Parabola Find Vertex, Focus, Directrix, and Graph
How to Find the Equation of a Parabola Given a Vertex and Focus
Conic Sections, Parabola, Shifted : Find Equation Given Vertex and Focus
Identify the vertex, focus and directrix of a parabola
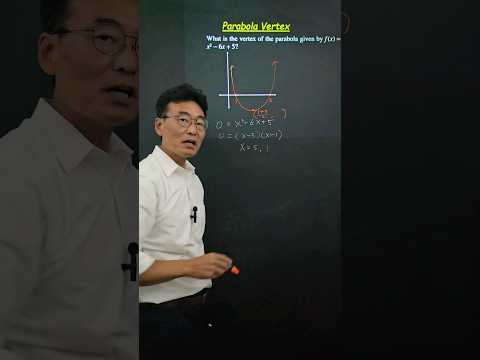
Vertex of Parabola Using X-Intercepts
Finding Equation of Parabola Given Vertex and Directrix
Write the equation of an ellipse given the center, vertex and focus
DETERMINE THE PARTS OF A PARABOLA GIVEN VERTEX AND FOCUS : Easy method to graph a parabola
Writing an equation of a parabola given the vertex and the focus
Writing the Equation of an Ellipse Given the Center, Vertex, and Focus
Write the equation of an ellipse given the center vertex and focus
Find the equation vertex (5, 2) Focus (3, 2)
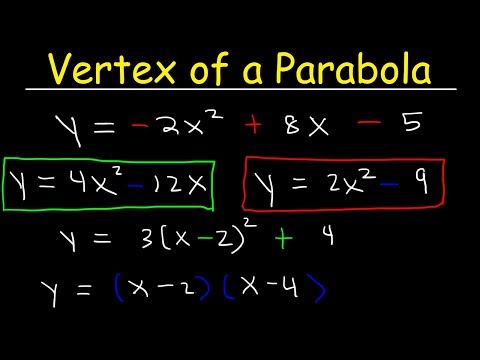
How To Find The Vertex of a Parabola - Standard Form, Factored & Vertex Form
Conic Sections: Find Equation of Parabola Given Vertex and Focus (Opens Up)
Writing the equation of a parabola given the vertex and focus
Комментарии
 0:04:51
0:04:51
 0:05:26
0:05:26
 0:03:46
0:03:46
 0:03:51
0:03:51
 0:02:23
0:02:23
 0:05:27
0:05:27
 0:05:01
0:05:01
 0:06:42
0:06:42
 0:01:36
0:01:36
 0:08:33
0:08:33
 0:02:52
0:02:52
 0:02:56
0:02:56
 0:01:34
0:01:34
 0:01:01
0:01:01
 0:02:00
0:02:00
 0:03:36
0:03:36
 0:05:55
0:05:55
 0:03:44
0:03:44
 0:04:46
0:04:46
 0:03:45
0:03:45
 0:04:25
0:04:25
 0:18:03
0:18:03
 0:06:20
0:06:20
 0:03:01
0:03:01