filmov
tv
A Breakthrough in Higher Dimensional Spheres | Infinite Series | PBS Digital Studios
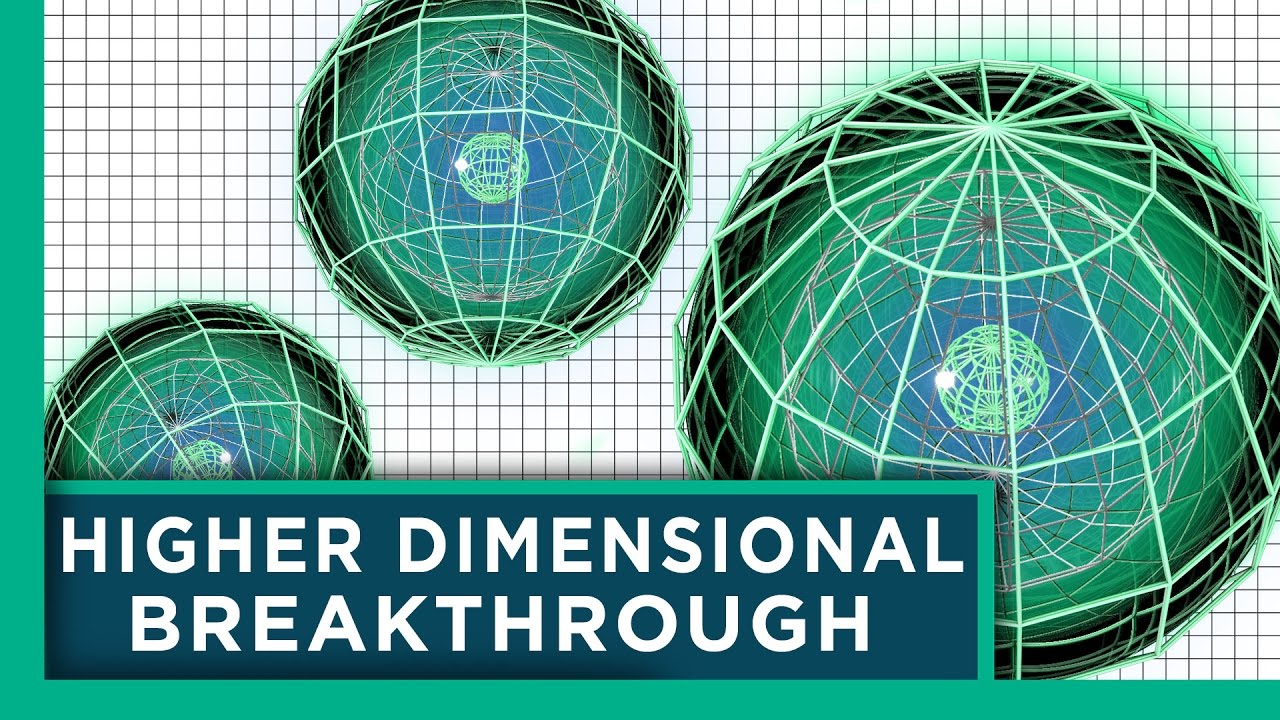
Показать описание
How do you stack hundred-dimensional oranges? Learn about recent breakthroughs in our understanding of hyperspheres in the first episode of Infinite Series, a show that tackles the mysteries and the joy of mathematics. From Logic to Calculus, from Probability to Projective Geometry, Infinite Series both entertains and challenges its viewers to take their math game to the next level.
Higher dimensional spheres, or hyperspheres, are counter-intuitive and almost impossible to visualize. Mathematician Kelsey Houston-Edwards explains higher dimensional spheres and how recent revelations in sphere packing have exposed truths about 8 and 24 dimensions that we don't even understand in 4 dimensions.
Tweet at us! @pbsinfinite
Email us! pbsinfiniteseries [at] gmail [dot] com
Sphere Packing in Higher Dimensions - Quanta Magazine
Why You Should Care about High-Dimensional Sphere Packing - Scientific American
Written and Hosted by Kelsey Houston-Edwards
Produced by Rusty Ward
Graphics by Ray Lux
Комментарии
 0:07:52
0:07:52
 0:27:07
0:27:07
 0:22:09
0:22:09
 0:27:52
0:27:52
 0:03:17
0:03:17
 0:15:46
0:15:46
 0:32:11
0:32:11
 0:01:22
0:01:22
 0:07:20
0:07:20
 8:46:05
8:46:05
 0:02:14
0:02:14
 0:03:01
0:03:01
 0:09:20
0:09:20
 0:26:42
0:26:42
 0:27:27
0:27:27
 0:09:11
0:09:11
 0:15:48
0:15:48
 0:11:25
0:11:25
 0:07:30
0:07:30
 0:37:37
0:37:37
 0:02:59
0:02:59
 0:10:31
0:10:31
 0:02:49
0:02:49
 0:00:51
0:00:51