Solving Polynomial Equations 1: A power series approach | Research Level Mathematics | Wild Egg Math

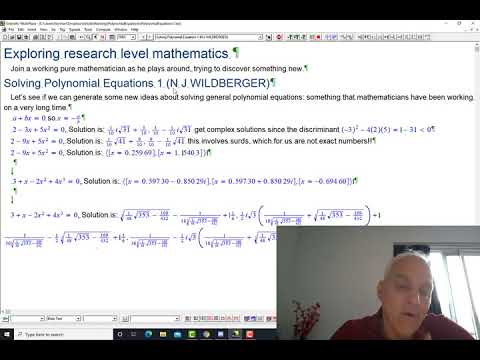
How do you solve a polynomial equation? Let's play around with this famous problem and see if we can tinker our way to some new insights. Along the way we strengthen our understanding of polynomial or polynumber arithmetic, and its extension to on-polynumbers or power series, and get some experience with the power of Scientific Workplace for writing and working on mathematics.

This is the first video in a series where we will reconfigure the landscape for the classical algebraic problem of solving a polynomial equation. Formulas involving so-called "radicals" --- which themselves cannot be exactly evaluated --- are finessed, and we move the subject in an entirely different direction, by harnessing the utility of formal power series and rational extensions of them.

The entire series will become available to Members of the Wild Egg Maths channel over the next few months -- be sure to click the JOIN button to participate in an exciting adventure to EXPLORE RESEARCH LEVEL MATHS. This is a unique opportunity to be guided into the fascinating world of mathematical research by one of the world's leading mathematical educators.

************************

Here are the Wild Egg Maths Playlists (some available only to Members!)

************************************************
Here are the Insights into Mathematics Playlists:

****************

[seyedsmousavi]

Very interesting and insightful. I really appreciate your efforts to reformulate the entire modern mathematics in a computable way. I hope people realise at some point they are no gods to play with infinities that arise when assuming one can understand something completely by just using itself and not anything more! Infinities are relative and show our theoretical weaknesses, so why pretending to be those who we are not!

[JGLambourne]

As an engineer what I really want is a numerical solution where I can specify the required precision and a budget in terms of computation and memory usage. If the problem cannot be solved to the required precision without going over budget then I want details of the best approximation found and the likely error. I worry that truncating the polynomial in t at some arbitrary term doesn't obviously give me an error bound for the solution. If we want to avoid real numbers then we need some kind of adaptive precision where errors are known at each stage.

[Stelu]

Hi Professor Wildberger,

Amazing series (as always! Your stuff is just so great!)

Can I just ask a quick etiquette question - I imagine there are people following along who might not be using Scientific Workplace (I have been writing things up using a Python/Sage environment). So it ok to share code of how we implement this with in different environments, and would the comments section be the place to do it?

[thomasfuhrmann]

Can we also set t=1 to simplify things since c_0!=0?

[brianaicheler]

I have joined this channel and pay for membership but can no longer access the videos since changing my PC. Has anyone any idea how to regain access?

[hayshussey]

I have a insight to a math paradox tha I would like to publish can you help me publish.

[jaanuskiipli]

Sorry for being so dumb, but if we try to solve a polynomial in t to be 0 then one way is yes as done here to set all the coefficients to be 0, but could there be other solutions?

[jasonc]

In other words, perturbation theory. It's how nonlinear problems in physics are actually solved.