filmov
tv
Suppose \( a, b, c \) are in A.P. Let \( A, G \) be the arithmetic mean and geometric mean betwe...

Показать описание
Suppose \( a, b, c \) are in A.P. Let \( A, G \) be the arithmetic mean and geometric mean between \( a \) and \( b, A^{\prime}, G^{\prime} \) be the arithmetic mean and geometric mean between \( b \) and \( c \), then
(a) \( A^{2}+G^{2}=A^{\prime 2}+G^{\prime 2} \)
(b) \( A^{2}-G^{2}=A^{2}-G^{2} \)
(c) \( A^{2}-A^{2}=G^{2}-G^{2} \)
(d) \( A^{2}+A^{2}=G^{2}+G^{2} \)
(a) \( A^{2}+G^{2}=A^{\prime 2}+G^{\prime 2} \)
(b) \( A^{2}-G^{2}=A^{2}-G^{2} \)
(c) \( A^{2}-A^{2}=G^{2}-G^{2} \)
(d) \( A^{2}+A^{2}=G^{2}+G^{2} \)
 0:06:47
0:06:47
 0:05:00
0:05:00
 0:00:33
0:00:33
 0:04:27
0:04:27
 0:04:31
0:04:31
 0:10:10
0:10:10
 0:08:55
0:08:55
 0:02:34
0:02:34
![[MPSI/MP2I] Ensembles et](https://i.ytimg.com/vi/CoHkEIW0W9g/hqdefault.jpg) 0:09:04
0:09:04
 0:20:33
0:20:33
 0:20:53
0:20:53
 0:02:42
0:02:42
 0:03:09
0:03:09
 0:01:52
0:01:52
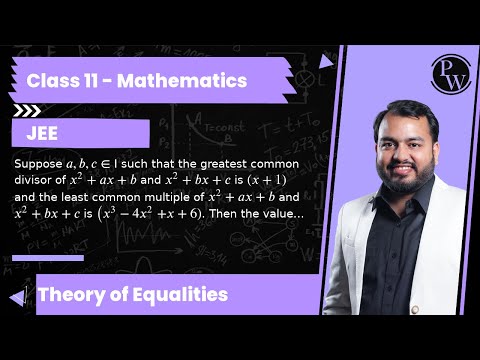 0:05:43
0:05:43
 0:03:14
0:03:14
 0:00:04
0:00:04
 0:14:06
0:14:06
 0:02:39
0:02:39
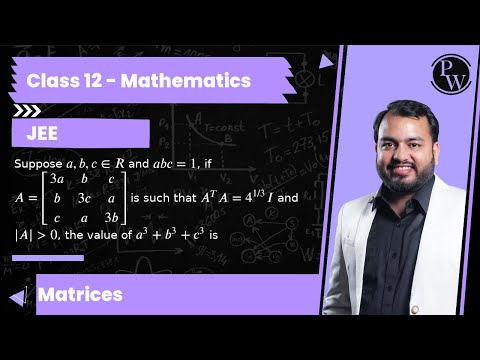 0:03:34
0:03:34
 0:08:27
0:08:27
 0:04:11
0:04:11
 0:03:19
0:03:19
 0:04:51
0:04:51