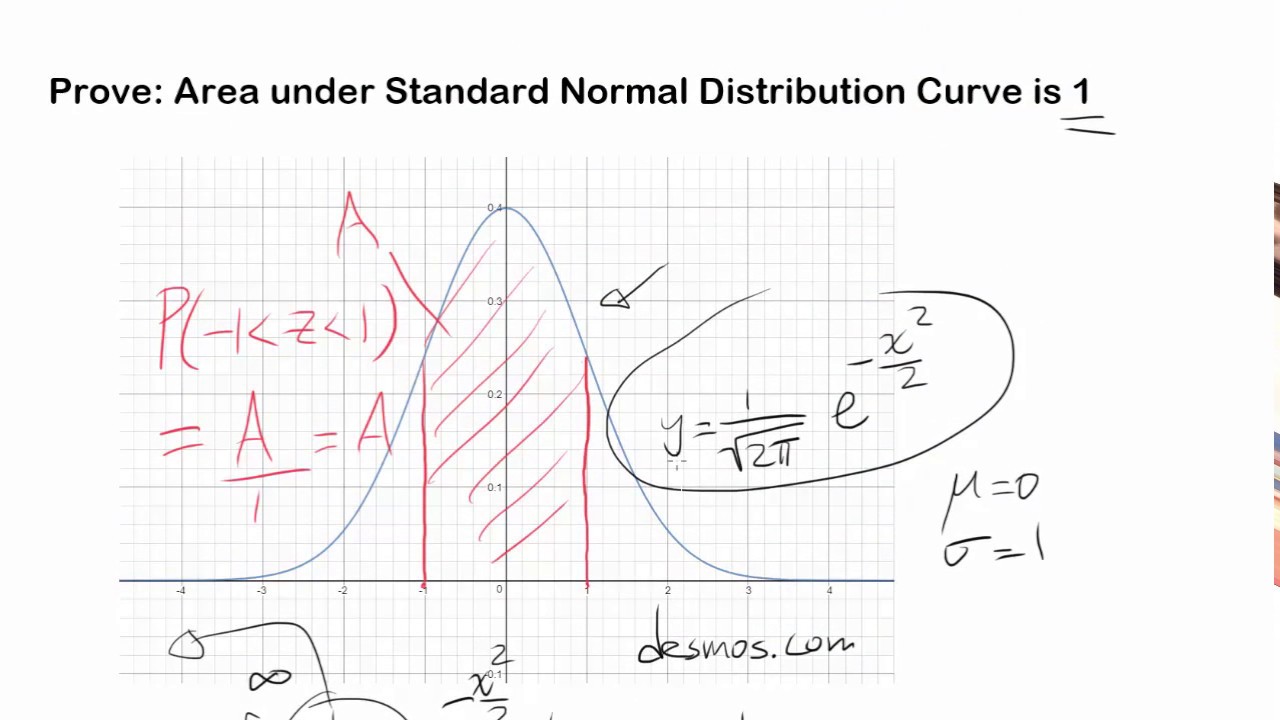
Prove: Area under Standard Normal Curve is 1

preview_player
Показать описание
Let's prove that the area under a standard normal curve (a bell-shaped curve with mean of 0 and standard deviation of 1) is equal to one.

Your support is truly a huge encouragement.
Please take a second to subscribe in order to send us your valuable support and receive notifications for new videos!

Every subscriber and like are immensely appreciated.
  1. LetsSolveMathProblems
  2. area
  3. one
  4. snd
  5. standard
  6. normal
Рекомендации по теме
Prove: Area under 0:08:15

Prove: Area under Standard Normal Curve is 1

Finding Areas Under 0:07:09

Finding Areas Under And What Is The Standard Normal Distribution Curve And Z Scores Explained

Standard Normal Distribution 0:51:03

Standard Normal Distribution Tables, Z Scores, Probability & Empirical Rule - Stats

Z-Scores, Standardization, and 0:06:57

Z-Scores, Standardization, and the Standard Normal Distribution (5.3)

Proof: Integral of 0:06:19

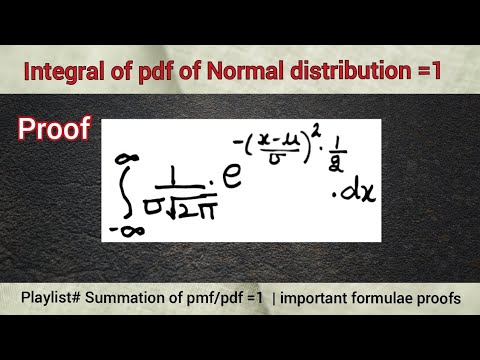
Proof: Integral of PDF of Normal Distribution is Equal to 1 (in English)

Normal Distribution (PDF, 0:05:26

Normal Distribution (PDF, CDF, PPF) in 3 Minutes

The Normal Distribution, 0:05:13

The Normal Distribution, Clearly Explained!!!

What does area 0:12:39

What does area have to do with slope? | Chapter 9, Essence of calculus

Why π is 0:24:46

Why π is in the normal distribution (beyond integral tricks)

Show that area 0:13:07

Show that area under the normal curve is always equal to 1.(Proof with Raza Latif)

Prove the Area 0:24:18

Prove the Area Under Normal Distribution is One

The Bell Curve 0:01:04

The Bell Curve (Normal/Gaussian Distribution) Explained in One Minute: From Definition to Examples

Normal Distribution | 0:16:35

Normal Distribution | Area under normal curve is unity proof

NORMAL DISTRIBUTION AREA 0:18:22

NORMAL DISTRIBUTION AREA PROOF

prove that area 0:03:39

prove that area under the Normal Curve is one

How To Find 0:00:25

How To Find The Total Area Under A Normal Distribution Curve

The Area under 0:07:32

The Area under the Normal Curve is 1

The Standard Normal 0:00:15

The Standard Normal Distribution (Proof of the Central Limit Theorem coming soon!)

NORMAL DISTRIBUTION |Area 0:19:52

NORMAL DISTRIBUTION |Area Under Normal Curve | Easiest Method | Probability

proof of integration 0:03:25

proof of integration of pdf of normal distribution is equal to 1

show that the 0:07:55

show that the area under the normal curve is unity(normal distribution lecture 2)

Properties of normal 0:21:19

Properties of normal distribution with proof area under the curve is one ch 9 lec 2

Normal Distribution EXPLAINED 0:10:59

Normal Distribution EXPLAINED with Examples

The Normal Distribution 0:08:50

The Normal Distribution and the 68-95-99.7 Rule (5.2)

Комментарии
Автор

Thanks for the work mate, God bless!!!

testing-lv
Автор

pretty great explanation and I like your style :)

MEE
Автор

Amazona. Treta videos, thanks. Coludidos you créate progresiva playlists to advance in polar integrales maybe?

algebraparatodos
Автор

I searched for this thanks. Also i have a question: in our math lecture my teacher explain this curve for e^-x^2. Is there a difference?

akif
Автор

Sir i m in class 12th please tell me i dont understand how u multiply 2 multiple and my sir told that e^x^2 is out of class 12 syllabus ....please tell me....

neerajchoprarealid
Автор

Hey, nice video ! What if i say :
The normal distribution is a probability distribution, the biggest value will be 1 (Since P<1 or P = 1). So, if we want to get ex.
the area of μ-3σ or μ+3σ we will get : integral 1/σradical2π * e^ (x-μ)^2/ 2σ^2 = P ( μ-3σ <= z <= μ+3σ) = 99, 7%. So by this :
integral 1/σradical2π * e^ (x-μ)^2/ 2σ^2 = P ( -oo <= z <= +oo) = 1
is it correct ?

BLooDyHD