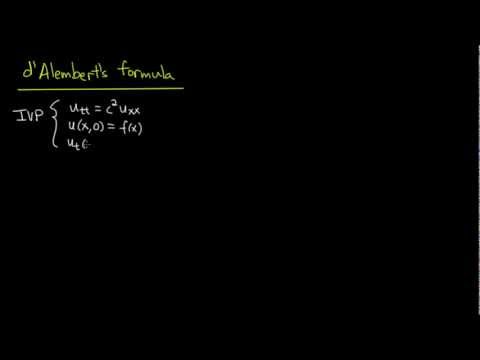
PDE 10 | Wave equation: d'Alembert's formula

An introduction to partial differential equations.

Part 10 topics:
-- derivation of d'Alembert's formula

[vadimartemov]

I have my masters admissions interview tomorrow, and I watch these to prepare! Really helped me to learn PDE from 0 to a manageable level just in two days! Thank you!

[HotPepperLala]

I JUST did this today in class!!! Please make more PDE videos! You are saving my math career here. I have to wake up every morning at 6:30am and fall asleep in class at 8:00am to 12:00am (summer class, so it's long).

[aapkamentor]

While introducing G(x) you integrated it to g(x)=c(p(x)+q(x)) without considering integration constant. How is it justified?

[geoffrey]

my professor glossed over this, the whole class forgot about it, and it showed up on the midterm. Everyone was completely stumped it was hilarious.

[PrashantSingh-rmzr]

I was having hard time in understanding this proof from apostol calculus volume 2, u made it simple dude, thanks a ton😍

[Ebn.Muhamed]

thanks bro for the lecture i didn't attend my college's lec. so it really helped me .

[sammanirox]

I don't understand how the choice of G(x)=-cp(x)+c q(x) is justified.(How it was thought to be the suitable G(x))

[someonetoogoodforyou]

I'm watching all of your videos. They're really excellent. Thank you

[mohegypt]

Good video, there's just one thing that I don't understand. At 6:52, when you divided the bottom eqn by C and added them together, you will have P(x)-P(x) which =0 and also if you divided it by C, then how are you still getting C in the equation? 

[raymondreed]

I have a hard time understanding how we are able to change arguments of x into arguments of x+-ct at 7:37. Weren't our initial position and initial velocity equations formed on the condition that they are equations of the SAME argument? how can we derive new equations and suddenly involve time in the arguments for p and q after deriving our equations at t=0?

[alvarot.]

You're computing the derivative with respect to t and then saying that the antiderivative is respecto to x??

[danieltambunan]

where do we get our general sollution of EM wave? is it from the Helmholtz then find the solution of E (electric field)?

[janina]

You already did this in previous videos but I just realized: In order for (x+ct) to make sense (and since x is an element of R^n as I understand) c needs to be in R^n as well, right? Because t is also just a real number, not a vector. So what do you mean by 1/c? Sorry if I'm missing something obvious...

[ericyoung]

Thanks for your video. I got confused how to classify the PDEs. is there any relations between first order PDEs and second order PDE?

[malkhaz.jokhadze]

Hello, what is the software that you are using?

[AhmedMohammed-wpke]

Thank You so much!, it was really helpful 

[multipledone]

What about Heat equation : d elembert solution

[natiyonatan]

thee video is very helpful
but i have a ques:
in most of the case i found that this is the general wave equation u(x, t) = f(c t - x) + g(c t + x)
but here (in the video) its written u(x, t) = f(x-ct) + g(c t + x)
help please...

[thehorizontries]

When you find u_t you differentiate p and q with respect to t but you used prime which is usually reserved for x derivatives and then you undo that by integrating with respect to x which makes no sense

[krishnaraghav]

which software do you use to write this?