filmov
tv
A Homemade Exponential System

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #ExponentialEquations #Exponentials
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
#ChallengingMathProblems #ExponentialEquations #Exponentials
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
A Homemade Exponential System
Solving A Homemade Exponential Equation
A Home-made Exponential Equation
A Homemade Exponential Equation
A Quick and Easy Exponential System
Let's Solve A Homemade Exponential
A Nice Homemade Exponential Equation
A Homemade Exponential Log Equation
A Homemade Exponential Equation
A Homemade Exponential Equation Solved in Three Ways
How To Solve A Homemade Exponential Equation
A Homemade Exponential Equation
Solving an Exponential System in Two Ways
An Exponential System | Real Solutions
Solving A Nice And Easy Exponential System
A Nice Exponential System | Two Ways
A Homemade Exponential Equation | 3^{x^2}9^x=27^{{sqrt(x)}
A Homemade Exponential Equation, x^x=2^{1/x}
Solving A Homemade Eulerian Exponential Equation
An Interesting Exponential Equation | Homemade
NEWYES Calculator VS Casio calculator
Solving a Nice Homemade Exponential Equation
Working with an Exponential System
Solving an Exponential System in Two Ways
Комментарии
 0:05:11
0:05:11
 0:08:46
0:08:46
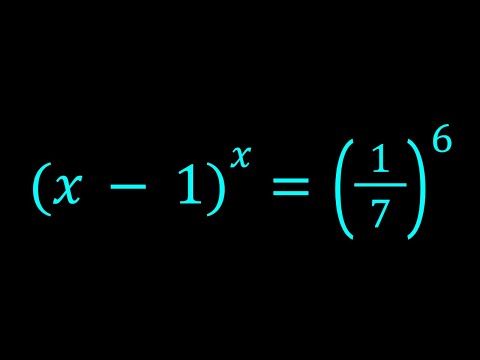 0:05:06
0:05:06
 0:10:08
0:10:08
 0:10:35
0:10:35
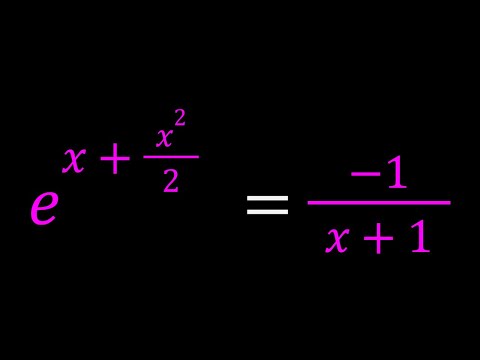 0:07:11
0:07:11
 0:05:31
0:05:31
 0:09:14
0:09:14
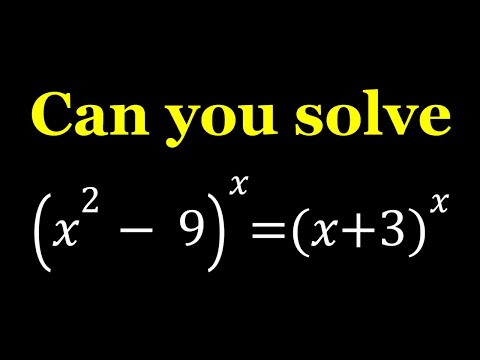 0:08:30
0:08:30
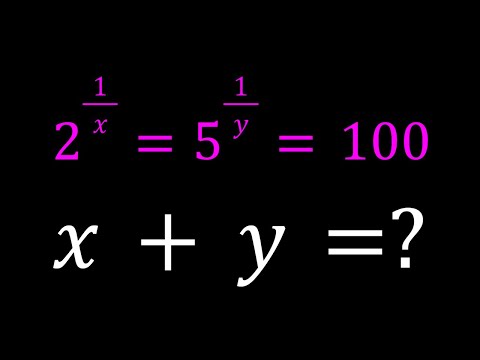 0:09:15
0:09:15
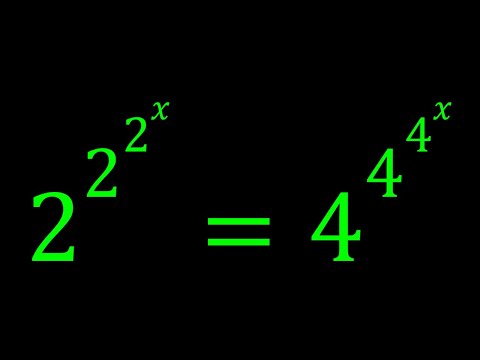 0:12:59
0:12:59
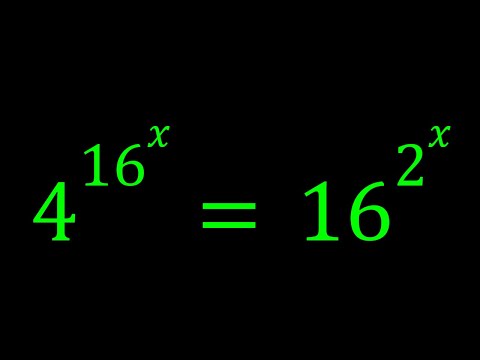 0:04:51
0:04:51
 0:10:59
0:10:59
 0:10:11
0:10:11
 0:09:38
0:09:38
 0:09:26
0:09:26
 0:08:59
0:08:59
 0:10:39
0:10:39
 0:09:18
0:09:18
 0:04:49
0:04:49
 0:00:14
0:00:14
 0:11:52
0:11:52
 0:08:36
0:08:36
 0:09:44
0:09:44