filmov
tv
Linear Algebra: 011 Vector Spaces VI Bases and Dimension

Показать описание
Linear Algebra: 011 Vector Spaces VI Bases and Dimension
Vector Spaces Explained | Linear Algebra
Abstract vector spaces | Chapter 16, Essence of linear algebra
Vector intro for linear algebra | Vectors and spaces | Linear Algebra | Khan Academy
Vector spaces | Lecture 16 | Matrix Algebra for Engineers
Linear Algebra 4.5.1 The Dimension of a Vector Space
4.1 Vector Spaces and Subspaces
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Linear Algebra: coordinates for abstract vector space, trace proof for dimension sketched, 8-30-24
Linear Algebra Lecture 14 | Vector Spaces Part 1 | Example and Non- examples of Vector Spaces
Vectors | Chapter 1, Essence of linear algebra
Linear Transformations on Vector Spaces
Linear Algebra Example Problems - Vector Space Basis Example #1
[Linear Algebra] Dimension
4.1 - Real Vector Spaces (Part 1)
Linear Algebra - 11 - Span
Subspaces and Span
Vector Spaces and Subspaces – Linear Algebra
Adding vectors | Vectors and spaces | Linear Algebra | Khan Academy
Linear Algebra: Vector Spaces
Linear combinations and span | Vectors and spaces | Linear Algebra | Khan Academy
Vector Spaces | Definition & Examples
Linear Algebra||Vector Space|| Group ||Field Lec:01
4.1 || Vector Spaces and Subspaces
Комментарии
 0:57:52
0:57:52
 0:17:30
0:17:30
 0:16:46
0:16:46
 0:05:49
0:05:49
 0:07:23
0:07:23
 0:09:27
0:09:27
 1:14:57
1:14:57
 0:09:59
0:09:59
 0:52:55
0:52:55
 0:27:55
0:27:55
 0:09:52
0:09:52
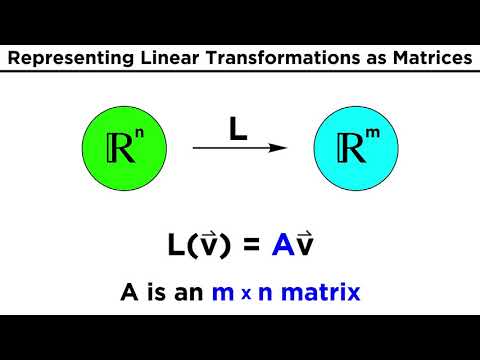 0:09:11
0:09:11
 0:04:47
0:04:47
![[Linear Algebra] Dimension](https://i.ytimg.com/vi/zr_iMt0k5TQ/hqdefault.jpg) 0:11:14
0:11:14
 0:24:45
0:24:45
 0:10:27
0:10:27
 0:05:50
0:05:50
 0:11:24
0:11:24
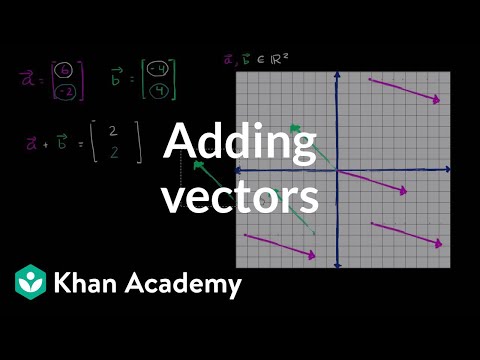 0:07:12
0:07:12
 0:27:36
0:27:36
 0:20:35
0:20:35
 0:08:11
0:08:11
 0:17:29
0:17:29
 0:35:11
0:35:11