Limit of sin(3x)/x as x approaches 0 | Calculus 1 Exercises

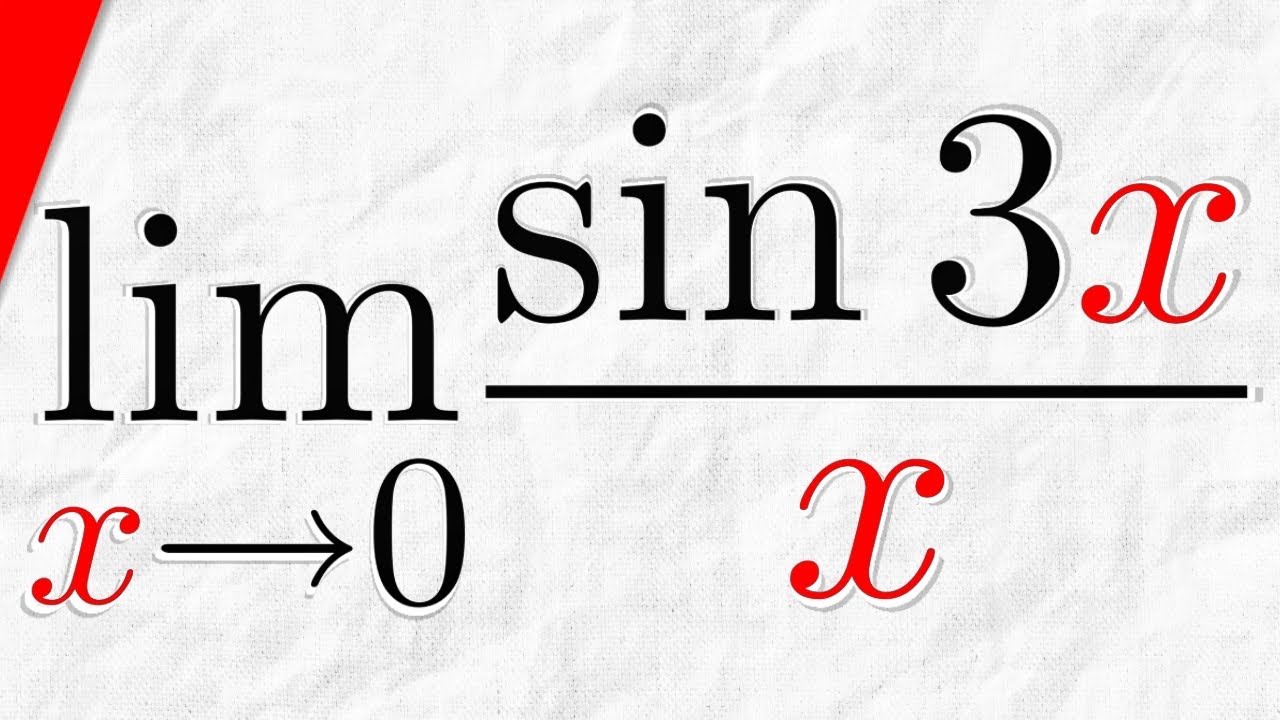
preview_player
Показать описание
We show the limit of sin(3x)/x as x goes to 0 is equal to 3. To evaluate this trigonometric limit, we need to remember the limit of sin(x)/x with x approaching 0, which is a fundamental trigonometric limit equal to 1! If we remember this, we'll be able to solve the problem in short order. #Calculus1 #apcalculus

◉Textbooks I Like◉

★DONATE★

Thanks to Loke Tan, Raül Beienheimer, Matt Venia, Micheline, Doug Walker, Odd Hultberg, Marc, Shlome Ashkenazi, Barbora Sharrock, Mohamad Nossier, Rolf Waefler, Shadow Master, and James Mead for their generous support on Patreon!

Outro music is mine. You cannot find it anywhere, for now.

Follow Wrath of Math on...

  1. Wrath of Math
  2. wrath of math
  3. limit of sinx/x as x approaches 0
  4. limit of sinx/x
  5. sin(2x)/x as x approaches 0
  6. limit of sin2x/x as x approaches 0
Рекомендации по теме
Limit of sin(3x)/x 0:02:59

Limit of sin(3x)/x as x approaches 0 | Calculus 1 Exercises

Find the limit 0:03:28

Find the limit of sin 3x divided by x as x approaches 0

Limit of sin3x/x 0:01:24

Limit of sin3x/x when x tends to 0

Find the limit. 0:01:24

Find the limit. lim x= 0 sin(3x)/x

Limit of sin(3x)/2x 0:03:20

Limit of sin(3x)/2x as x approaches 0, (check desc. for similar videos)

Find the limit 0:02:01

Find the limit of sin 3x over x as x gets closer to 0 ODM3 Review Q8

Limit of sin(7x)/sin(3x) 0:01:46

Limit of sin(7x)/sin(3x) as x Approaches Zero

limit of 3x/tan(x)) 0:01:48

limit of 3x/tan(x)) as x goes to 0 ( limits of trigonometric functions )

AP Calculus AB 0:01:59

AP Calculus AB 4.7 Using L'Hôpital's Rule to Find the Limit of a Rational Function ( sin(3...

Limit as x 0:01:01

Limit as x approaches zero of sin(3x)/(2x)

Find the limit 0:03:30

Find the limit as x approaches 0 of (sin 3x)/(sin 2x)

Limit (x-sin x)/x^3 0:13:14

Limit (x-sin x)/x^3 as x goes to 0

How to Use 0:02:09

How to Use L'hopitals Rule to find the limit of sin(2x)/sin(3x) as x approaches zero

Find the limit 0:02:41

Find the limit of sin 3x times sin 5x divided by x squared as x approaches zero

😎🔥Limit of sin(3x)/(2x) 0:01:28

😎🔥Limit of sin(3x)/(2x) as x ➡️ 0 in 1.5 Minutes!✅

Limit as x 0:04:36

Limit as x approaches 0 y = sin^3x/(sinx-tanx)

Find the limit 0:03:29

Find the limit of sin 3x divided by 5x cubed - 4x as x approaches zero sin 3x/(5x^3 -4x)

lim x approaches 0:11:55

lim x approaches 0: sin2x/x, sin3x/x, sin4x/x, sin5x/x

Limit of x-sinx/x^3 0:01:35

Limit of x-sinx/x^3 as x approaches 0

Find the limit 0:03:04

Find the limit of sin(2x)/tan(3x) as x approaches 0

Lim sin2x/sin3x as 0:04:04

Lim sin2x/sin3x as x goes to 0

`underset(x to 0)(lim) 0:01:59

`underset(x to 0)(lim) (sin 3x)/(x)` is equal to

Limit of sin(4x)/x 0:01:03

Limit of sin(4x)/x as x Approaches Zero

Limit of sin^8(x)/x 0:02:40

Limit of sin^8(x)/x as x approaches 0

Комментарии
Автор

Help support the production of this course by joining Wrath of Math to access exclusive and early Calc 1 videos, plus lecture notes at the premium tier!

WrathofMath
Автор

Very clear and simple explanation. 2:58

EE-Spectrum
Автор

so we can generally say

lim (x -> 0) [sin(cx) / x] = c
lim (x -> 0) [sin(x) / (cx)] = 1/c

am i right?

adi-tiny
Автор

Is it because when you use l'hopital's rule it is equal to 1? Because when you differentiate the top and bottom you get cos theta/1 and substituting the limit you get cos(0)=1 and 1/1=1.

syndrac
Автор

how about when there is a #x in the denominator rather than sin(#x) -- ex: sin(x)/5x

eXCey