10 - Series and Sigma Summation Notation - Part 1 (Geometric Series & Infinite Series)

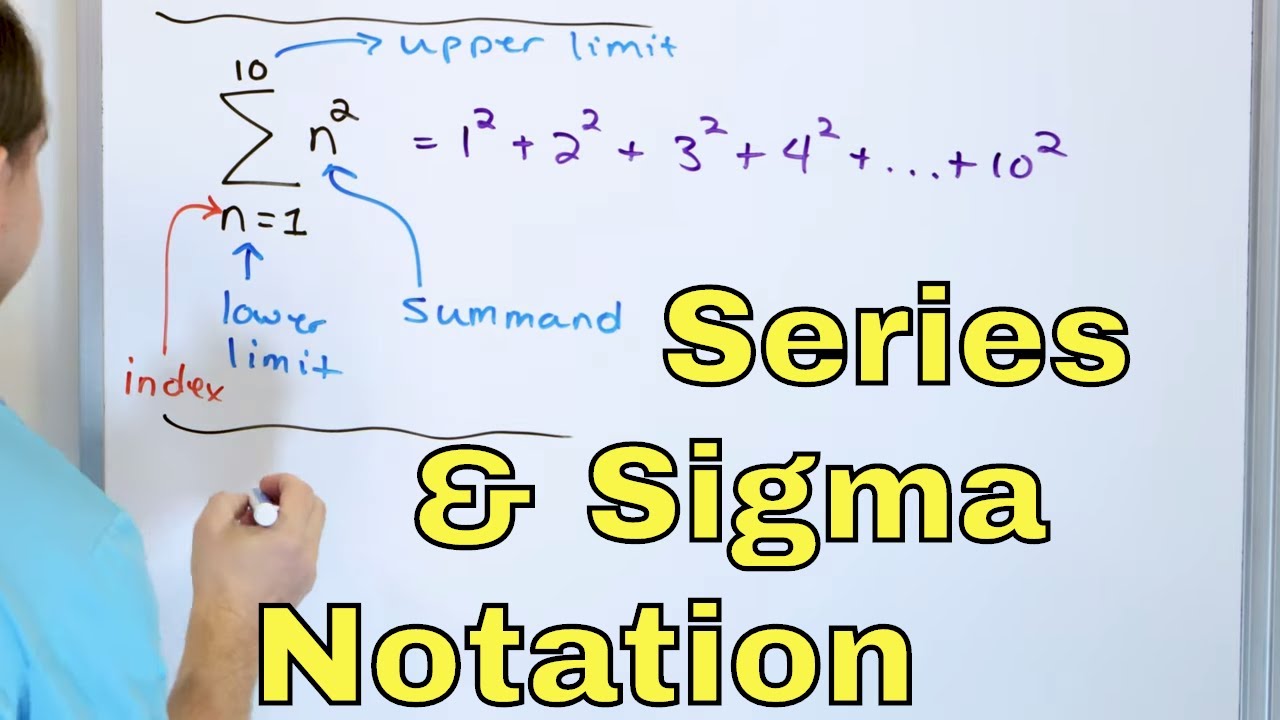
In this lesson, you will learn what a series is in math and how they compare to sequences. A series is the sum of the terms of a sequence. We have already discussed some special sequences such as the arithmetic sequence and geometric sequence. We now extend this idea and discuss the arithmetic and geometric series, infinite series, finite series, and more. We also spend a great deal of time introducing the sigma notation for summation, which is how we actually write the series down when solving problems..

[josephciaravino]

This man is a gift to humanity! 😆 I love how he takes time to point things out any sensible person would just accept as given and breeze over. 👍Thank you!

[prettyalina]

Was feeling anxiety going into this. Im happy to say I understand now. Nothing to be afraid about. You're a wonderful teacher, please keep up the good work!

[real_essential_protectiona]

AMAZING! So glad I found this channel 😀Phenomenal teacher.

[engmohamoudhassan]

Thanks professor.
Your explanation is amazing and shows extraordinary teaching experience

[marlenebaugh]

This instructor makes life with maths so easy! I am preparing for my college algebra (clep) and your videos are very very helpful. Thanks Mr Jason

[amerx]

the best teacher ever. I am learning java from his website and it is great. I watched some of his math videos and he is unbelievably good. Thanks a lot from all my hart.

[robertstack]

In the60s engineering cirrculum the general idea of sequence, series was presented and executed in a matter of seconds and the student, smart or dumb was expected to digest and assume 100% genius of the topics. Unhappily I was the "dumb." Now after watching these presentations by this gentleman, I want my $410/semester tuition back for all the eight courses In the two semesters I attended at U of M. The problem was not me, but the instructors. These people were ineffectual and presumptive. This man on these and many other courses obviously has been gifted my expert knowledge of many subjects, but also quite adept at teaching in a simple clear concise manner. He breaks esoteric subjects down into simple ideas and equations that anyone can understand. Moreover and importantly, he developes the interest in furthering their education. I especially enjoyed his videos on Laplace Transforms and Elementary Differential Equations. It is my wish that he would write a text and name it Higher Mathematics for Scientists and Engineers, a similar title used by Ivan Sokolnoff. The difference between the two texts would be this author's text would be understandable for us dumb engineers.

[TheAdamsalam]

I cannot emphasize enough how amazing was your explanation of the topic, , Thanks a lot
I wish you keep doing such a great job

[ኢትዬ]

I love you start with the derivations unlike other YouTubers who go straight to the formula

[abukarmurudimumin]

This is a wonderful math teacher who can teach math to me easily since he's very experienced Prof!
He taught to me more about high math, thank you very much.

[jkscout]

If only more professors would quit professing and actually teach like this. You are an amazing teacher!

[philn]

Perfect explanation thank you. I'm self teaching linear algebra, calculus, and more advanced math - I find your tutorials just gold.

[dominikawisniewska]

from online school i can’t learn anything your videos are a huge help thank you

[ShaktimanTamang-wfkd]

Wonderful way to teach here. I loved it. Thanks a lot sir.
I am from Nepal😊😊

[sleepygrumpy]

Excellent content you are a very gifted teacher sir!!!

[nicanorrocamora]

Clearly explained Sir.. Thanks for the knowledge you imparted to me..

[hambalipearson]

Thank you sir you have made this topic really simple

[LarryZAR]

9:11
In order for you to get to 1 you have to get to 1/2 before that 1/4 before that 1/8 etc
Therefore
sum of (1/2+1/4+1/8...)=1

[rrrbb]

whenever possible, it's helpful to define infinite series using recursion. you can just solve for its value in most cases:

S = 1 + (1/2)S.
(1-(1/2))S = 1.
(1/2)S = 1.
S=2.

S= (1/2)^0 + (1/2)^1 + (1/2)^2 + ... + (1/2)^n + (1/2)^(n+1) S.
= 1 + 1/2 + 1/4 + 1/8 + ... + 1/(2^n) + (1/2)^(n+1)S.

Note that the last term is multiplied times S. It's the tail of S expanded n times. But something AMAZING happens if you are careful. S isn't really the value. If the tail of the recursion goes to zero S = Sum(S, n), when n goes to infinity, because Tail(S, n) goes to zero.

S = Sum(S, n) + Tail(S, n).

This lets you calculate the closed form formula for n terms!

S - Tail(S, n) = Sum(S, n).

S - (1/2)^(n+1)S = Sum(S, n).
S(1 - (1/2)^(n+1)) = Sum(S, n).
2(1 - (1/2)^(n+1)) = Sum(S, n).
2 - (1/2)^n = Sum(S, n).

try it out...

adding the first 4 terms 0..3 replacements is...

Sum(S, 3) = 1/1 + 1/2 + 1/4 +1/8 = 15/8
= 2-(1/2)^3 = 2 - (1/8) = (2*8-1)/8 = 15/8

I honestly have no idea how people figure out most infinite series closed forms without using recursion.

Note the sum like 1/2 + 1/4 + 1/8 + ... = 1:

S = 1/2 + 1/2 S.
2S = 1 + S.
S = 1.
S
= 1/2 + 1/2 (1/2 + 1/2 S)
= 1/2 + 1/4 + 1/8 + ... + 1/(2^n) + 1/(2^(n+1))S.

and this to top it off:

S = 0.9 + 0.1 S
10 S = 9 + S
9 S = 9
S = 1

S = 1
= 0.9 + 0.1(0.9 + 0.1 S)
= 0.9 + 0.09 + 0.01(0.9 + 0.1 S)
= 0.9999....

And this works perfectly well for divergent series like "-1 = 1+2+4+8+...", where it is very clear what's going on. S=Sum(S, n) only when Tail(S, n) is zero; S is an important number in calculating the closed form; and is not necessarily the total. It is part of the recursive definition that replaces infinite iteration.

-1/12 = S
-1 = 12 S
1 = -12 S
1 = (1-13)S
13 S + 1 = S

S = 1 + 13 S
= 13^0 + 13 S
= 13^0 + 13(13^0 + 13 S)
= 13^0 + 13^1 + 13^2 + ... + 13^n + 13^(n+1) S

subtract the tail, and you have a formula for the first 13 powers.

more generally...

A = 1 + x A
(1-x)A = 1/(1-x)

When you differentiate this, you get the famous "-1/12 = 1+2+3+4+..." strange sequence. It is no paradox though because S isn't the value. S-Tail(S, n) is the value, and that goes to infinity as n goes to infinity, and it gives you the n(n+1)/2 formula, in case you didn't know it.

[dfuzesavetf]

how can someone be this talented at teaching