filmov
tv
Use Laurent series to find the residue

Показать описание
This video shows how to find the residue of the function f(z) where z=a+bi and i=sqrt(-1) for the the function at 1 .
First part is to find the Laurent series at 1 and then find the z^-1 coefficient .
this gives us res(f,1) .
Here the function is 1/(z(z-1)^2)
#complexnumbers
#complexlog
#euler
#imagianary
#real
#polarn
#mathtricks
#maths
#mathematics
#residue
First part is to find the Laurent series at 1 and then find the z^-1 coefficient .
this gives us res(f,1) .
Here the function is 1/(z(z-1)^2)
#complexnumbers
#complexlog
#euler
#imagianary
#real
#polarn
#mathtricks
#maths
#mathematics
#residue
Laurent Series Explained | How to Determine Laurent Series | Complex Analysis #9
Laurent Series and Taylor Series, when to use which? | Complex Analysis #10
Example of Laurent's Series | L16 | TYBSc Maths | Complex Power Series @ranjankhatu
Laurent Series Harder Example
Find the Laurent Series and use the Ratio Test to find the Annulus of Convergence
Use Laurent series to find the residue
Complex Analysis 11: Laurent Series
Use Laurent Series to Find the Residues
Finding Residue Using Laurent Series Expansion | Example | L22 | Residue Theorem @ranjankhatu
Laurent Series of Complex Functions
Laurent Series of f(z) = 1/(z - 3) in the Region |z| greater than 3
Complex Analysis Laurent Series Example
Example of Laurent's Series | L 14 | TYBSc Maths | Complex Power Series @ranjankhatu
Example of Laurent's Series | L15 | TYBSc Maths | Complex Power Series @ranjankhatu
problem on taylor's series in complex analysis|problem on laurent series|Theta Classes
[Complex Analysis] Find the Laurent Series for z/(z+1)(z-2) when |z| is greater than 2
Find the Residue of a Function using Laurent Series and known Taylor Series
19. Taylor's and Laurent's Series | Problem#1 | Most Important | Complete Concept
Laurent Series of the Function f(z)=e^2z/(z-1)^3
Integration using Laurent Series and Residues
Complex Analysis -Laurent Series For Complex Number | Problems By GP
Taylor's and Laurent's Series Examples (complex analysis)
Basic Complex Analysis - Unit 3 - Lecture 12 - Example of Laurent Series
Taylor and Maclaurin Series
Комментарии
 0:13:56
0:13:56
 0:04:14
0:04:14
 0:14:04
0:14:04
 0:05:50
0:05:50
 0:14:01
0:14:01
 0:04:48
0:04:48
 0:07:16
0:07:16
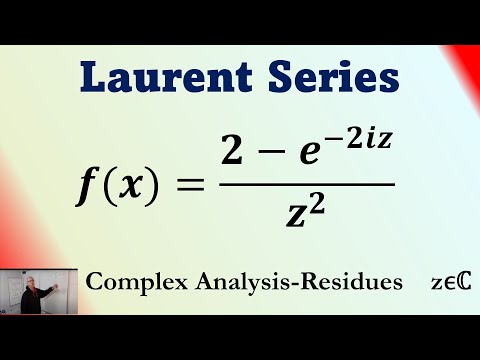 0:13:43
0:13:43
 0:07:23
0:07:23
 0:07:20
0:07:20
 0:02:40
0:02:40
 0:04:48
0:04:48
 0:23:47
0:23:47
 0:24:50
0:24:50
 0:16:10
0:16:10
![[Complex Analysis] Find](https://i.ytimg.com/vi/MKEt30jni78/hqdefault.jpg) 0:03:10
0:03:10
 0:08:21
0:08:21
 0:11:25
0:11:25
 0:12:57
0:12:57
 0:09:55
0:09:55
 0:18:31
0:18:31
 0:28:20
0:28:20
 0:05:47
0:05:47
 0:09:34
0:09:34