filmov
tv
Laurent Series Explained | How to Determine Laurent Series | Complex Analysis #9
Показать описание
Everything you need to know about Laurent Series explained. The video will contain problems on Laurent Series and how to solve them all for each Laurent Series.
The video will include concepts as:
► Definition of Laurent Series
► Principal part and Analytic part of a Laurent Series
► Convergence of the Principal part and Analytic part
► A theorem about when we can use Laurent Series
► How to determine all possible Laurent Series around some point of expansion
► How to solve Laurent Series.
LINK TO COMPLEX ANALYSIS PLAYLIST
LINK TO CANVAS
SUPPORT
Consider subscribing, liking or leaving a comment, if you enjoyed the video or if it helped you understand the subject. It really helps me a lot.
CLARIFICATION OF THE METHOD:
To determine all possible Laurent Series:
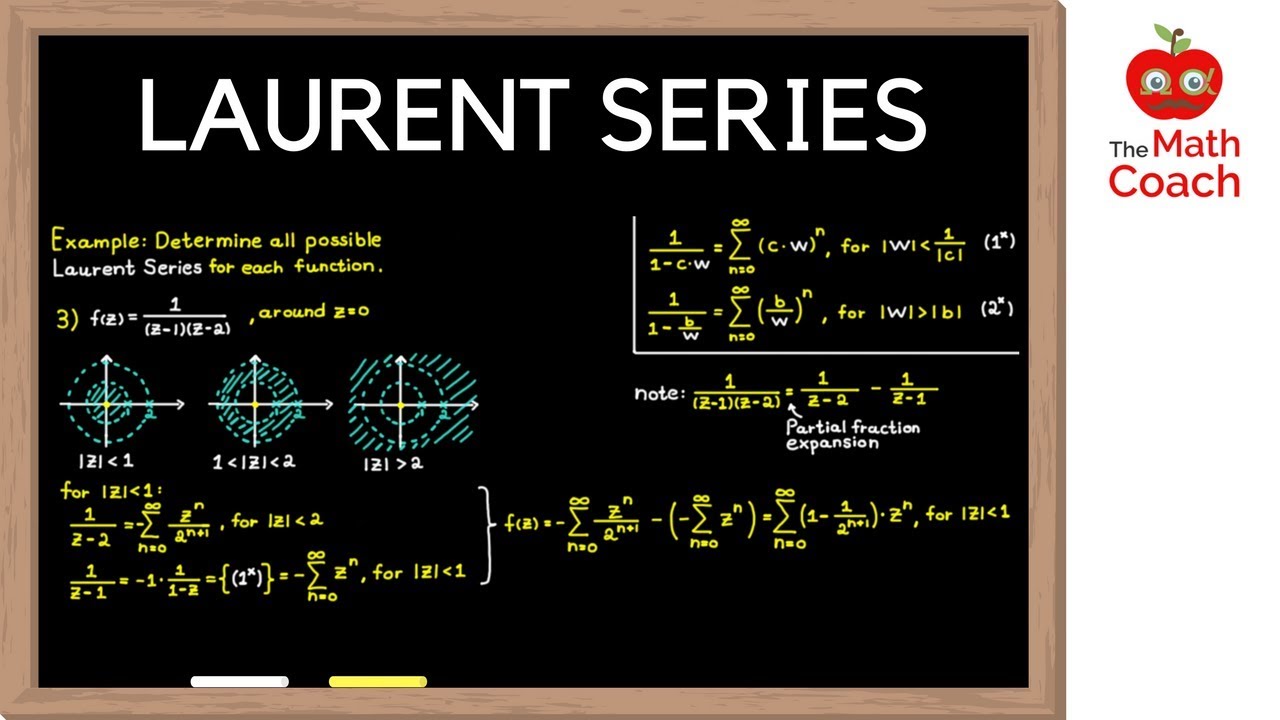
Start by marking the important points on the graph, the important points are the poles of the function and the point of expansion. The will divide the complex plan into several domains and each of these domains will have its own Laurent Series, since none of these domain will contain any poles of the function.
You may note that most of the time we only need to determine ONE of the parts (analytic or principal part) to determine the Laurent Series. This comes from the fact that both of these parts are only present (converges) if we are inside an annulus domain. If you want to determine a Laurent Series outside of a circle then you only need the principal part (since the analytic part will not converge in this domain) and if you want to determine the Laurent Series inside of a circle then you only need the Analytic part (since the principal part will not converge in this domain).
CONCEPTS FROM THE VIDEO
► Laurent Series
A Laurent Series is a specific kind of power series and is used to approximate an analytic function f(z) around some point z_0 (note that z_0 can be an isolated singularity) in the complex plane
... + (a_-3) *(z-z_0)^-3 + (a_-2) *(z-z_0)^-2 + (a_-1) *(z-z_0)^-1 + a_0 + a_1 *(z-z_0) + a_2 *(z-z_0)^2 + a_3 *(z-z_0)^3 + ...
where the coefficients a_n are determined by contour integration, but 99,9 % of the cases these are determined by geometric series. Note that: "... + (a_-3) *(z-z_0)^-3 + (a_-2) *(z-z_0)^-2" is called the principal part, while "a_0 + a_1 *(z-z_0) + a_2 *(z-z_0)^2 + a_3 *(z-z_0)^3 + ..." is called the analytic part.
►Geometric Series
A geometric series is a series with a constant ratio between successive terms. An example on a geometric series is
1/(1-w) = w^0 + w^1 + w^2 +... for abs(w) smaller than 1
which can be modified to the following
1/(1- 1/w) = (1/w)^0 + (1/w)^1 + (1/w)^2 +... for abs(w) bigger than 1
and the reason these two series are used a lot to solve these kind of problems, is because this geometric series looks pretty much like the Laurent Series we would like to obtain.
► Poles
A pole is a specific kind of singularity, the short and the most intuitive definition is that poles are points z_0 in the complex plane so that f(z_0) = g(z_0)/0, where g(z_0) =\= 0.
► Partial Fraction
The partial fraction decomposition or partial fraction expansion of a rational function is used to express the fraction as a sum of fractions with a simpler denominator.
[Link to video is coming].
TIMESTAMPS
00:00 Intro
00:13 Theorem Laurent Series
01:06 What is an Annulus domain
02:04 Good things to know
03:40 Why geometric series are the best
Examples: Determine all possible Laurent Series for the following functions
05:20 f(z) = 1/(z-2) around z=0
08:02 f(z) = 1/(z-2) around z=1
09:48 f(z) = 1/((z-1)(z-2)) around z=0
SOCIAL
SOURCES:
Fundamentals of Complex Analysis with Applications to Engineering, Science, and Mathematics: Pearson New International Edition.
HASHTAGS
#TheMathCoach #ComplexAnalysis #Complex Analysis Playlist
The video will include concepts as:
► Definition of Laurent Series
► Principal part and Analytic part of a Laurent Series
► Convergence of the Principal part and Analytic part
► A theorem about when we can use Laurent Series
► How to determine all possible Laurent Series around some point of expansion
► How to solve Laurent Series.
LINK TO COMPLEX ANALYSIS PLAYLIST
LINK TO CANVAS
SUPPORT
Consider subscribing, liking or leaving a comment, if you enjoyed the video or if it helped you understand the subject. It really helps me a lot.
CLARIFICATION OF THE METHOD:
To determine all possible Laurent Series:
Start by marking the important points on the graph, the important points are the poles of the function and the point of expansion. The will divide the complex plan into several domains and each of these domains will have its own Laurent Series, since none of these domain will contain any poles of the function.
You may note that most of the time we only need to determine ONE of the parts (analytic or principal part) to determine the Laurent Series. This comes from the fact that both of these parts are only present (converges) if we are inside an annulus domain. If you want to determine a Laurent Series outside of a circle then you only need the principal part (since the analytic part will not converge in this domain) and if you want to determine the Laurent Series inside of a circle then you only need the Analytic part (since the principal part will not converge in this domain).
CONCEPTS FROM THE VIDEO
► Laurent Series
A Laurent Series is a specific kind of power series and is used to approximate an analytic function f(z) around some point z_0 (note that z_0 can be an isolated singularity) in the complex plane
... + (a_-3) *(z-z_0)^-3 + (a_-2) *(z-z_0)^-2 + (a_-1) *(z-z_0)^-1 + a_0 + a_1 *(z-z_0) + a_2 *(z-z_0)^2 + a_3 *(z-z_0)^3 + ...
where the coefficients a_n are determined by contour integration, but 99,9 % of the cases these are determined by geometric series. Note that: "... + (a_-3) *(z-z_0)^-3 + (a_-2) *(z-z_0)^-2" is called the principal part, while "a_0 + a_1 *(z-z_0) + a_2 *(z-z_0)^2 + a_3 *(z-z_0)^3 + ..." is called the analytic part.
►Geometric Series
A geometric series is a series with a constant ratio between successive terms. An example on a geometric series is
1/(1-w) = w^0 + w^1 + w^2 +... for abs(w) smaller than 1
which can be modified to the following
1/(1- 1/w) = (1/w)^0 + (1/w)^1 + (1/w)^2 +... for abs(w) bigger than 1
and the reason these two series are used a lot to solve these kind of problems, is because this geometric series looks pretty much like the Laurent Series we would like to obtain.
► Poles
A pole is a specific kind of singularity, the short and the most intuitive definition is that poles are points z_0 in the complex plane so that f(z_0) = g(z_0)/0, where g(z_0) =\= 0.
► Partial Fraction
The partial fraction decomposition or partial fraction expansion of a rational function is used to express the fraction as a sum of fractions with a simpler denominator.
[Link to video is coming].
TIMESTAMPS
00:00 Intro
00:13 Theorem Laurent Series
01:06 What is an Annulus domain
02:04 Good things to know
03:40 Why geometric series are the best
Examples: Determine all possible Laurent Series for the following functions
05:20 f(z) = 1/(z-2) around z=0
08:02 f(z) = 1/(z-2) around z=1
09:48 f(z) = 1/((z-1)(z-2)) around z=0
SOCIAL
SOURCES:
Fundamentals of Complex Analysis with Applications to Engineering, Science, and Mathematics: Pearson New International Edition.
HASHTAGS
#TheMathCoach #ComplexAnalysis #Complex Analysis Playlist
0:13:56
Laurent Series Explained | How to Determine Laurent Series | Complex Analysis #9
0:07:16
Complex Analysis 11: Laurent Series
0:08:22
Complex Analysis 15 | Laurent Series
0:07:20
Laurent Series of Complex Functions
0:04:48
Complex Analysis Laurent Series Example
0:05:50
Laurent Series Harder Example
0:04:14
Laurent Series and Taylor Series, when to use which? | Complex Analysis #10
0:14:04
Example of Laurent's Series | L16 | TYBSc Maths | Complex Power Series @ranjankhatu
0:21:38
Laurent's Series | Laurent's Theorem (complex analysis)
0:06:10
The Laurent series of f(z)=exp(1/z)
0:28:47
Week7Lecture1: Laurent Series
![[Complex Analysis] Find](https://i.ytimg.com/vi/MKEt30jni78/hqdefault.jpg)
0:03:10
[Complex Analysis] Find the Laurent Series for z/(z+1)(z-2) when |z| is greater than 2
0:24:50
Example of Laurent's Series | L15 | TYBSc Maths | Complex Power Series @ranjankhatu
0:29:56
Laurent Decomposition -- Complex Analysis 19
0:29:56
Laurent series
0:08:32
Integrals using Laurent Series (C a unit circle centered @ origin)
0:07:21
Laurent Expansion (Series) || Example 1|| Complex Analysis MAT324
0:10:12
Taylor’s and Laurent’s series/Complex Integration(Laurent’s Series) problems in simple explanation...
0:09:55
Laurent's Theorem - Complex Analysis by a Physicist
0:23:47
Example of Laurent's Series | L 14 | TYBSc Maths | Complex Power Series @ranjankhatu
0:19:16
mod04lec32 - Laurent Series
0:02:40
Laurent Series of f(z) = 1/(z - 3) in the Region |z| greater than 3
0:07:14
How to find Laurent Series about a point | IAS Math | Previous Year Solution | Complex Analysis
0:09:36
Complex Analysis 10: Taylor Series
Комментарии