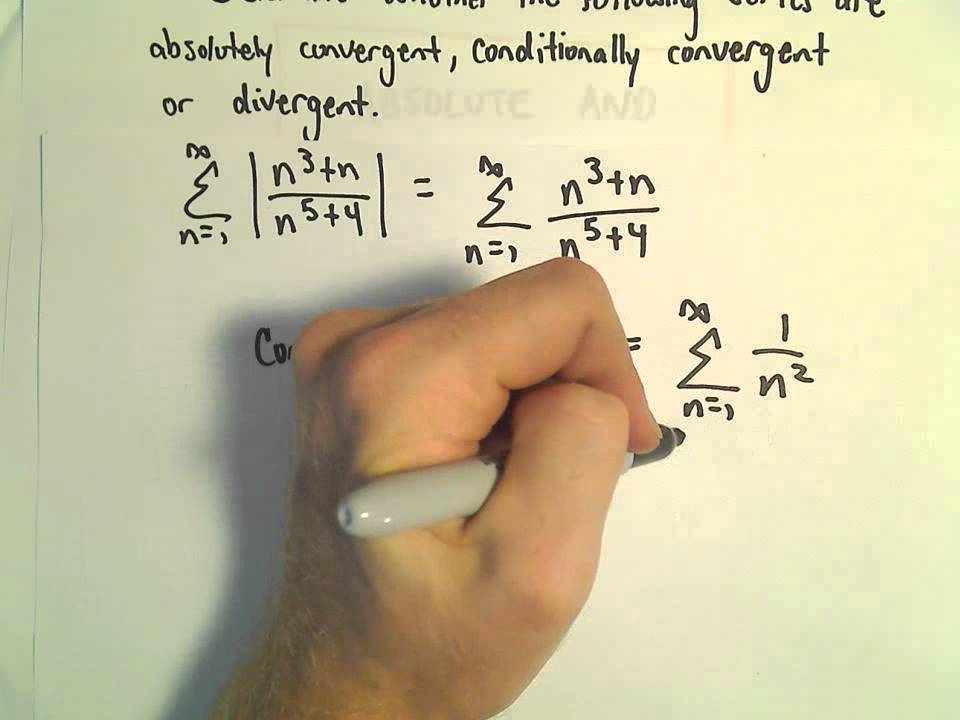
Absolute Convergence, Conditional Convergence, Another Example 3

preview_player
Показать описание
  1. patrickJMT
  2. series
  3. converge
  4. diverge
  5. absolute
  6. conditional
Рекомендации по теме
Absolute Convergence, Conditional 0:13:07

Absolute Convergence, Conditional Convergence, and Divergence

Absolute Convergence vs 0:03:41

Absolute Convergence vs Conditional Convergence vs Convergence

Absolute Convergence, Conditional 0:02:35

Absolute Convergence, Conditional Convergence, Another Example 1

Absolute and Conditional 0:05:26

Absolute and Conditional Convergence

Absolute & Conditional 0:11:22

Absolute & Conditional Convergence - Example 1

Conditional & absolute 0:05:00

Conditional & absolute convergence | Series | AP Calculus BC | Khan Academy

Absolute Convergence, Conditional 0:02:09

Absolute Convergence, Conditional Convergence, Another Example 3

Absolutely and Conditionally 0:08:33

Absolutely and Conditionally Convergent Series

Absolute Convergence, Conditional 0:11:21

Absolute Convergence, Conditional Convergence and Divergence

Checking for absolute 0:19:15

Checking for absolute convergence (5 examples)

Calculus BC – 0:16:04

Calculus BC – 10.9 Determining Absolute or Conditional Convergence

Absolute Convergence, Conditional 0:02:38

Absolute Convergence, Conditional Convergence, Another Example 2

Absolute and Conditional 0:05:37

Absolute and Conditional Convergence

Absolute & Conditional 0:05:29

Absolute & Conditional Convergence - Infinite Series

Absolute & Conditional 0:24:46

Absolute & Conditional Convergence | Calculus 2 Lesson 28 - JK Math

Testing for Absolute 0:29:49

Testing for Absolute & Conditional Convergence | Easy Explanation! | Math with Professor V

Absolute Convergence, Conditional 0:23:20

Absolute Convergence, Conditional Convergence, and Growth Regressions

Absolute & Conditional 0:08:10

Absolute & Conditional Convergence - Example 2

Absolute Convergence | 0:13:02

Absolute Convergence | Conditionally Convergent | Infinite Series

Calculus 2 - 0:03:15

Calculus 2 - Absolute and Conditional Convergence - Practice Problem

Absolute vs. Conditional 0:11:39

Absolute vs. Conditional Convergence

What Is Conditional 0:01:56

What Is Conditional Convergence?

👊Absolute vs. 🤷🏻‍♀️conditional 0:01:00

👊Absolute vs. 🤷🏻‍♀️conditional convergence 🤔🤔 #apcalculus #apcalc #unit5 #shorts...

Absolute and conditional 0:13:50

Absolute and conditional convergence (KristaKingMath)

Комментарии
Автор

@1Yasi Dividing everything in this equation by n^5 and taking the limit as n--> infinity, you will be left with 0/infinity which = 0 meaning this series converges. The test for divergence fails and you have to use another method to solve this.

MrKayhen
Автор

why no conditional convergent samples? ty for your videos, they are great!

rostyloco
Автор

why did u divide by 1/n^2 in the second part?

mppmahendra
Автор

whoops, I said that the limit = 0 which means it converges, this is not the case. This means you must use other tests to figure out whether or not this series converges or diverges and patrickJMT did the other tests.

MrKayhen
Автор

not Abs.Convergent. Just Convergent, since the +4 is like nothing so you can write it like
(n^3 +n)/n^5 . by simplifying we write, (1/n^2)+(1/n^4) = convergent+convergent = convergent

MyVipr
Автор

Patrick you fucked me up on this question... doesnt your answer (1) only mean that the series is divergent? Check again please

osama