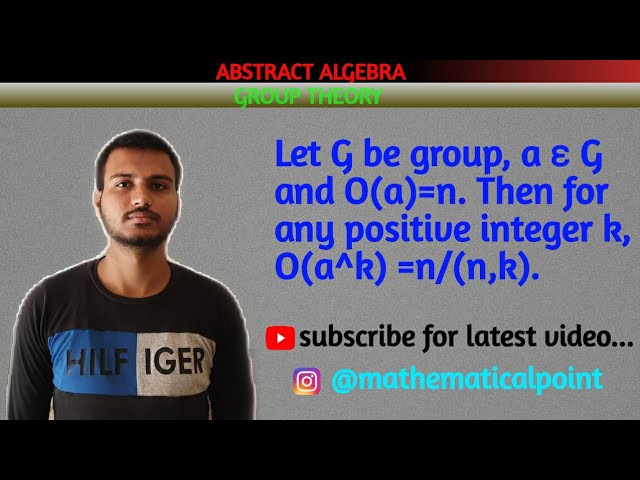
Let G be group, a ɛ G and O(a)=n. Then for any positive integer k, O(a^k) =n/(n,k).

Показать описание

In this lecture we will discuss that Let G be group, a ɛ G and O(a)=n. Then for any positive integer k, O(a^k) =n/(n,k).

******************************************************************************

For definition of order of element of group

*****************************************************************************

For previous lecture

*****************************************************************************

For definition of group

*****************************************************************************

*****************************************************************************

**LIKE**

*SHARE**

**SUBSCRIBE**

#grouptheory #abstractalgebra

--------------------------------------------------------------------------------------------------------------------------------------------------------------

Рекомендации по теме

Комментарии

Very nice bhai 🥰😊😊mast prhaaya 👍🏻thnku so much

navpreetKaur-xxbt

Kya ap jo isse agla topic hai Idempotent element uski theroms bhi krwa skte ho because ap bhut achaa krvate ho..

shivaniSharma-

Hi sir..Pls explained in English language

jothikajo

Really thank u so so so so much sir 😇😇😇😇😁😁😁😁😁😁😁😁😁😁😁😁😁😁😁😁😁

Finished-hc

Jab ye therom paper mai aye to kya humne ye cor. Bhi likhni pdti hai...??

shivaniSharma-

Mzaaa agya bro thnkx so much bs end pr thoda sa doubt bcha h corolli m

Indiandragon

Bhaia (show that the equation x^2 ax=a^-1 is solvable for x in a group G if and only if a is the cube of some element in G.) please is ko explain kijiya. 🙏

Sahil-ykhq

bhai gcd of (N1, k1)=1 let karne sa kya ho jaya ga

chunmunchunmun

Let G be group, a ɛ G and O(a)=n. Then for any positive integer k, O(a^k) =n/(n,k).

Let G be group, a ɛ G and O(a)=n. Then for any positive integer k, O(a^k) =n/(n,k).

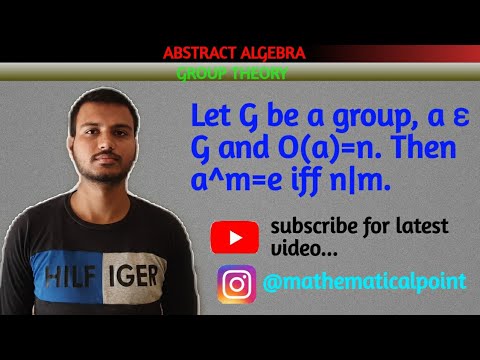
Let G be a group, a ɛ G and O(a)=n. Then a^m=e iff n|m.

Let G be a group ; then o(a)|o(G) for an element a in G.

Proof: Group Element is the Inverse of its Inverse | Abstract Algebra

Let G be a group and Phi an automorphism of G. If a in G has order o(a), then o(phi(a)) is o(a) .

Group Theory | Let G be group of even order prove that at least one element of order 2? | Pure math

let G be a group and a belong to a to G has infinite order, then all distinct power of the a are

if G be finite group and a be any element of G s.t. o(a)=n them a^m=e iff n|m

Corollary Let G be a finite group, and let a belongs to G. Then, a|G|= e.

If G be a Group,then (ab)'=b'a' /Group Theory/3rd sem/unit-1/Telugu expalination

Let G be a group and let Z(G) be the center of G. If G/Z(G) is cyclic, then G is Abelian.

Let G be a subgroup of a group and let a,b ∈ G. Then aH = H iff a∈H (Coset theorem bsc )

Let G be cyclic group of order n then a_k is generator of G if gcd(k, n)=1

Lecture 13 | Let G be a group, Then a^k=e iff k=nq where q is an integer |Group Theory by S.M.Yousaf

Lecture 18 | Let G be a group and x be an element of odd order in G then for any y in G y^2 =x exist

#24 Let G be a group and a be an element of order 30 in G then the order of a^18 is | UPTGT 2004

Abstract Algebra | If G/Z(G) is cyclic then G is abelian.

Let G be cyclic group and a is it's generator. The element a^k is also a generator iff ( k,d)=1

Let (𝐺, .) be a group of all non-zero complex no. Show that H={a+ib:a^2+b^2=1} is a subgroup of 𝐺....

let G be a group & x be an element of odd order in G then for any element y in G their exist y^2...

Proof that f(a) = a^(-1) is a Group Isomorphism if G is Abelian

Let G be a cyclic group order n generated by a...(B.sc Group theory Theorem)

GATE CS 2014 Set 3 | Question: 3 Let G be a group with 15 elements. Let L be a subgroup of G.

Let G be a group of order 24 then G can have a subgroup of order cucet 2018 theory iit jam maths cmi