filmov
tv
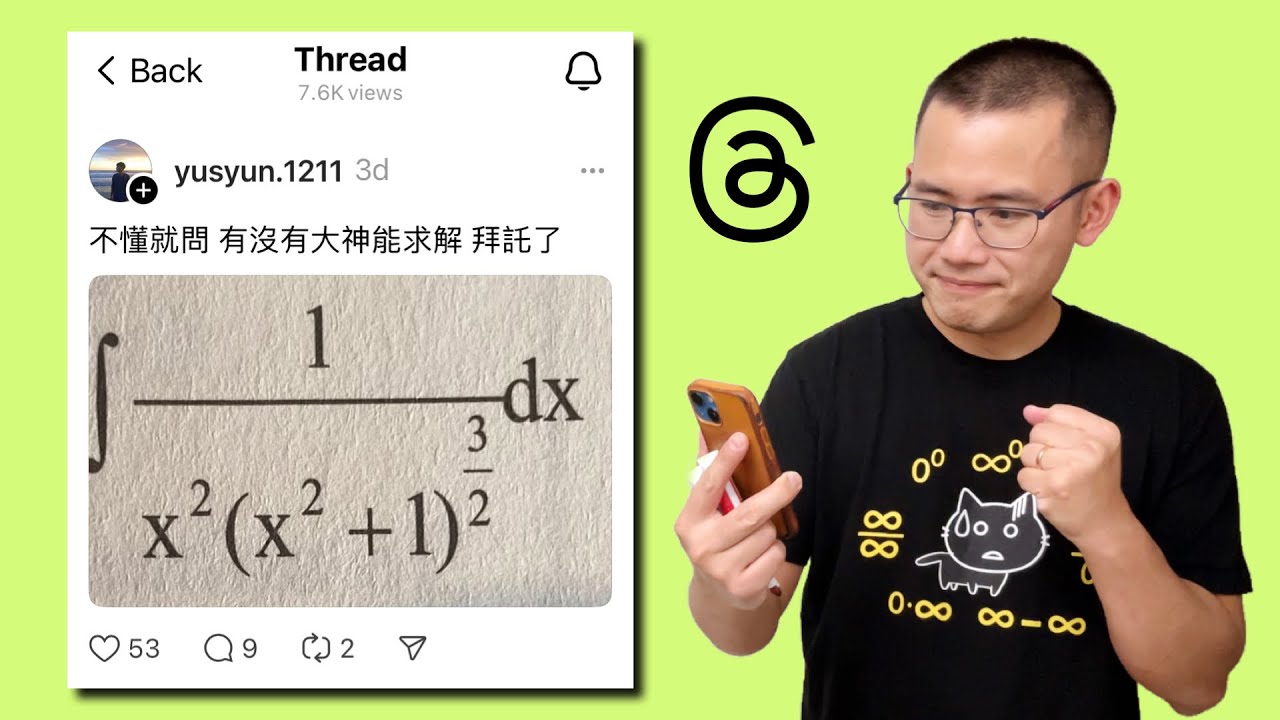
Helping you with the integral of 1/(x^2*(x^2+1)^(3/2)) using trigonometric substitution, Calculus 2
Показать описание
-----------------------------
Support this channel and get my calculus notes on Patreon: 👉
-----------------------------
#calculus #bprpcalculus #apcalculus #tutorial #maths
Helping you with the integral of 1/(x^2*(x^2+1)^(3/2)) using trigonometric substitution, Calculus 2
A wonderful integral!
This Will Help You With Your Calc 2 Integral!
An integral trick whenever you have (f+f’)*e^x (Berkeley Math Tournament Integral Bee)
Help me solve this integral!
Learn to Solve an Integral (What Makes You Beautiful Parody) AP Calculus BC
Integration and the fundamental theorem of calculus | Chapter 8, Essence of calculus
Help me solve this integral #calculus #maths
Integral of the Day 11.11.24 | Trig Sub & Then...Can You Solve It? | Math with Professor V
Find The Integral – How To Do Basic Calculus Integration
Integral of 1/(1 - 2x) using u-substitution #shorts
Introduction to integral calculus | Accumulation and Riemann sums | AP Calculus AB | Khan Academy
Integral of ln(x) with 'infinite' integration by parts
Integral of 1/(1+e^x)
Integration Tricks (That Teachers Won't Tell You) for Integral Calculus
Integral of the Day 1.17.24 | Integration Help! | Math with Professor V
Tutorials & Lectures for Calculus 1, 2, and 3! #mathwithprofessorv #calculusvideos #integrals
You'll love this embedded integral
Solving the Gaussian Integral the cool way
Only FEYNMAN'S TRICKS can help solve this TERRIFYING INTEGRAL
Actually, this double integral will be easier to evaluate in polar coordinates!
Learn to take the integral of with u sub trig and ln
Calculus Tutorials & More! #mathwithprofessorv #calculusvideos #chainrule #integrals
You need to try this integral
Комментарии
 0:07:21
0:07:21
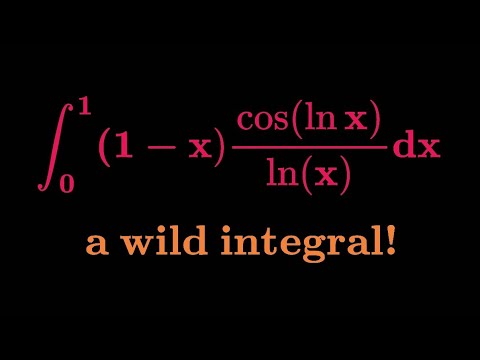 0:10:51
0:10:51
 0:00:44
0:00:44
 0:05:37
0:05:37
 0:02:03
0:02:03
 0:03:39
0:03:39
 0:20:46
0:20:46
 0:00:35
0:00:35
 0:12:36
0:12:36
 0:11:08
0:11:08
 0:00:44
0:00:44
 0:04:52
0:04:52
 0:00:59
0:00:59
 0:00:58
0:00:58
 0:11:26
0:11:26
 0:04:52
0:04:52
 0:00:54
0:00:54
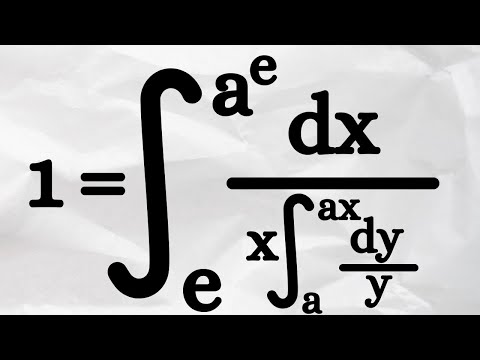 0:05:47
0:05:47
 0:09:39
0:09:39
 0:14:17
0:14:17
 0:05:03
0:05:03
 0:00:59
0:00:59
 0:00:31
0:00:31
 0:16:51
0:16:51