filmov
tv
Henry Adams (3/3/15): The Vietoris Rips Complex of the Circle
Показать описание
Given a metric space X and a distance threshold r, the Vietoris-Rips simplicial complex has as its simplices the finite subsets of X of diameter less than r. A theorem of Jean-Claude Hausmann states that if X is a Riemannian manifold and r is sufficiently small, then the Vietoris-Rips complex is homotopy equivalent to the original manifold. Little is known about the behavior of Vietoris-Rips complexes for larger values of r, even though these complexes arise naturally in applications using persistent homology. We show that as r increases, the Vietoris-Rips complex of the circle obtains the homotopy types of the circle, the 3-sphere, the 5-sphere, the 7-sphere, ..., until finally it is contractible. Joint work with Michal Adamaszek.
Henry Adams (3/3/15): The Vietoris Rips Complex of the Circle
Henry Adams (3/12/21): Vietoris-Rips thickenings: Problems for birds and frogs
BilTop | Henry Adams | An introduction to Vietoris-Rips complexes
Henry Adams - Bridging applied and geometric topology
Henry Adams (8/30/21): Vietoris-Rips complexes of hypercube graphs
Henry Adams (3/22/22): Gromov-Hausdorff distances, Borsuk-Ulam theorems, and Vietoris-Rips complexes
Henry Adams (5/1/21): Bridging applied and quantitative topology
Vietoris Rips complex and Persistence Barcodes
Henry Adams: Bounding Gromov-Hausdorff distances with Borsuk-Ulam theorems and Rips complexes
What is the difference between Vietoris-Rips and Cech complexes?
DTM-based filtrations: Rips filtration on a noisy dataset
Henry Adams (10/11/17): Metric reconstruction via optimal transport
Applied topology 23: Paper Introduction: Coordinate-free coverage in sensor networks
Vietoris-Rips complex
Algebraic Stability of Persistence Diagrams [Ziva Urbancic]
Michael Lesnick (5/3/21): l_p-Metrics on Multiparameter Persistence Modules
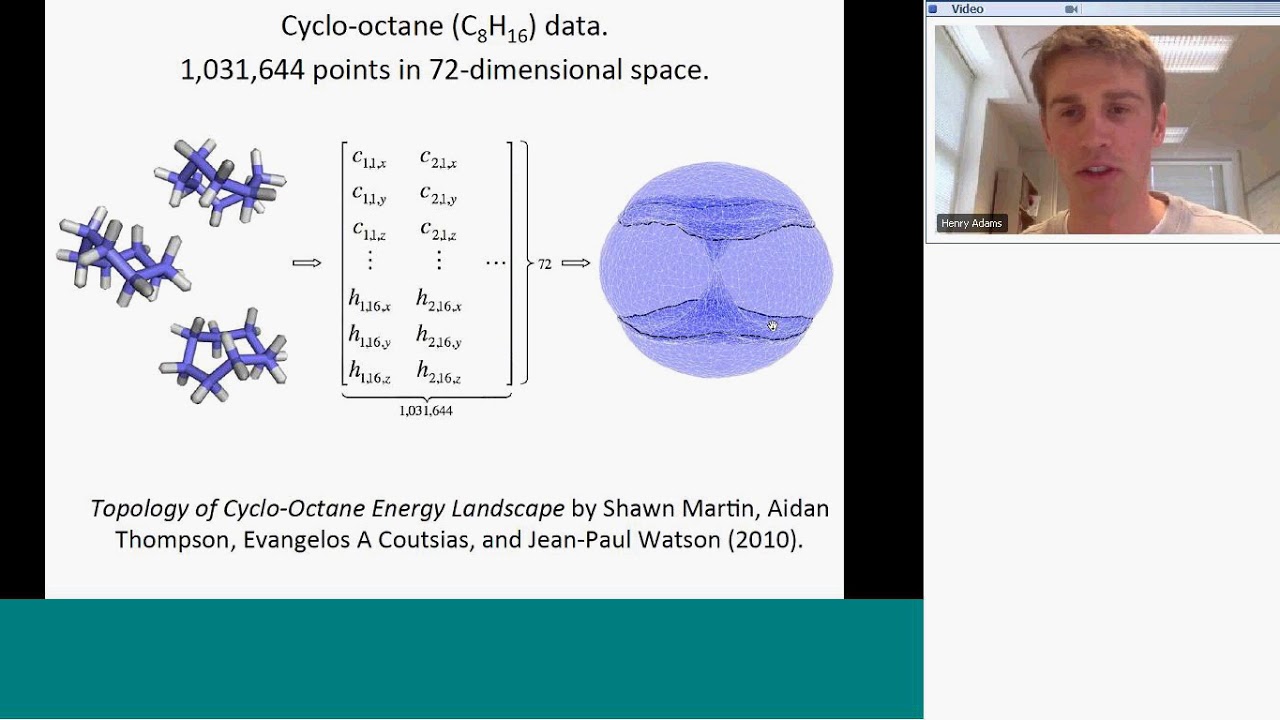
Henry Adams (6/4/20): Descriptors of Energy Landscapes using Topological Analysis (DELTA)
Let’s talk about random Cech and Vietoris-Rips complexes [Andrew M. Thomas]
Applied topology 16: Sublevelset persistent homology
Meet the Team: Christian Vietoris
Intuitive Persistence - Damiano - 2020
Chad Giusti (4/13/22): An approach to assigning semantics to persistent homology classes
Johnathan Bush (11/5/21): Maps of Čech and Vietoris–Rips complexes into euclidean spaces
Computing Persistence diagrams using Linear Algebra [Donald Woukeng Feudjio]
Комментарии
 0:56:36
0:56:36
 1:17:41
1:17:41
 1:02:54
1:02:54
 0:22:07
0:22:07
 0:44:56
0:44:56
 0:57:04
0:57:04
 0:58:22
0:58:22
 0:00:10
0:00:10
 0:56:21
0:56:21
 0:10:52
0:10:52
 0:00:20
0:00:20
 0:48:47
0:48:47
 0:10:35
0:10:35
 0:08:50
0:08:50
 0:10:22
0:10:22
 1:13:54
1:13:54
 0:49:08
0:49:08
 0:10:20
0:10:20
 0:07:45
0:07:45
 0:01:01
0:01:01
 0:08:40
0:08:40
 0:50:16
0:50:16
 0:44:43
0:44:43
 0:11:45
0:11:45