Henry Adams (5/1/21): Bridging applied and quantitative topology

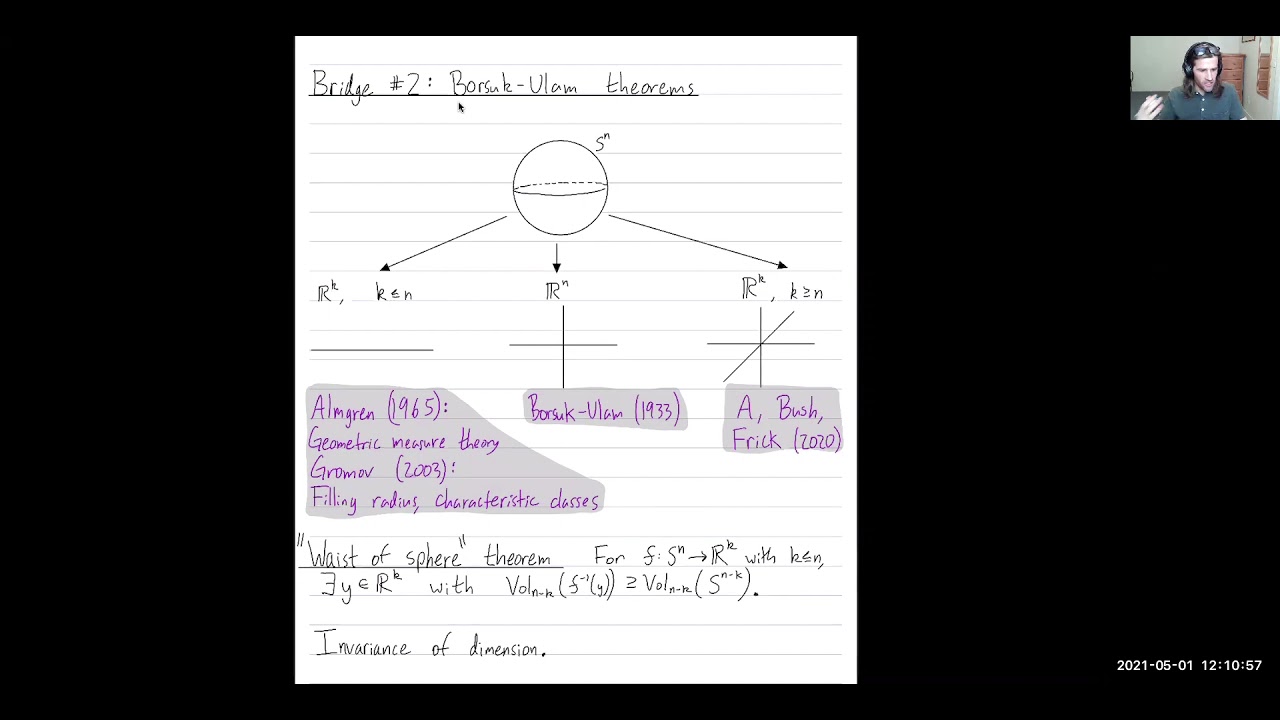
I will survey emerging connections between applied topology and quantitative topology. Vietoris-Rips complexes were invented by Vietoris in order to define a (co)homology theory for metric spaces, and by Rips for use in geometric group theory. More recently, they have found applications in computational topology for approximating the shape of a dataset. I will describe a recent result by my student Michael Moy showing that Vietoris-Rips simplicial complexes and Vietoris-Rips metric thickenings (defined using optimal transport) have the same persistence diagrams, and based upon this work I will speculate on the homotopy types of Vietoris-Rips complexes of n-spheres.