The Jordan Form of a 3 by 3 matrix: Example 1

Показать описание

We give an example of finding the Jordan form of a 3x3 matrix. The algebraic multiplicity of the only eigenvalue is 3 and the geometric multiplicity is 1. The Jordan form has two ones above the main diagonal.

#mikedabkowski, #mikethemathematician, #profdabkowski

Рекомендации по теме

Комментарии

Finally. Your method is the easiest I saw. Most examples just use a huge system of equations to try and find each term of the matrix in Jordan form, but yours is way more simple! Thanks!

TheJoaovicente

Sorry professor I don’t quite get in 9:59. How can you immediately get the Jordan matrix {{9, 1, 0}, {0, 9, 1}, {0, 0, 9}}? I know that the diagonals should be 9 because 9 is the eigenvalue, but how can you determine the 1s and 0s in the other entries of the matrix? Can you teach me please? Thank you🙏🙏🙏

sultanhsu

Thank you prof Mike, your analysis and linear algebra lectures are wonderful

tianshugu

Hi, many thanks, you made something click for me! Say, is it standard to teach the entire proof of computing a Jordan form? It's very pretty and interesting to grasp, but given the practical uses, it seems so redundant.

hhhhheeeelllllllllooooo

you sound alot like MatPat! thank you for the video.

yassinenajar

The Jordan Form of a 3 by 3 matrix: Example 1

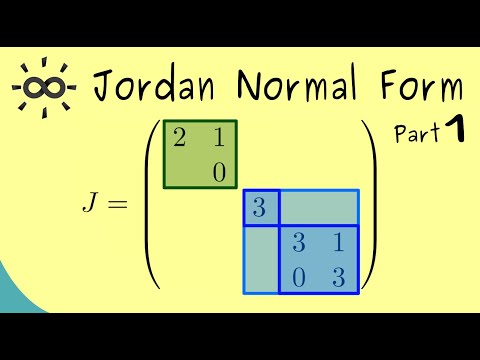
Jordan Normal Form - Part 1 - Overview

The Jordan Form of a 3 by 3 matrix: Example 1

Jordan Form

Systems of Differential Equations: Diagonalization and Jordan Canonical Form

Introduction to Jordan Canonical Form

Jordan Canonical Form

What is...the Jordan normal form?

Linear Algebra 22: Convert a 3x3 matrix into Jordan form (Ch10 Pr2c)

Determining the Jordan form of a matrix

Jordan canonical forms and invariant theory

Overview of Jordan Canonical Form

Example of Jordan Canonical Form: 2x2 Matrix

Advanced Linear Algebra, Lecture 4.7: Jordan canonical form

Determining the Jordan canonical form - example 1

LAII 009 Example of a Jordan normal form

The Jordan Form of a 3 by 3 matrix: Example 2

Jordan Canonical Form of a 3x3 Matrix

Jordan canonical form

LAII 007 Example of a Jordan normal form

Some examples on the Jordan form of a given matrix and generalised eigenvectors

Advanced Linear Algebra 25: Jordan Decomposition Generalized Eigenspace

Gauss Jordan Elimination & Reduced Row Echelon Form

Example of Jordan Canonical Form: Real 4x4 Matrix with Basis 1

Comparison of the Diagonal and the Jordan Canonical Forms, 14/3/2016