filmov
tv
LAII 009 Example of a Jordan normal form
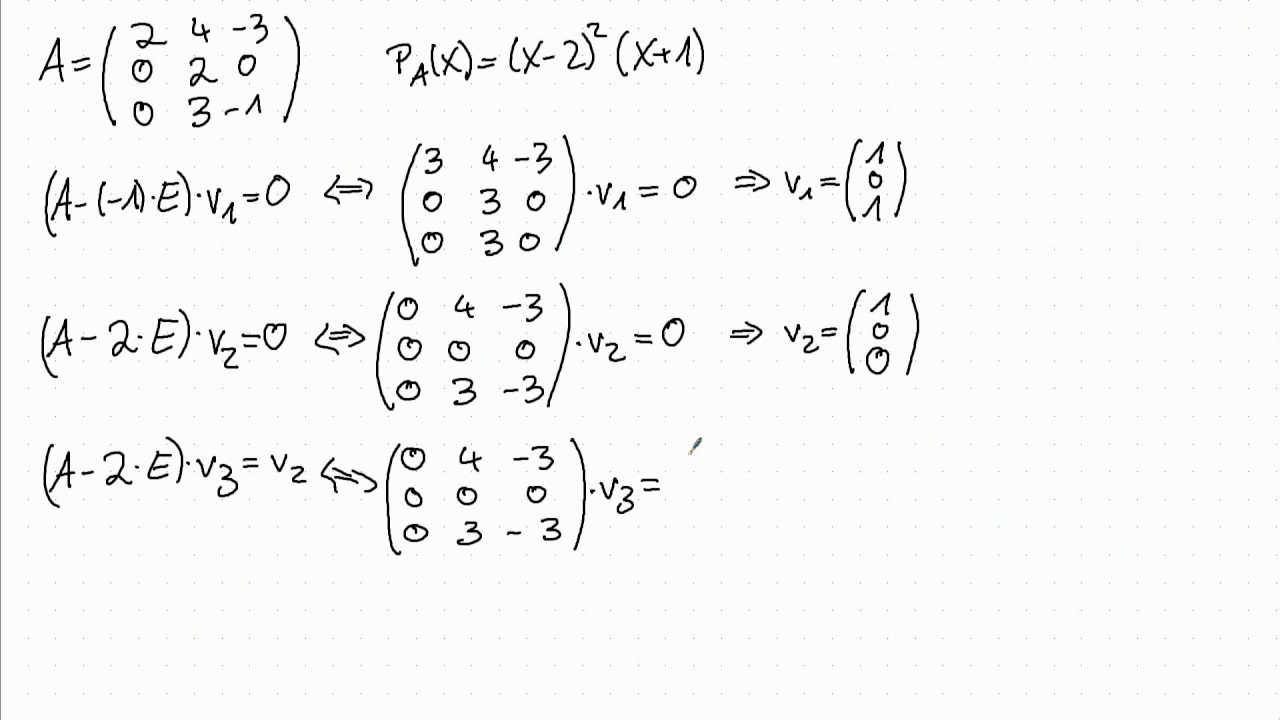
Показать описание
We will compute the Jordan normal form and the base change matrix for a matrix with two eigenvalues and two Jordan blocks.
LAII 009 Example of a Jordan normal form
Lung inflation in Science Lesson #science #teacher #biology
What’s mean is hi qi lai in chinese
Даже Ваше Имя Тайно Зашифровано в Библии - Ведущий криптограф совершает революционное открытие...
How to read Vernier Caliper in Urdu/Hindi | Easy Method
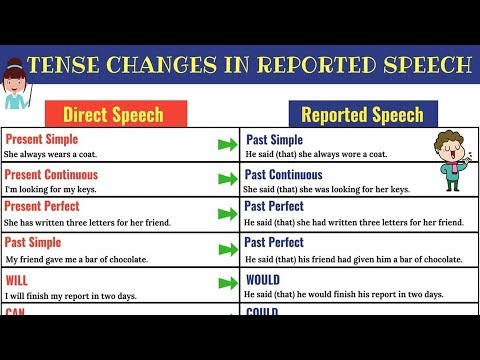
REPORTED SPEECH: Verb Tense Changes | Direct and Indirect Speech in English
LAII 007 Example of a Jordan normal form
A few numbers and letters to draw two birds #simplestrokes #draw #simpledrawing #digitalpainting
How to find out Percentage from Calculator Easy Way in Different Situations | Part-1
Letter To Your Friend || Formal/Informal Letter Writing In English || Beautiful Handwriting
English Pronunciation | The Letter 'A' | 9 Ways to Pronounce the Letter A
Count from 1 to 10 in Arabic
How many mililiter(ml) in 10 milligram(mg).
constructing an angle of 30° degree || How to construct 30° degrees
Understanding Ohm’s Law: Exploring Voltage, Current, and Resistance
🚂 The Treasure-Train 💎 | Classic Detective Mystery by Arthur B. Reeve 🕵️♂️
3rd Grade Division Strategies
Cash Receipts Journal (CRJ) | Explained with Examples
Molly Wright: How Every Child Can Thrive by Five | TED
Measles Signs and Symptoms
cambridge writing task 2 band 9 sample Essay - do you agree or disagree
Punnett Square Basics | Mendelian Genetic Crosses
How to draw the Tiger by AMANDA LAI Part 8 of 9
2018 Spyderco Amsterdam Meet - Brian Lai Amalgam Prototype Sample
Комментарии
 0:06:33
0:06:33
 0:00:16
0:00:16
 0:00:17
0:00:17
 0:29:04
0:29:04
 0:11:09
0:11:09
 0:05:02
0:05:02
 0:05:35
0:05:35
 0:00:40
0:00:40
 0:00:35
0:00:35
 0:04:13
0:04:13
 0:17:37
0:17:37
 0:00:28
0:00:28
 0:02:37
0:02:37
 0:00:35
0:00:35
 0:00:57
0:00:57
 8:42:54
8:42:54
 0:03:18
0:03:18
 0:18:51
0:18:51
 0:07:43
0:07:43
 0:00:44
0:00:44
 0:07:27
0:07:27
 0:02:52
0:02:52
 0:01:45
0:01:45
 0:01:31
0:01:31