filmov
tv
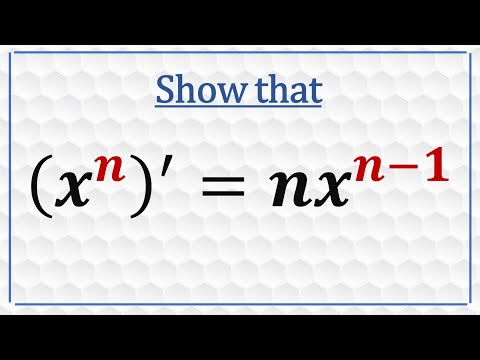
Proof of the Power Rule

Показать описание
Download the workbook and see how easy learning calculus can be.
Proof of the Power Rule
A 'proof' of the power rule
(Proof) The power rule of derivatives (for natural exponents) | Quick Math
Proof of the Power Rule (for positive integers)
Proof of the Power Rule
(Single-Variable Calculus 1) Proof of Power Rule 1
Power Rule: Visual Proof in Calculus
The Power Rule - Intuitive Proof
AS Pure Mathematics - 14B Exponential Functions and differentiating Exponential functions
Power rule | Derivative rules | AP Calculus AB | Khan Academy
Justifying the power rule | Derivative rules | AP Calculus AB | Khan Academy
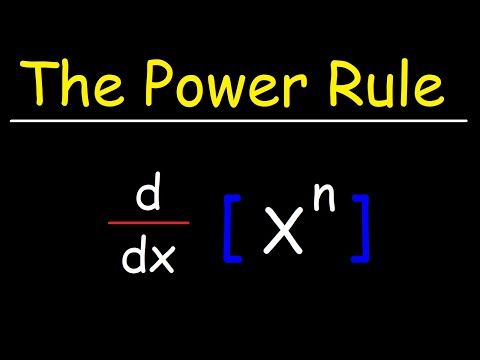
The Power Rule For Derivatives
Proof of the 'Power Rule' for derivatives using Induction
Proving the Power Rule (for Natural Numbers) with the Binomial Expansion | Differentiation
the power of derivative power rule
Proof of the Power Rule and Using the Derivative
Proof of the power rule
Proof of Power Rule Explained | Algebra Tutorial
PROOF of Power Rule of Derivatives | Calculus MCV4U | jensenmath.ca
What is a Derivative? Deriving the Power Rule
Power Rule for Differentiation (3 of 4: Derivation of rule)
3.7 Proof of the power rule*
Power Rule proof 🔥 | watch video 🔥 | only 2 Minutes 🔥
Proof of the Power Rule and Other Derivative Rules
Комментарии
 0:06:10
0:06:10
 0:03:41
0:03:41
 0:03:23
0:03:23
 0:04:36
0:04:36
 0:10:28
0:10:28
 0:15:08
0:15:08
 0:00:43
0:00:43
 0:06:47
0:06:47
 0:24:21
0:24:21
 0:03:54
0:03:54
 0:06:42
0:06:42
 0:12:01
0:12:01
 0:01:42
0:01:42
 0:08:50
0:08:50
 0:00:31
0:00:31
 0:14:13
0:14:13
 0:12:41
0:12:41
 0:00:37
0:00:37
 0:11:37
0:11:37
 0:10:05
0:10:05
 0:10:26
0:10:26
 0:03:21
0:03:21
 0:00:15
0:00:15
 0:09:39
0:09:39