Find Pivots, Pivot Rows, and Pivot Columns with Row Echelon Form | Linear Algebra

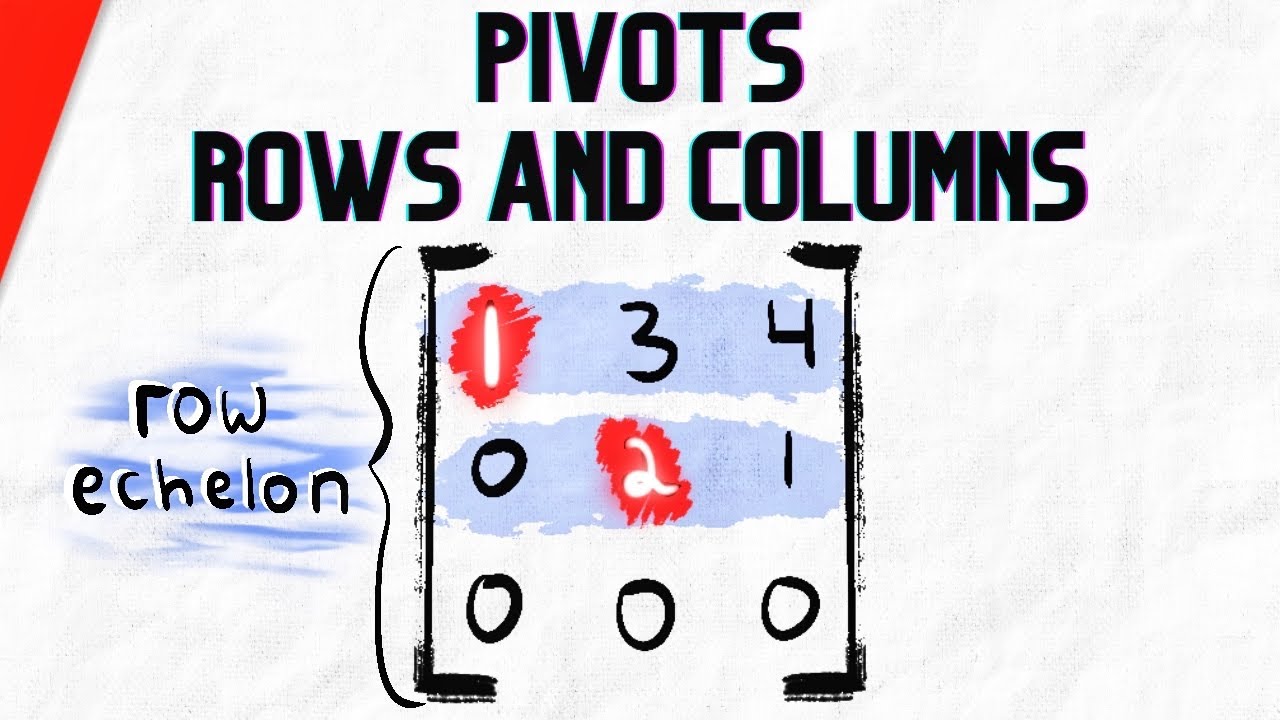
We go over how to find the pivot positions, pivots, pivot rows, and pivot columns of a matrix by considering its row echelon or reduced row echelon forms. #linearalgebra

Join Wrath of Math to get exclusive videos, lecture notes, and more:

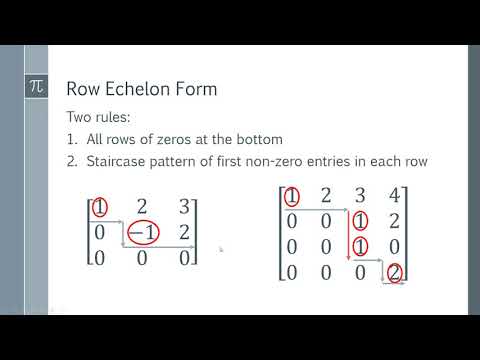
The pivot positions of a matrix are the positions of the leading 1s when it is written in row echelon or reduced row echelon form. The entries of the matrix that are in these position are the pivots of the matrix. The rows containing the pivot positions are the pivot rows and the columns containing the pivot positions are the pivot columns.

★DONATE★

Follow Wrath of Math on...

[WrathofMath]

Support this course by joining Wrath of Math to access exclusive and early linear algebra videos, plus lecture notes at the premium tier!

[AshishPathak]

wonderful explanation. great videos.... please have a series on ordinary differential equations and numerical analysis

[PunmasterSTP]

This video, this video? It's pivotal!

[darkestlight]

thanks a lot for this. I was looking for 1 piece of info and you really helped.

[TahaQuddus]

I shocked in pivotation
Thanks that was good

[wryltxw]

can you consider doing a series on probability exercises.

[MuheebKhan-zu]

Is there any difference between pivot and leading entry؟ please explain

[hudsonvanBeek]

Hey I’m taking linear algebra in university and these videos are amazing for when I’m reviewing content from the classes… would also like it if you could go over a bit more things in future videos to help assist my understanding videos about (span, sub space, linear independence, basis and dimensions, sensor problems, proofs, orthogonal and best fit, matrix multiplication, determinant and inverse, characteristic polynomial, and PCA)

[SyedaAniqaliaquat]

making videos on linear algebra and its application 4th edition kindly chpter 1 matrices

[SyedaAniqaliaquat]

kindly provide pdf on matrices chp please